NIOS Class 12 Economics Chapter 10 Correlation Analysis, Solutions to each chapter is provided in the list so that you can easily browse through different chapters NIOS Class 12 Economics Chapter 10 Correlation Analysis and select need one. NIOS Class 12 Economics Chapter 10 Correlation Analysis Question Answers Download PDF. NIOS Study Material of Class 12 Economics Notes Paper 318.
NIOS Class 12 Economics Chapter 10 Correlation Analysis
Also, you can read the NIOS book online in these sections Solutions by Expert Teachers as per National Institute of Open Schooling (NIOS) Book guidelines. These solutions are part of NIOS All Subject Solutions. Here we have given NIOS Class 12 Economics Chapter 10 Correlation Analysis, NIOS Senior Secondary Course Economics Solutions for All Chapters, You can practice these here.
Correlation Analysis
Chapter: 10
Module – IV Statistical Tools
TEXT BOOK QUESTIONS WITH ANSWERS
INTEXT QUESTIONS 10.1
Q.1. It has been noted that there is a positive correlation between the I.Q. level and the size of women’s shoes. With smaller size of shoes of women corresponds to lower intelligence level and higher size of shoes of women corresponds to higher intelligence level of women. Comment on the conclusion that economic factors case hemlines to rise the fall.
Ans. IQ level and the size of women’s shoes may have positive relation, yet the economic factors will have positive relation with the women’s IQ level. Better economy and better facilities and infrastructure means better IQ. And per facilities and infrastructure means lower IQ.
Q.2. A researcher has a large number of data pairs. (age, height) of humans being from birth to 70 years. He computes a correlation coefficient. Would you expect it to be positive or negative? Why?
Ans. Correlation of age and height will be positive. This is because when the age increase the height also increases and it may happen until the age of 25. After that the height will not increase and remain the same with the increase in age. So it will positive correlation.
INTEXT QUESTIONS 10.2.
Q.1. What sort of correlation would be expected between a company’s expenditure on health and safety and the number of work related accidents.
(a) Positive.
(b) Negative.
(c) None.
(d) Infinite.
Ans. (b) negative.
Q.2. When “r” is negative, one variable increase in value,……..
(a) The other increases.
(b) The other increases at a greater rate.
(c) The other variable decreases in value.
(d) There is no change in the other variable.
(e) All of the above.
Ans. (c) The other variable decrease in value.
INTEXT QUESTIONS 10.3.
Q.1. The coefficient of correlation ranges between:
(a) 0 and 1
(b) -1 and +1
(c) Minus infinite and plus infinity.
(d) 1 and 100.
Ans. (b) -1 and +1.
Q.2. If two variables are absolutely, independent of each other the correlation between them must be:
(a) -1
(b) 0
(c) +1
(d) +0.1
Ans. (b) 0
Q.3. The coefficient of correlation:
(a) Can be larger than 1.
(b) Cannot be larger than 1.
(c) Cannot be negative.
Ans. (b) Cannot be larger than 1.
Q.4. If height is independent of average yearly income, what is the predicted correlation between these two variables?
(a) 1
(b) -1
(c) 0
(d) Impossible to say for sure.
Ans. (c) 0.
Q.5. A student produces a correlation of +1.3. This is:
(a) A high positive correlation.
(b) A significant correlation.
(c) An impossible correlation.
(d) Only possible of N is large.
Ans. (c) An impossible correlation.
Q.6. If a scored the top mark in the apprentices test on computing and the correlation between that test and the test on English language was +1.0 what position did A get in the test on English language?
(a) Middle.
(b) Bottom.
(c) Top.
(d) Cannot say from the information given.
Ans. (c) Top.
Q.7. Which correlation is the strongest +0.65 or -0.70?
(a) -0.70.
(b) +0.65
(c) Depends on N.
(d) Cannot say from the information given.
Ans. (a) -0.70.
Q.8. The symbol for the Karl Pearson Correlation Coefficient is:
(α) Σ
(b) σ
(c) a
(d) r
Ans. (d) r.
Q.9. For a normal good, if price increases then the quantity demanded decreases. What type of correlation coefficient would you expect in this situation?
(a) 0
(b) positive.
(c) 0.9
(d) Negative.
(e) Unknowable.
Ans. (d) Negative.
INTEXT QUESTIONS 10.4.
Q.1. Given a set of paired data (X, Y)
(a) If X is independent of X, then what value of a correlation coefficient would you expect?
Ans: The value of a correlation coefficient would be zero.
(b) If Y is linearly dependent on X, then what value of a correlation coefficient would you expect?
Ans. The value of a correlation coefficient would be either +1, -1 or between +1 and -1.
Q.2. State whether the following statement is true or false: “If opposite correlation exists between height and weight, a person with above average height is expected to have above average weight”.
Ans. It is true if a positive correlation exists between height and weight, a person with above average height is expected to have above average weight. This is so because in positive correlation two variable change in the same directions and in the same proportion.
INTEXT QUESTIONS 10.5.
Q.1. Positive values of covariance indicate
(a) A positive variance of the X values.
(b) A positive variance of the Y values.
(c) The standard deviation is positive.
(d) Positive relation between two variables.
Ans. (d) Positive relation between two variables.
INTEXT QUESTIONS 10.6.
Q. 1. The marks awarded by two judges in a certain beauty contest are given below:
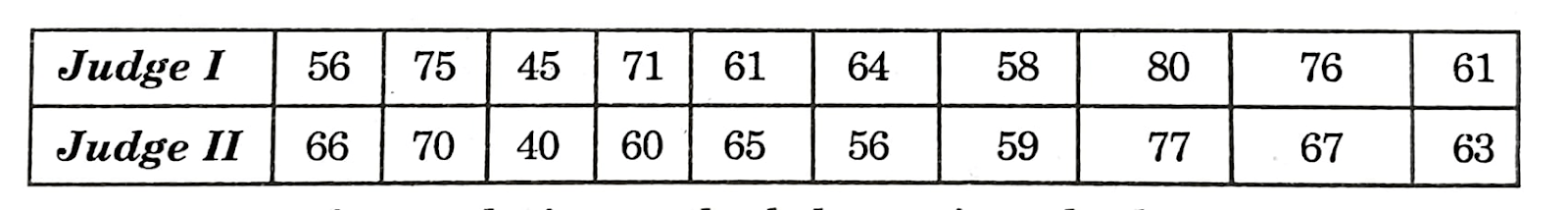
By using rank correlation method, determine whether the two judges have common taste in the judgement of beauty?
Ans. Rank = 1 – 6ΣD² / N(N² – 1)
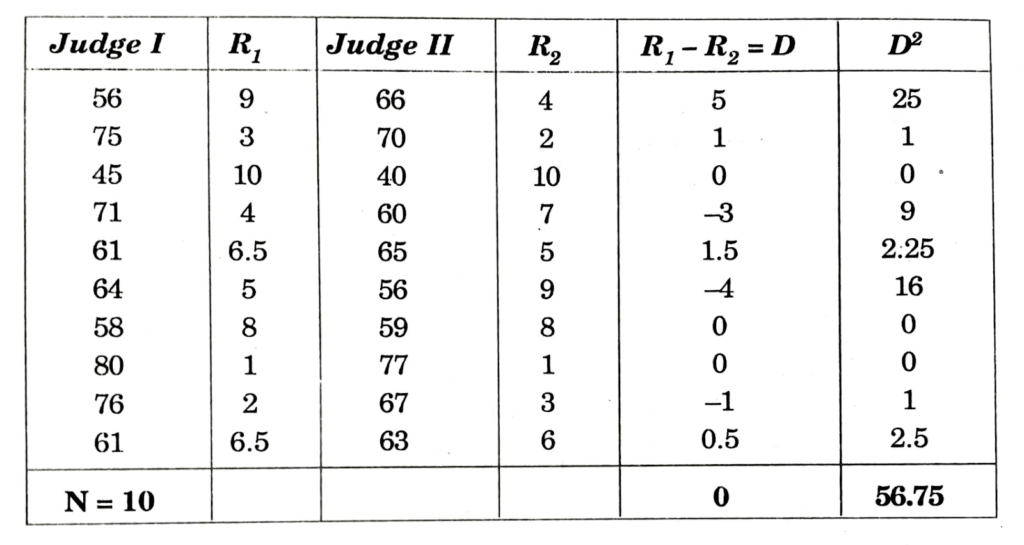
R = 1 – 6ΣD²/N(N² – 1)
= 1 – 6 x 56.75 / 10 (10² – 1) = 1 – 340.5/990
= 1 – 0.34 = 0.66
The two judges have positive correlation.
TERMINAL EXERCISE
Q.1. The data relating to variable X and Y is given below:
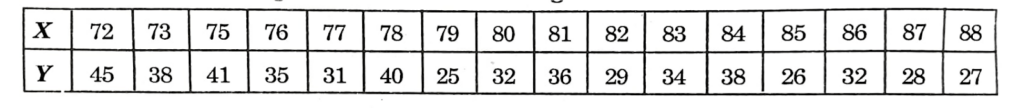
(a) Sketch a scatter plot.
(b) Compute the correlation coefficient, r.
Ans. (a)
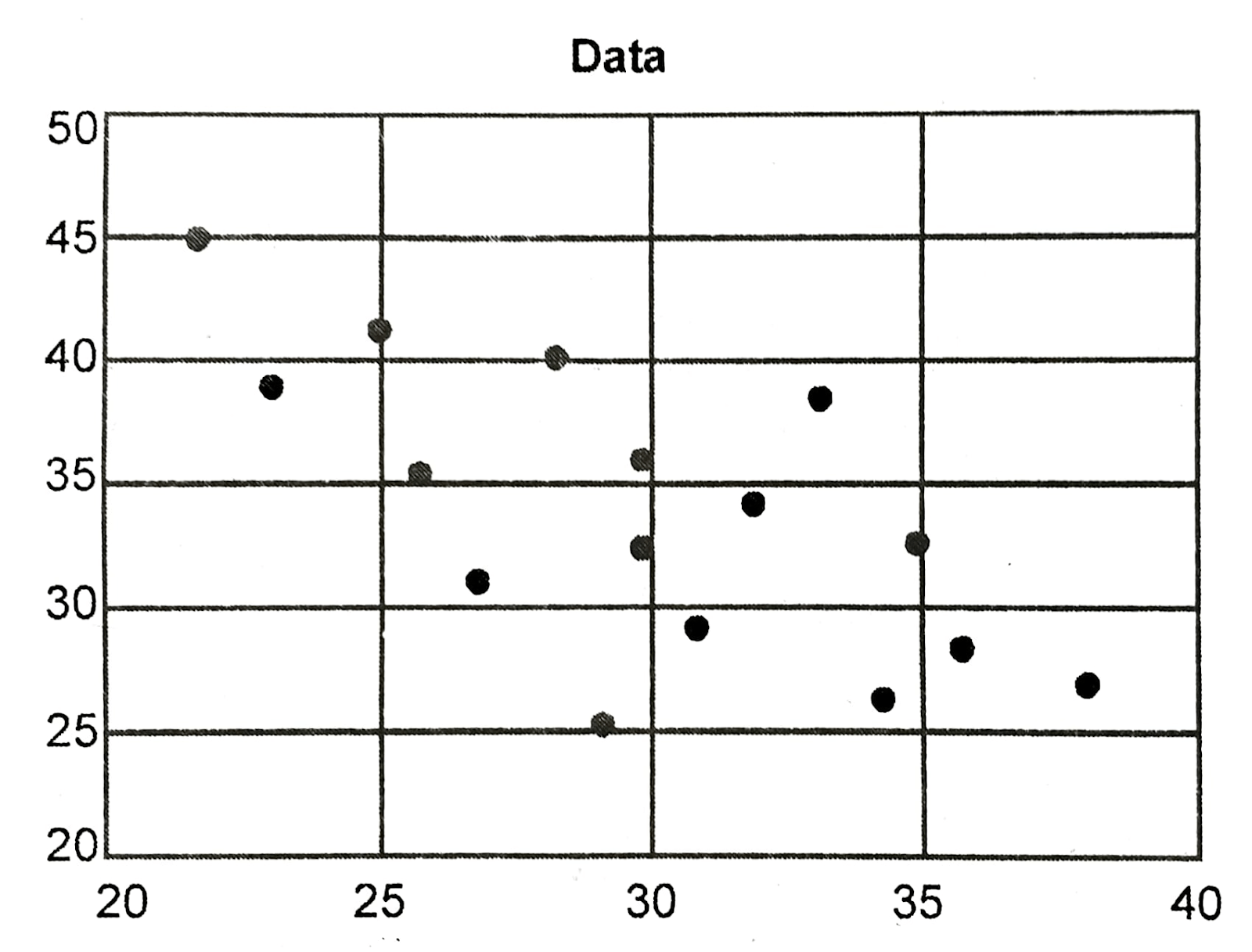
(b)
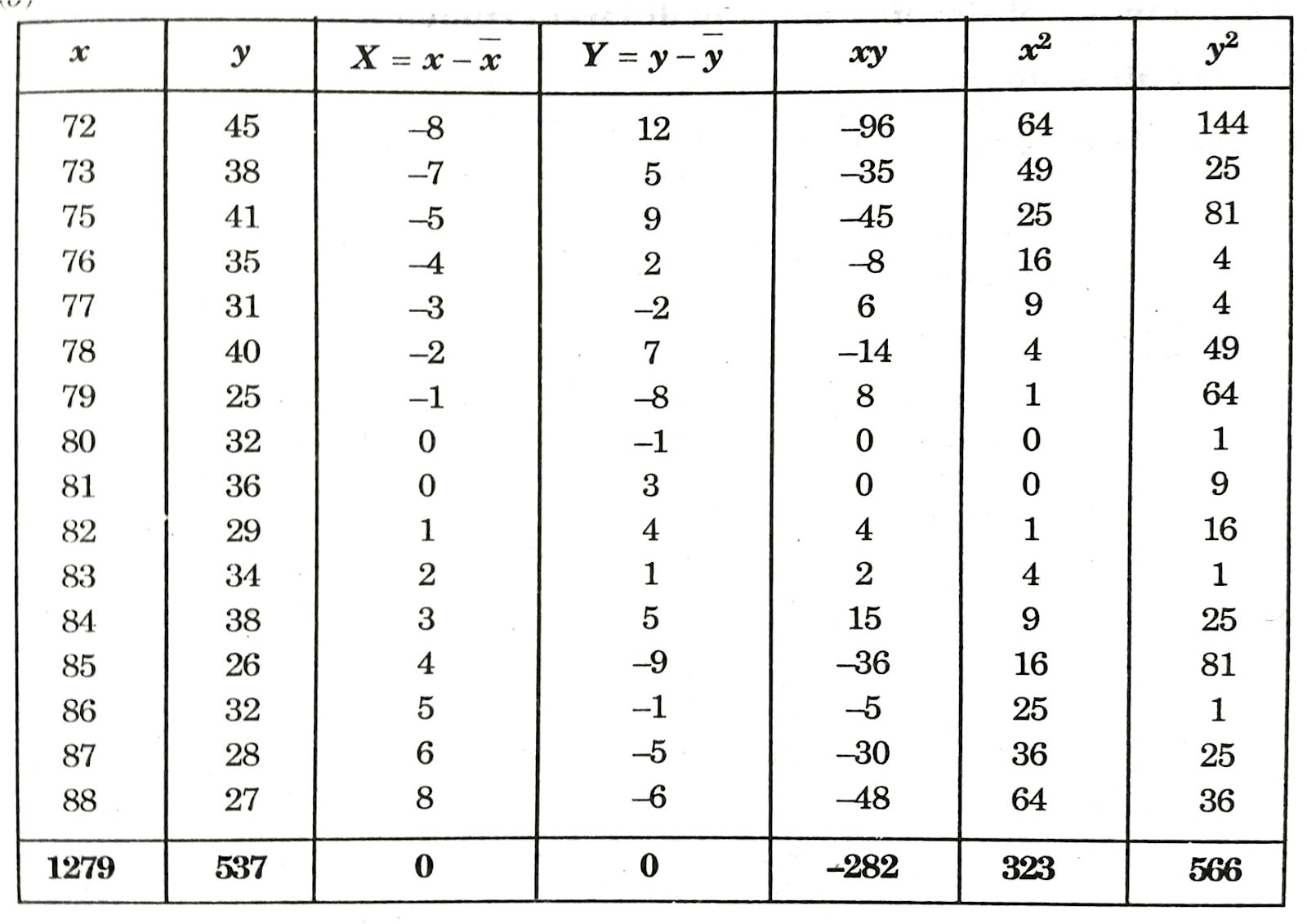
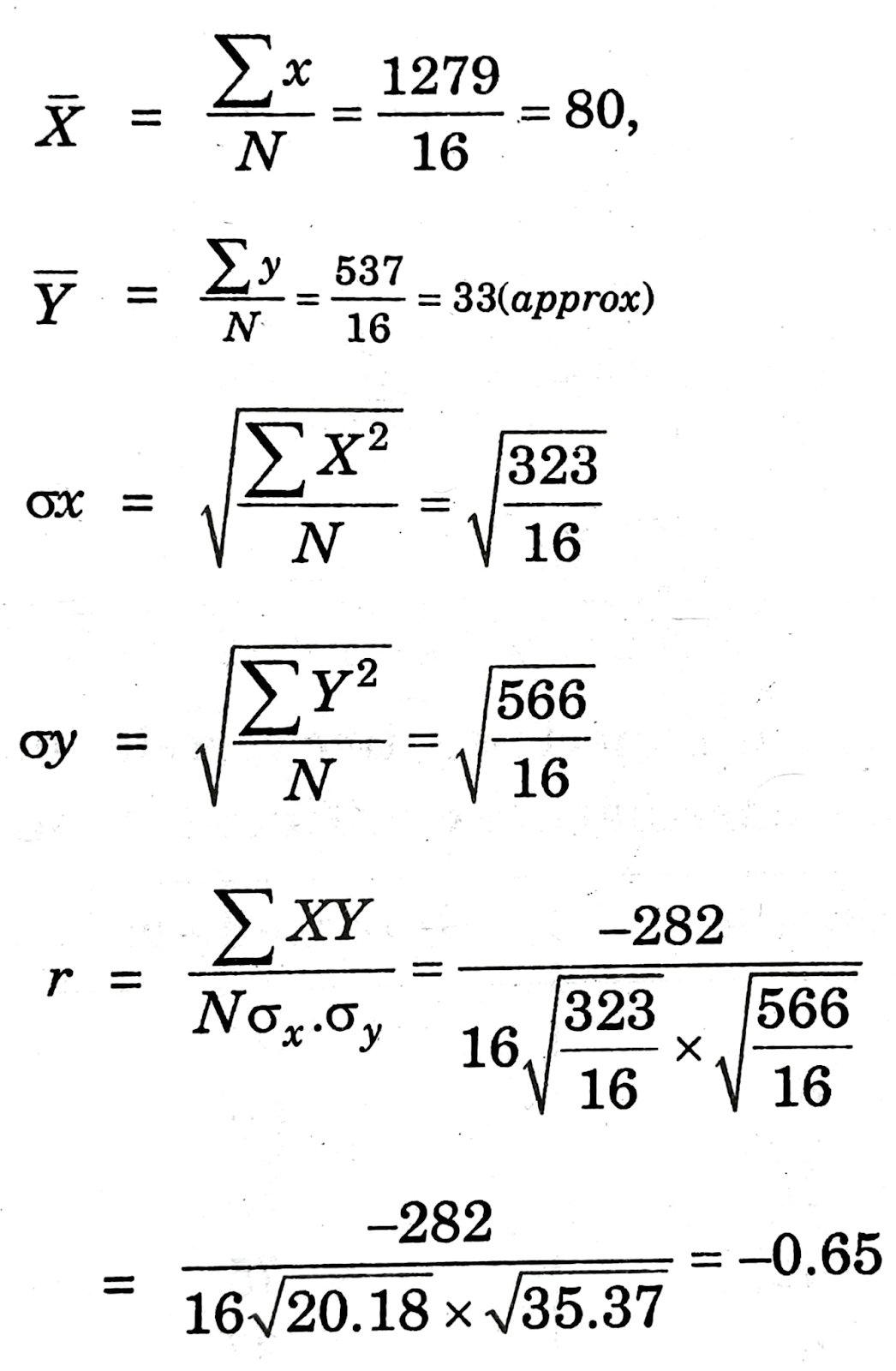
It is a negative correlation.
Q.2. Calculate and analyze the correlation coefficient between the number of study hours and the number of sleeping hours of different students.
| Number of Study hours | 2 | 4 | 6 | 8 | 10 |
| Number of sleeping hours | 10 | 9 | 8 | 7 | 6 |
Ans.
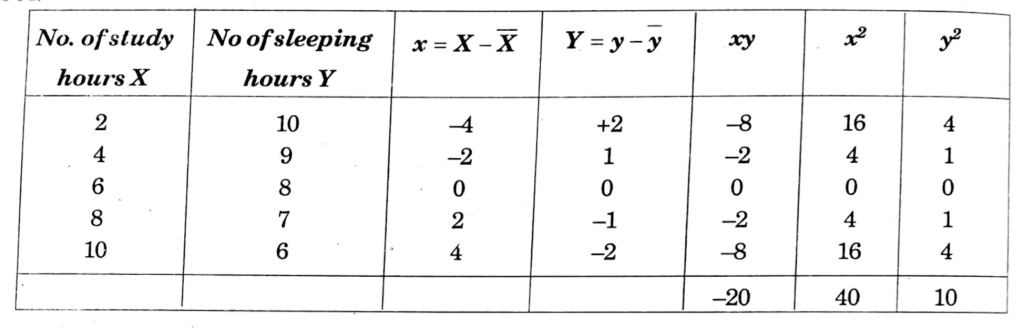
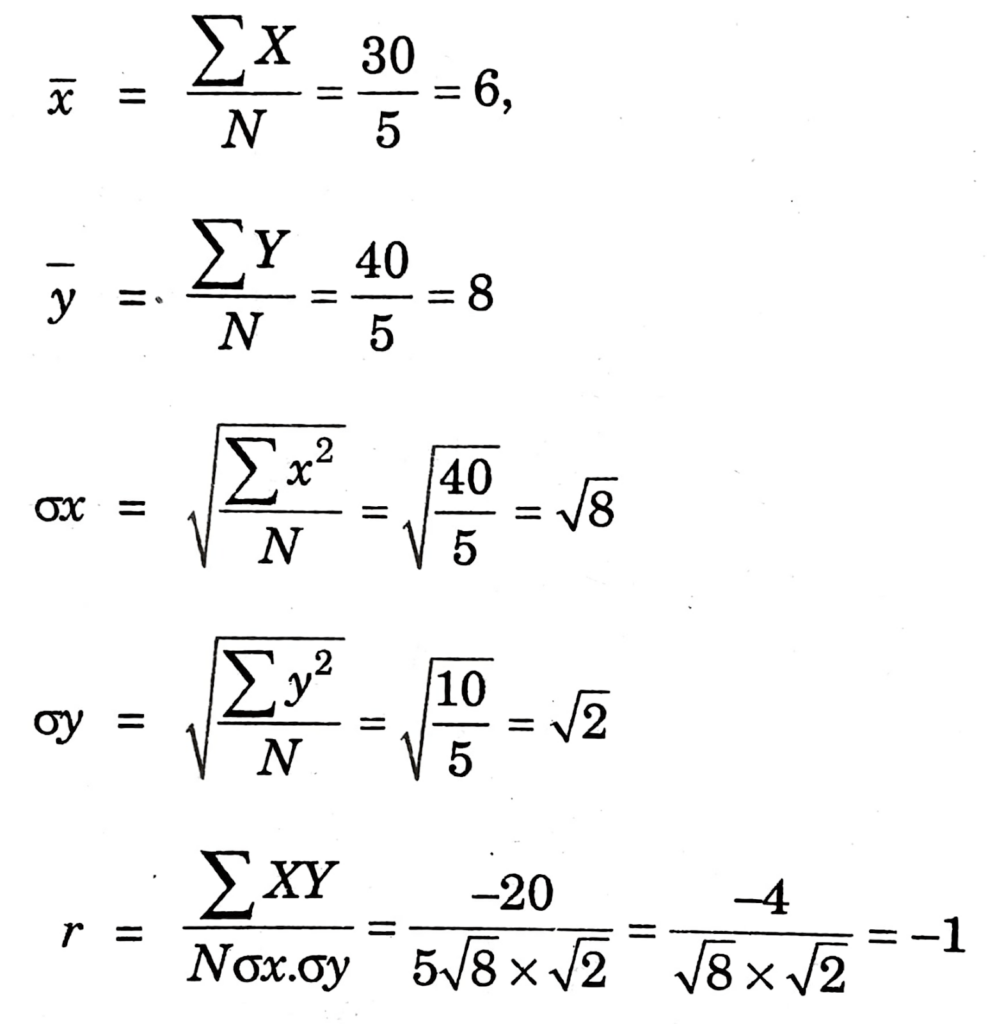
Study hrs and sleeping hrs have perfect negative correlation.
Q.3. Find the value of the correlation coefficient from the following table:
| Subject | Age X | Glucose Level Y |
| 1 | 43 | 99 |
| 2 | 21 | 65 |
| 3 | 25 | 79 |
| 4 | 42 | 75 |
| 5 | 57 | 87 |
| 6 | 59 | 81 |
Ans.
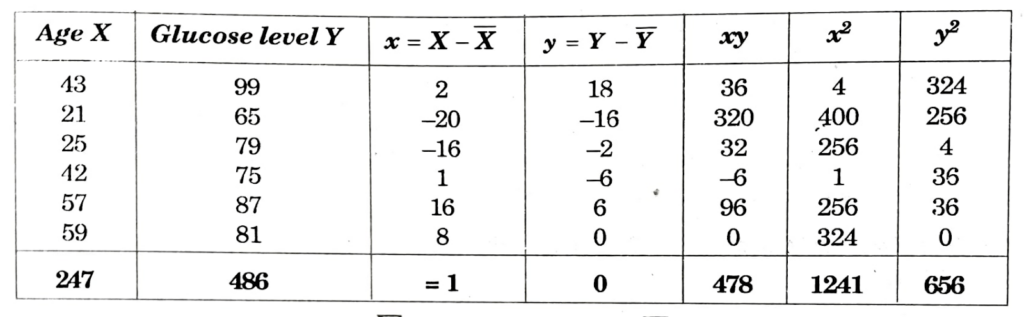
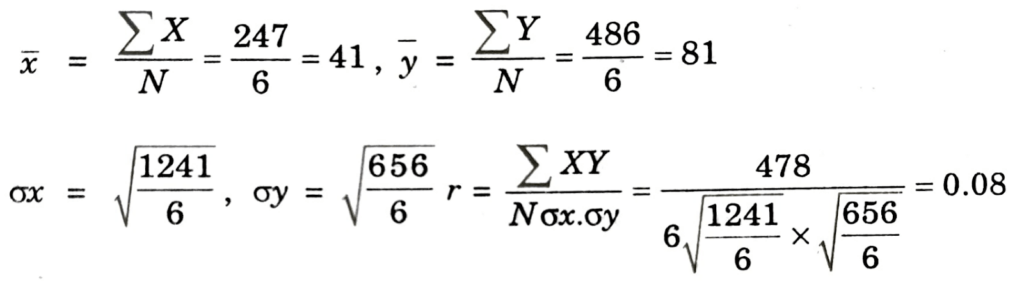
The value of r = 0.08 shows very low positive correlation between age and glucose levels.
Q.4. The values of the same 15 students in two subjects A and B are given below; the two numbers within the brackets denoting the ranks of the same student in A and B respectively.
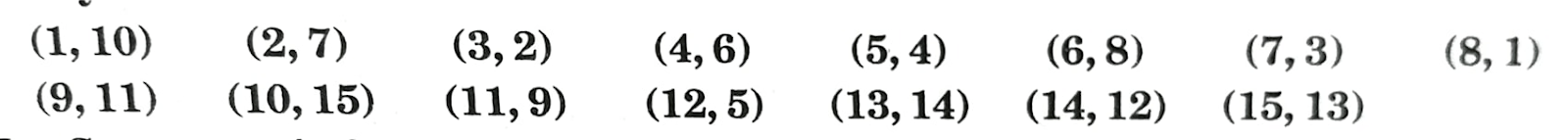
Use Spearman’s formula to find the rank correlation coefficient.
Ans.
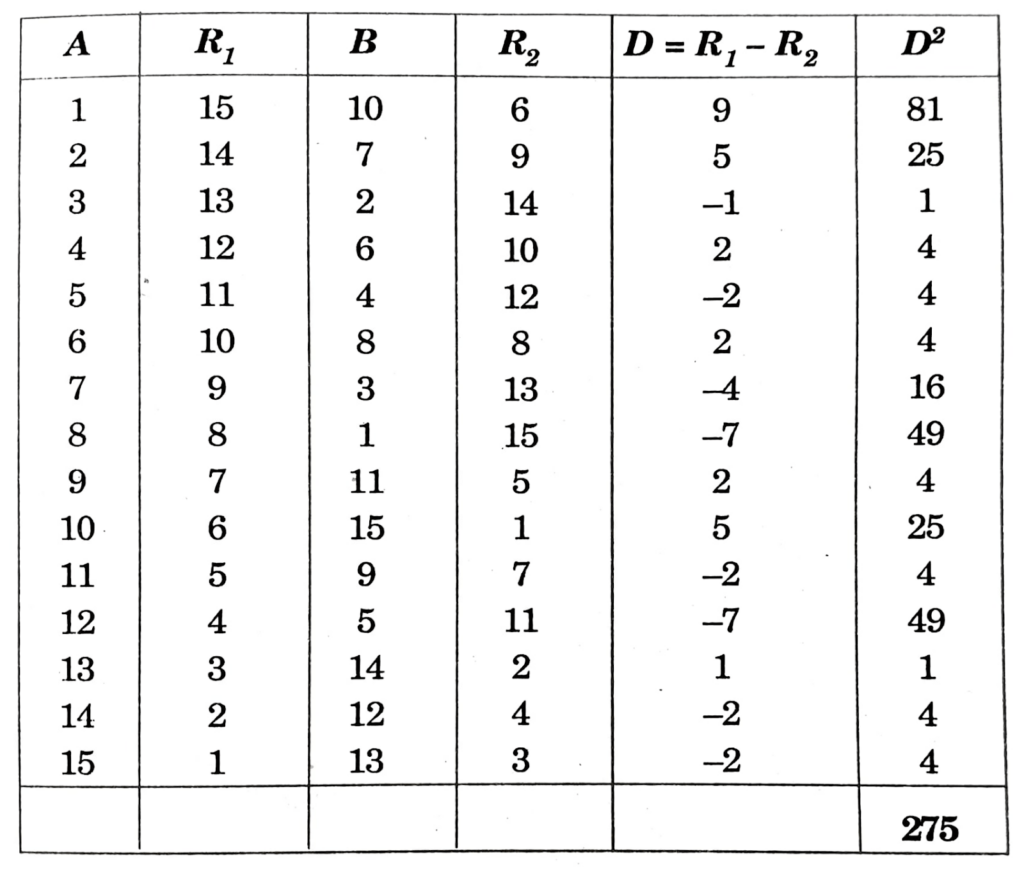
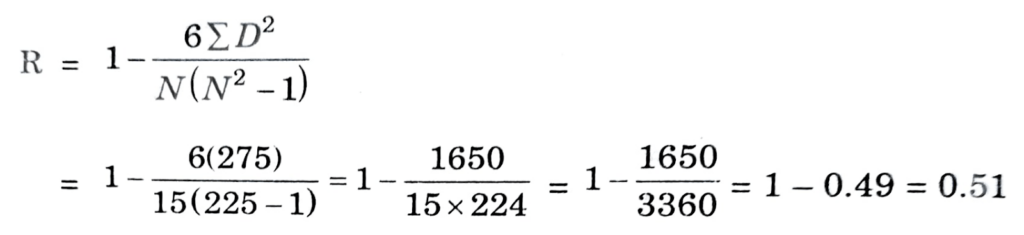
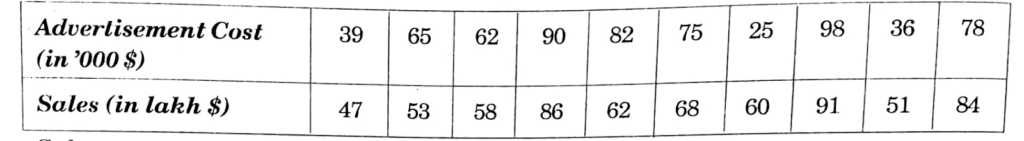
Q.5. Calculate Karl Pearson’s coefficient of correlation from the advertisement cost and sales as per the data given below:
Ans.
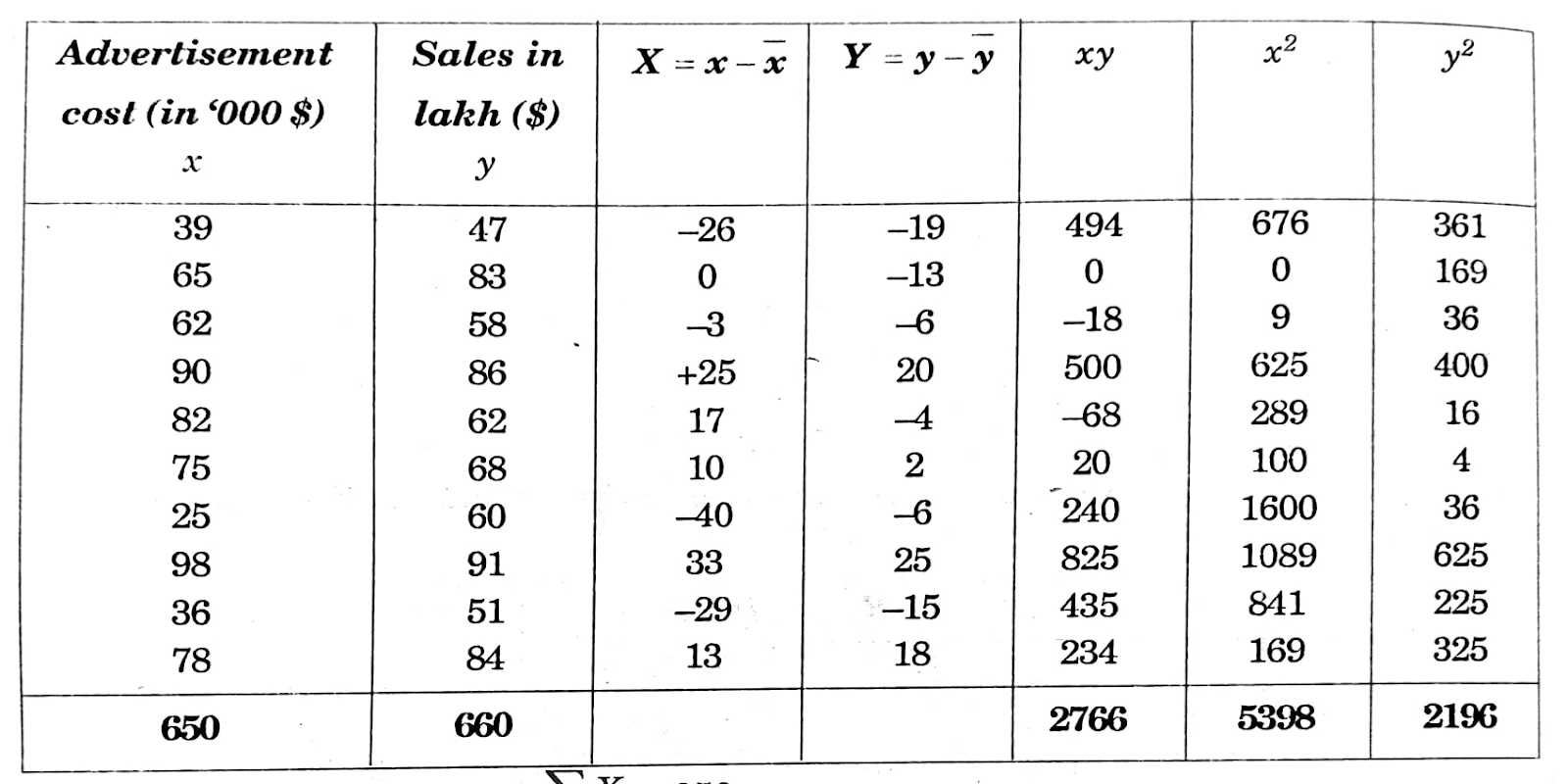
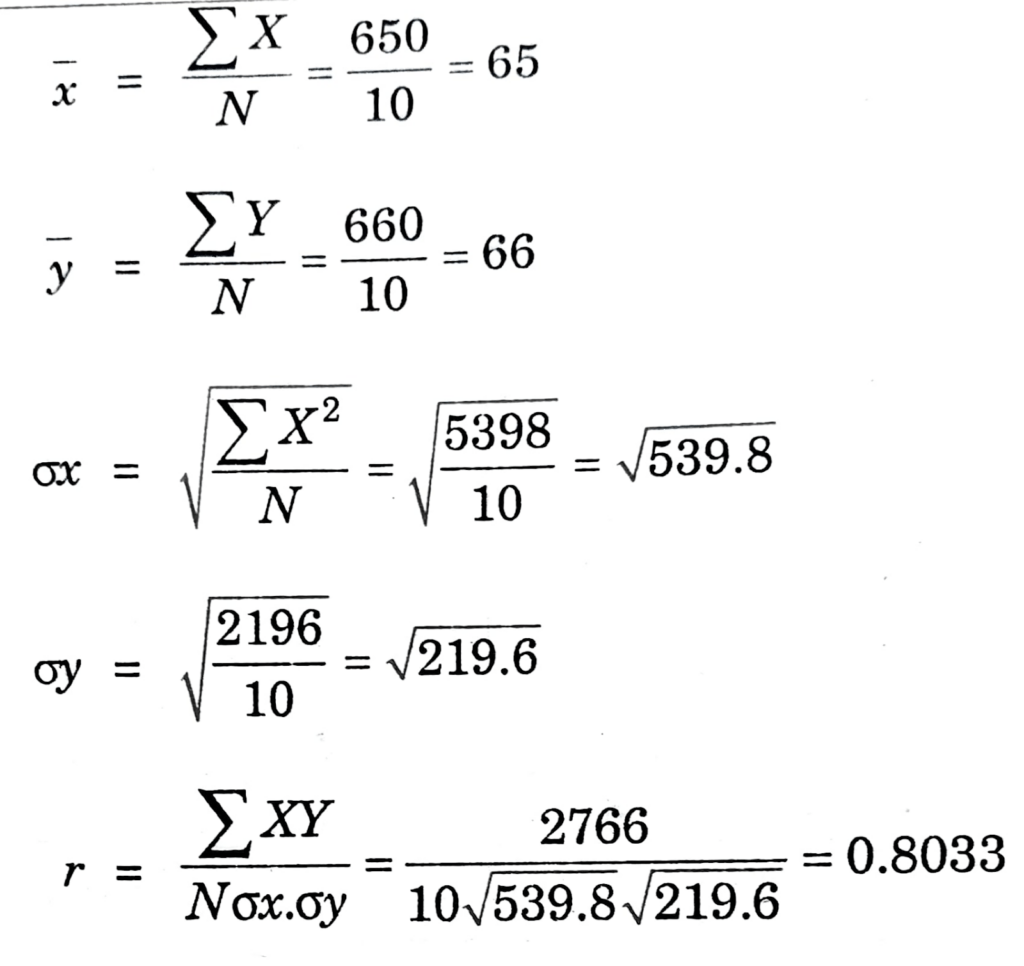
The correlation is positive and moderate.
Q.6. The following observations are given for two variables:
| Y | X |
| 5 | 2 |
| 8 | 12 |
| 18 | 3 |
| 20 | 6 |
| 22 | 11 |
| 30 | 19 |
| 10 | 18 |
| 7 | 9 |
(a) Compute and interpret the sample covariance for the above data.
(b) Compute and interpret the sample correlation coefficient.
Ans.
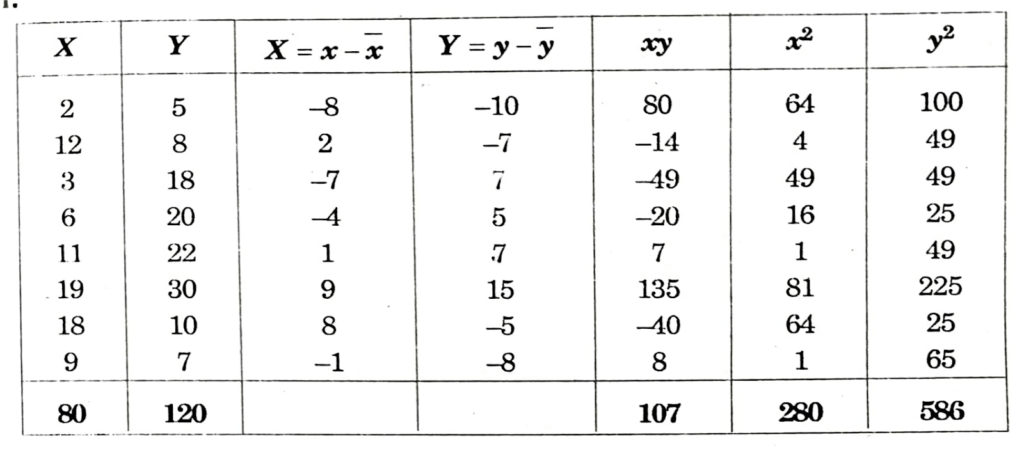
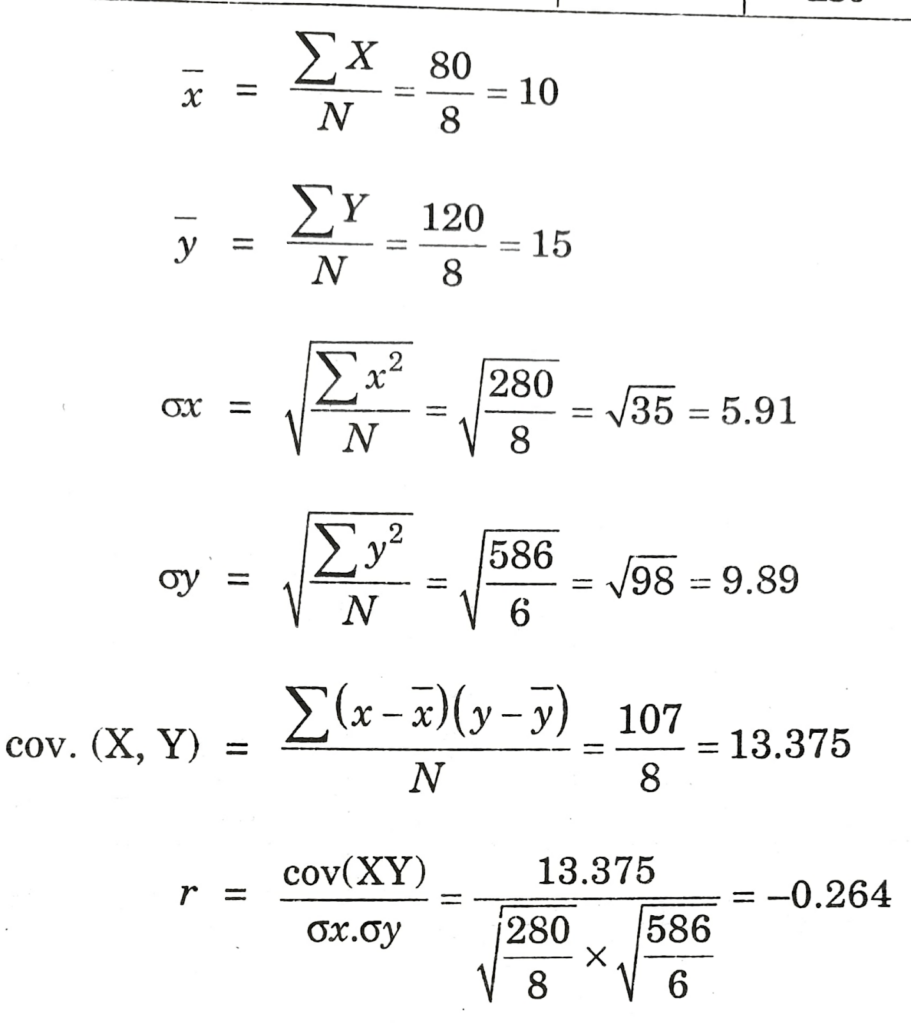
Q.7 A trainee manager wondered whether the length of time his trainees revised for an examination had any effect on the marks they scored in the examination. Before the exam, he asked a random sample of them to honestly estimate how long, to the nearest hour, they had spent revising. After the examination he investigated the relationship between the two variables.
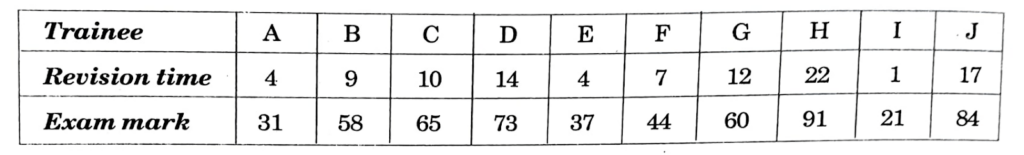
(a) Plot the scatter diagram in order to inspect the data.
(b) Calculate the correlation coefficient.
Ans. (a) Revision time X₁ and Exam mark Y
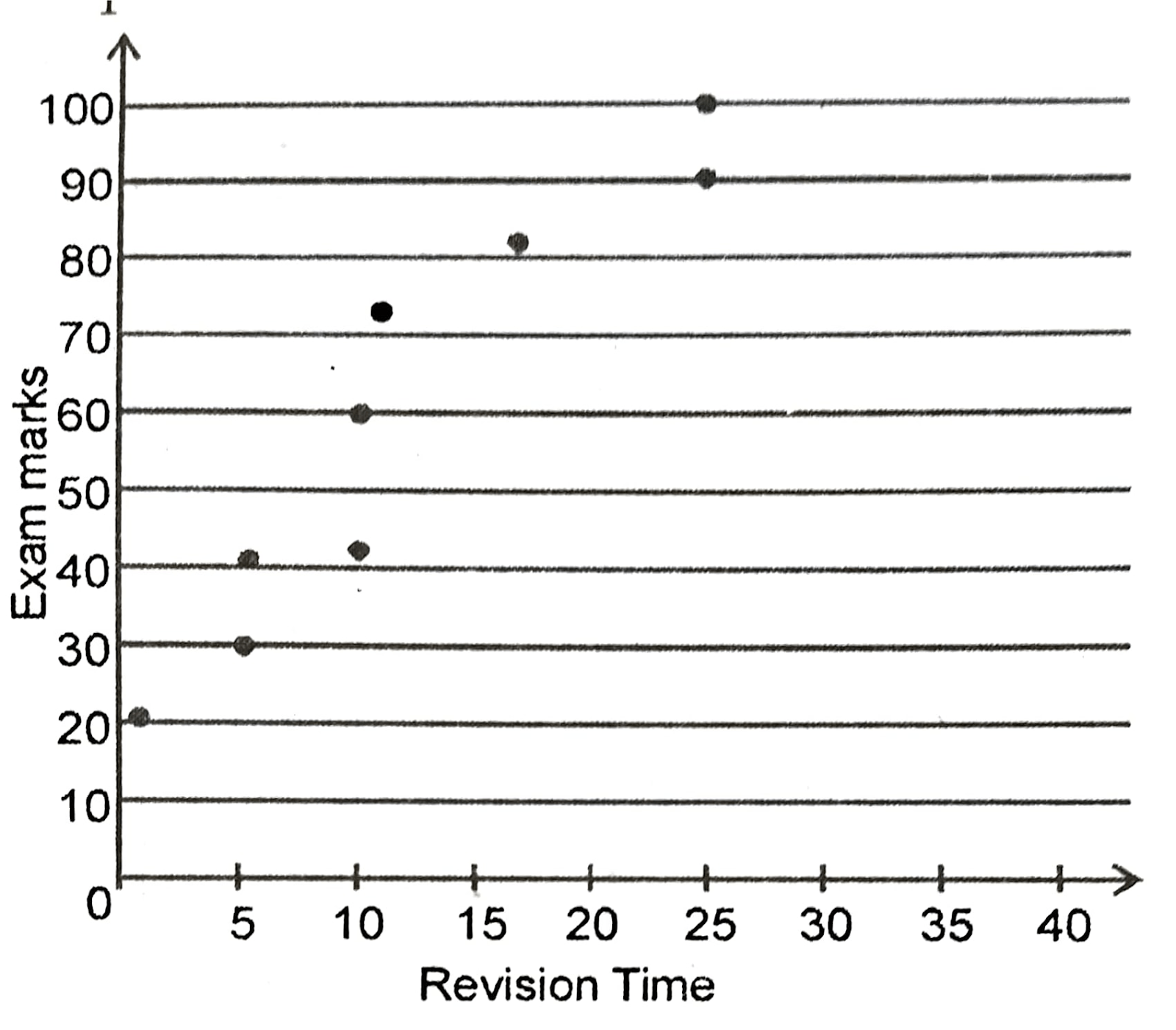
(b)
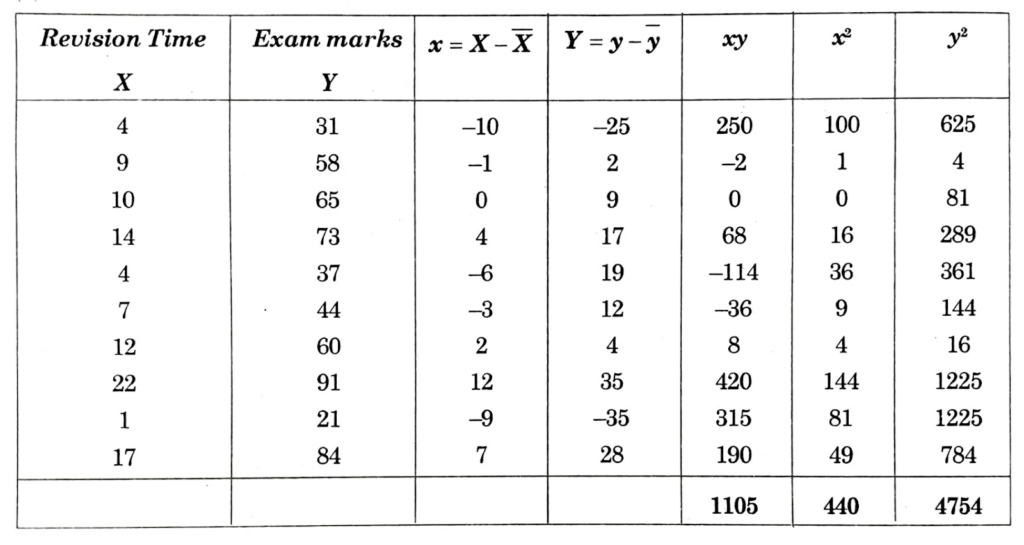
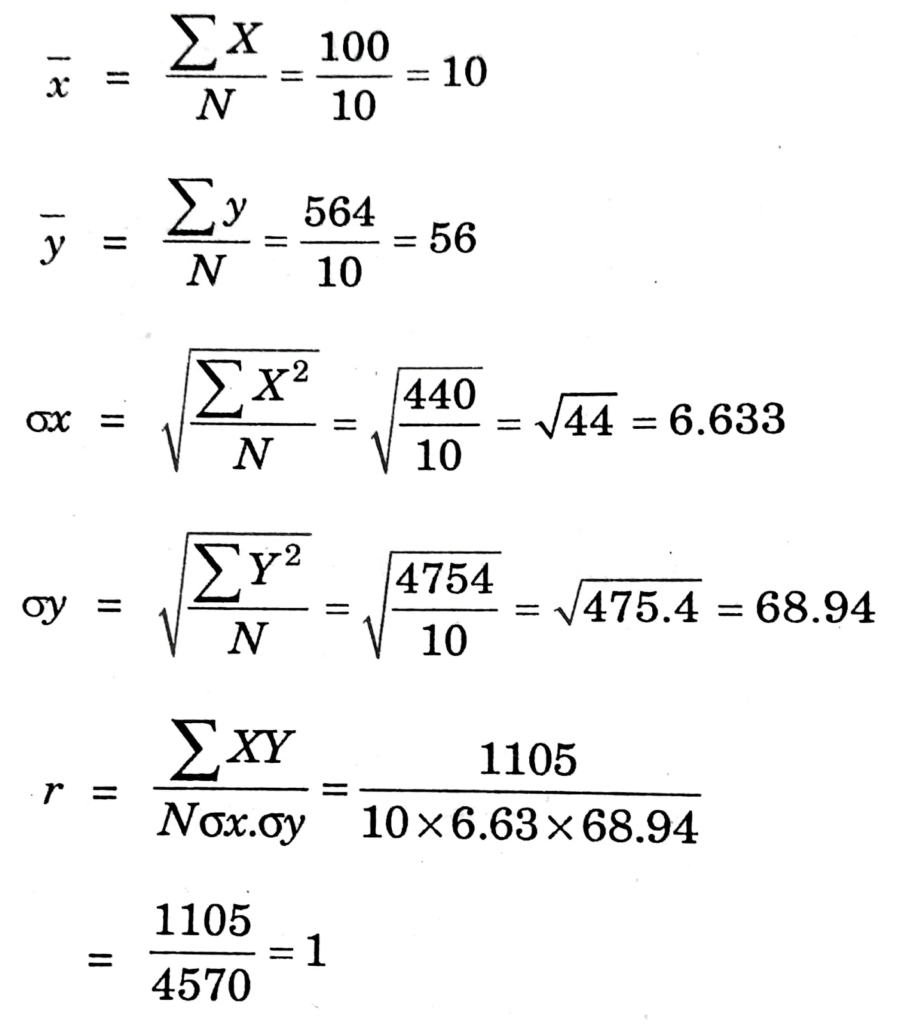
Q.8. Positive values of covariance indicate:
(a) A positive variance of the x values.
(b) A positive variance of the y values.
(c) The standard deviation is positive.
(d) Positive relation between two variables.
Ans. (d) positive relation between two variables.
Q.9. A numerical measure of linear association between two variables is the:
(a) Variance.
(b) Coefficient of variation.
(c) Correlation coefficient.
(d) Standard deviation.
Ans. (c) Correlation coefficient.
Q.10. The coefficient of correlation ranges between:
(a) 0 and 1.
(b) -1 and +1.
(c) Minus infinite and plus infinity.
(d) 1 and 100.
Ans. (c) Minus infinite and plus infinity.
Q.11. The coefficient of correlation:
(a) Can be larger than 1.
(b) Cannot be larger than 1.
(c) Cannot be negative.
(d) None of these.
Ans. (a) Can be larger than 1.
Q.12. If height is independent of average yearly income, what is the predicted correlation between these two variables?
(a) 1
(b) -1
(c) 0
(d) Impossible to say for sure.
Ans. (c) 0.
Q.13. A student produces a correlation of + 1.3. This is:
(a) A high positive correlation.
(b) A significant correlation.
(c) An impossible correlation.
(d) Only possible if N is large.
Ans. (a) A high positive correlation.
Q.14. What sort of correlation would be expected between a company’s expenditure on health and safety and the number of work related accidents.
(a) Positive.
(b) Negative.
(c) None.
Ans. (b) Negative.
Q.15. If A scored the top mark in the apprentices test on computing and the correlation between that test and the test on English language was +1.0, what position did A get in the test on English language?
(a) Middle.
(b) Bottom.
(c) Top.
(d) Cannot say from the information given.
Ans. (c) Top.
Q.16. Which correlation is the strongest + 0.65 or – 0.70
(a) -0.70
(b) +0.65
(c) Depends on N.
(d) Cannot say from the information given.
Ans. (b) + 0.65.
Q.17. The symbol for the Karl Pearson Correlation Coefficient is:
(a) Σ
(b) σ
(c) a
(d) r
Ans. (d) r.
Q.18. When “r” is negative, one variable increase in value, _________.
(a) The other increases.
(b) The other increases at a greater rate.
(c) The other variable decreases in value.
(d) There is no change in the other variable.
(e) All of the above.
Ans. (c) The other variable decrease in value.
Q.19. If two variable are absolutely independent of each other the correlation between them must be:
(a) -1
(b) 0
(c) +1
(d) +0.1
Ans. (b) 0.
Q.20. For a normal good, if price increases then the quantity demanded decreases. What type of correlation coefficient would you expect in this situation?
(a) 0
(b) Positive.
(c) 0.9
(d) Negative.
(e) Unknowable.
Ans. (d) negative.
Some Other Important Questions For Examinations
Very Short Answer Type Questions
Q.1. Define correlation.
Ans. According to Croxton and Cowden, correlation is defined as “When the relationship is of a quantitative nature, the appropriate statistical tool for discovering and measuring the relationship and expressing it in a brief formula is known as correlation.”
Q.2. What are the principal methods of calculating coefficient of correlation?
Ans. The principal methods are as under:
(i) Scattered Diagram Method.
(ii) Karl Pearson’s Coefficient of Correlation.
(iii) Spearman’s Rank Correlation Coefficient.
Q.3. What is the difference between positive and negative correlation?
Ans. When two variables move in the same direction, such a relation is called positive correlation. For example, the relationship between price and supply. When two variables change in different directions, it is called negative correlation. For example, relationship between price and demand.
Q.4. State the kinds of correlation.
Ans. (a) Positive and negative correlation.
(b) Linear and non-linear correlation.
(c) Simple and multiple correlation.
Q.5. Coefficient of correlation is between -1 and +1. How would you express it arithmetically?
Ans. – 1 < r < + 1.
Q.6. What is the nature of correlation of two variables when they move in the same direction?
Ans. Positive correlation.
Q.7. What is the principal shortcoming of scattered diagram as a method of estimating correlation?
Ans. A scattered diagram does not measure the precise extent of correlation. It gives only an approximate idea of the relationship. It is not a quantitative measure of the relationship.
Q.8. When is Rank Correlation method used?
Ans. Rank Correlation method is used for the variables whose quantitative measurement is not possible, such as beauty, bravery, wisdom etc.
Q.9. What does correlation measure?
Ans. Correlation measures the direction and intensity of relationship. It measures covariation and not causation.
Q.10. What does the presence of correlation between two variables X and Y simply mean?
Ans. The presence of correlation between two variables X and Y simply means that when the value of one variables is found to change in one direction, the value of other variable is found to change either in the same direction (i.e. positive direction) or in the opposite direction (i.e. negative direction) but in a definite way.
Q.11. Define negative correlation.
Ans. Negative correlation: When the changes in the values of two series are in opposite direction, there will be negative correlation. For example, if the prices are increasing demand is decreasing.
Q.12. When do r and rₖ give identical results?
Ans. r and rₖ give identical results when the first differences of the values of the items in the series arranged in the order of magnitude are constant.
Q.13. Which type of correlation is indicated by the following scatter diagram?
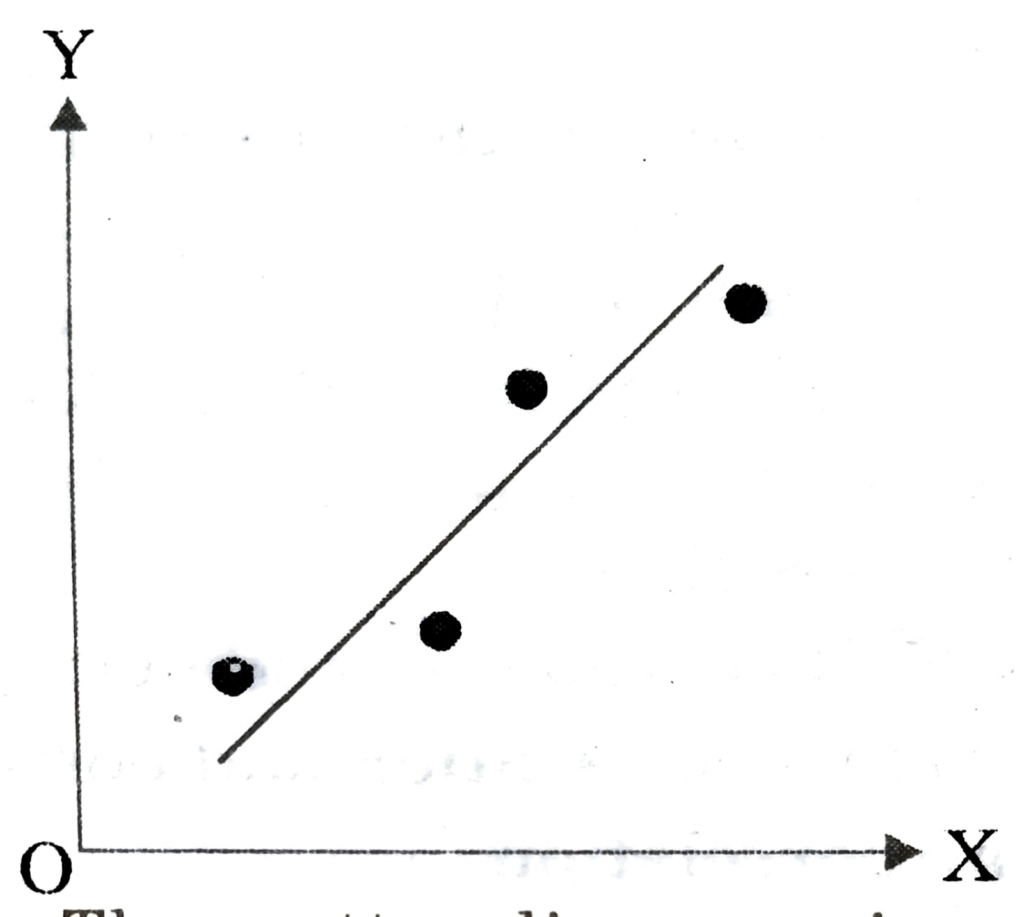
Ans. The scatter diagram given in the question indicates the positive relation.
Q.14. Which type of correlation is indicated by the values of X and Y variables?
| X | 30 | 35 | 40 | 50 | 55 | 60 |
| Y | 80 | 90 | 100 | 110 | 120 | 140 |
Ans. Here r = -1 as the values of X and Y variables move in the same direction.
Q.15. What does a high value of r indicate?
Ans. A high value of r indicates linear relationship. Its value is said to be high when it is close to +1 or -1.
Q.16. Give the formula of ranking coefficient of correlation of Spearman.
Ans.
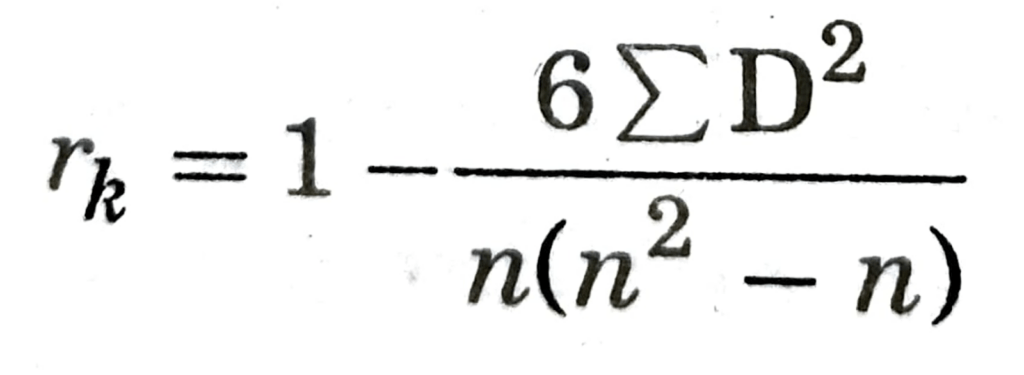

Hi! my Name is Parimal Roy. I have completed my Bachelor’s degree in Philosophy (B.A.) from Silapathar General College. Currently, I am working as an HR Manager at Dev Library. It is a website that provides study materials for students from Class 3 to 12, including SCERT and NCERT notes. It also offers resources for BA, B.Com, B.Sc, and Computer Science, along with postgraduate notes. Besides study materials, the website has novels, eBooks, health and finance articles, biographies, quotes, and more.



