NIOS Class 12 Economics Chapter 11 Index Numbers, Solutions to each chapter is provided in the list so that you can easily browse through different chapters NIOS Class 12 Economics Chapter 11 Index Numbers and select need one. NIOS Class 12 Economics Chapter 11 Index Numbers Question Answers Download PDF. NIOS Study Material of Class 12 Economics Notes Paper 318.
NIOS Class 12 Economics Chapter 11 Index Numbers
Also, you can read the NIOS book online in these sections Solutions by Expert Teachers as per National Institute of Open Schooling (NIOS) Book guidelines. These solutions are part of NIOS All Subject Solutions. Here we have given NIOS Class 12 Economics Chapter 11 Index Numbers, NIOS Senior Secondary Course Economics Solutions for All Chapters, You can practice these here.
Index Numbers
Chapter: 11
Module – IV Statistical Tools
TEXT BOOK QUESTIONS WITH ANSWERS
INTEXT QUESTIONS 11.1.
Q.1. The Paasche Index Number is based on:
(a) Base Year Quantity.
(b) Current Year Quantity.
(c) Average of Base and Current Year Quantity.
(d) none of the above.
Ans. (a) Base Year Quantity.
Q.2. What is an index number?
Ans. An index number is a statistical measure, designed to measure changes in a variable, or group of related variables. Index number is a single ratio (or a percentage) which measures the combined change of several variables between two different times, places or situations. Index number express the relative change in price, quantity or value compared to a base period. It is used to measure change in prices, paid for raw materials, numbers of employees and customers, annual income and profit etc.
Q.3. Write one use of index numbers.
Ans. Index numbers are used in studying trends and tendencies. Since index numbers are most widely used for measuring changes over a period of time, the time series so formed enable us to study the general trend of the phenomenon under study.
Q.4. State any two characteristics of index number.
Ans. (i) Index numbers are expressed in terms of percentage to show the extent of relative change.
(ii) Index numbers measure relative changes. They measure the relative change in the value of a variable or a group of related variables over a period of time or between places.
INTEXT QUESTIONS 11.2.
Q.1. From the following data compute an index number by using weighted average or relative method:
| Commodities | Base Year Prices (in ₹) | Current Year Price (in ₹) | Weights (W) |
| A | 100 | 90 | 30 |
| B | 20 | 20 | 15 |
| C | 7 | 60 | 20 |
| D | 20 | 15 | 10 |
| E | 40 | 55 | 25 |
Ans.
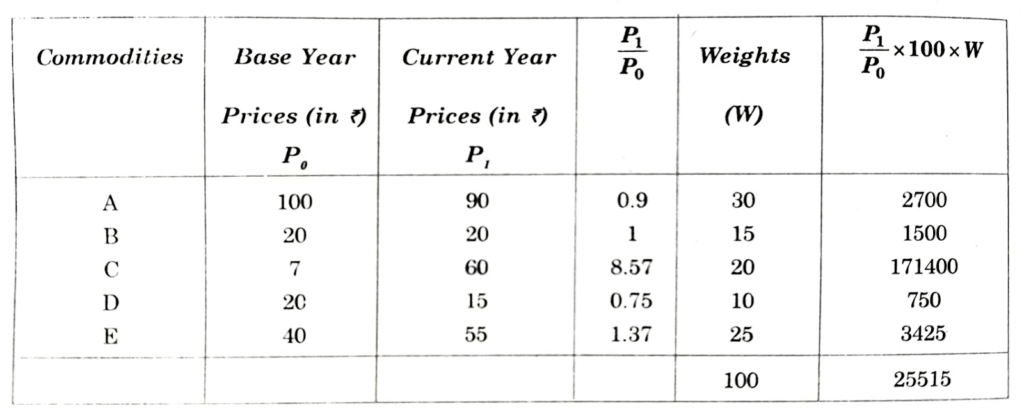
P₀₁ (Weighted Arithmetic Mean)
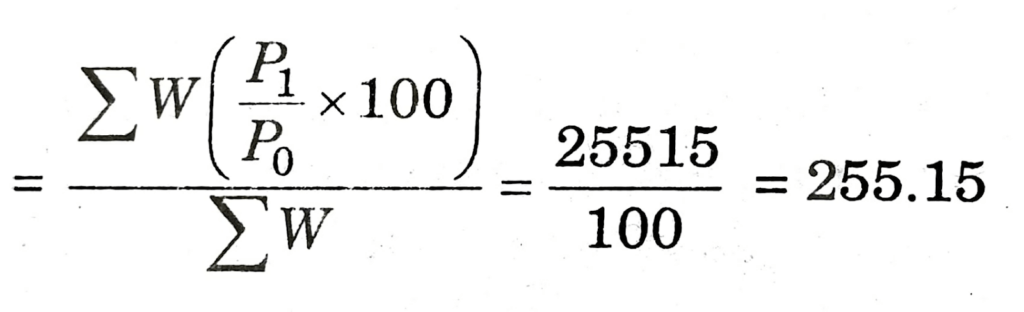
INTEXT QUESTIONS 11.3.
Q.1. Suppose a person was earning 1500 per month in 2005, what should be his salary in 2010, if the cost of living index number in 2010 with base year 2005 is 170.30?
Ans.
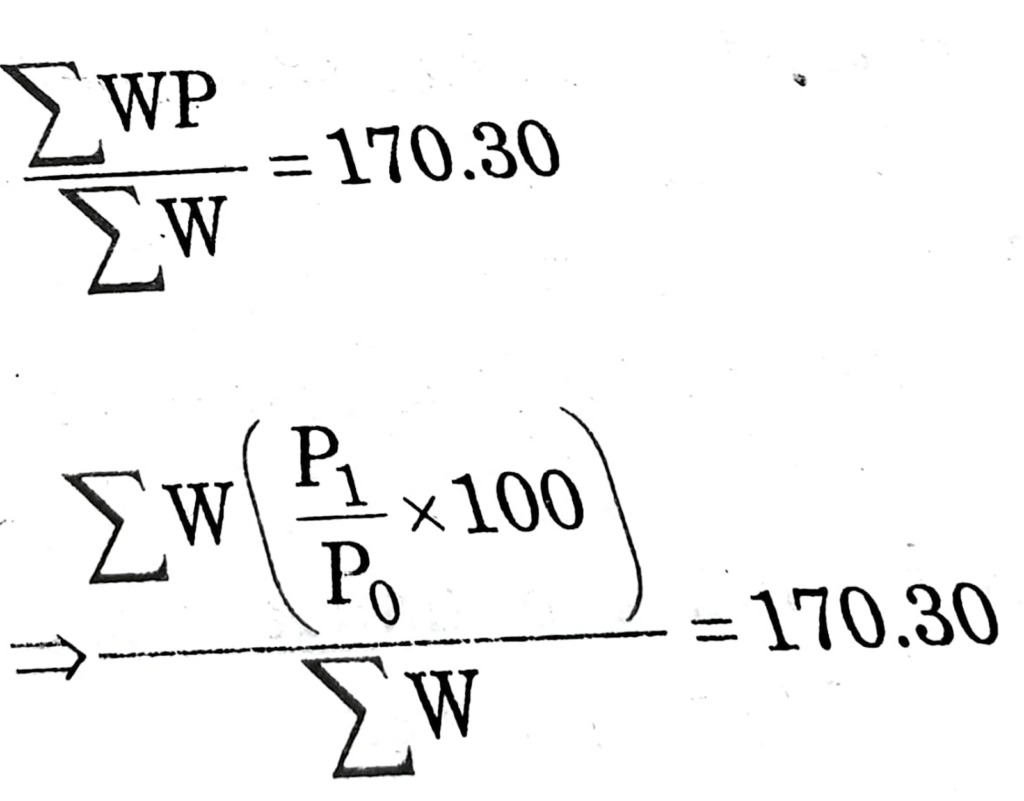
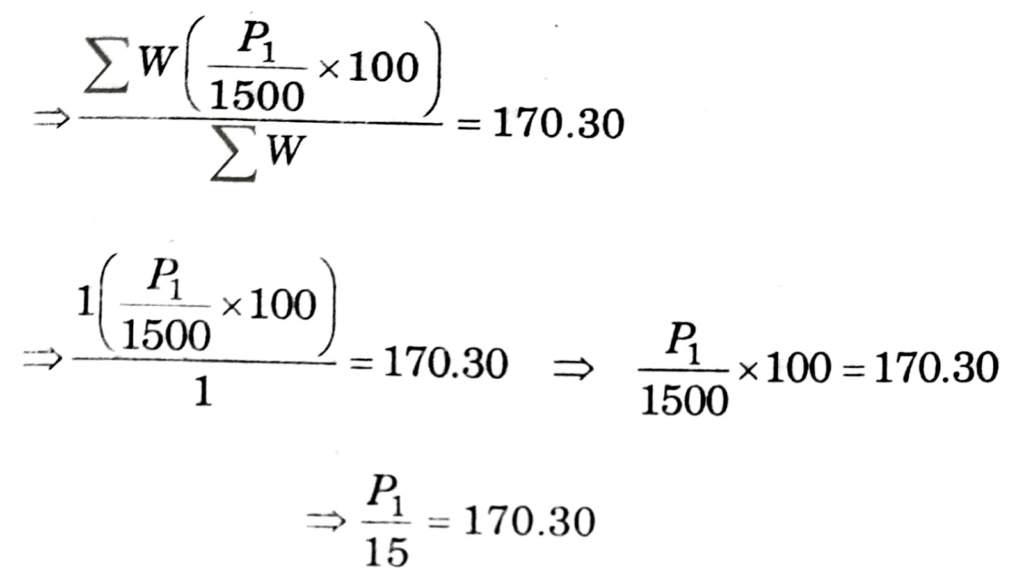
= P₁ = 15 x 170.30 = 2554.5
∴ Salary in 2010 is 2554.5 per month.
TERMINAL EXERCISE
Q.1. Use the following to answer question a – d:
A company buys four products with the following characteristics:
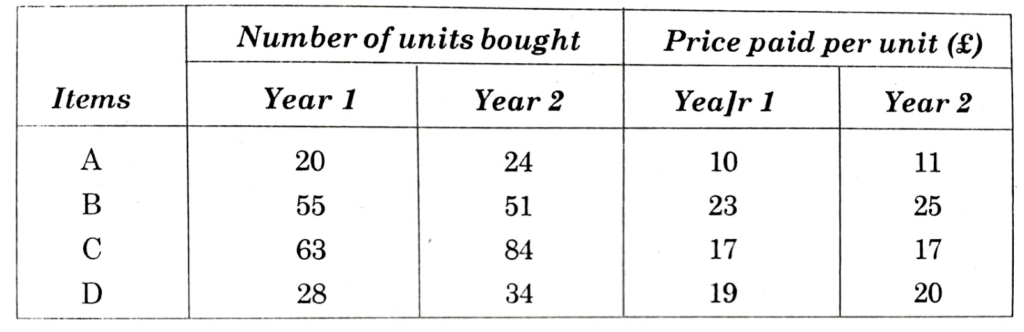
(a) Find the simple price indexes for the products for year 2 using year l as the base year.
Ans.
| Items | Previous year 1 P₀ | Previous year 2 P₁ |
| A | 10 | 11 |
| B | 23 | 25 |
| C | 17 | 17 |
| D | 19 | 20 |
| 69 | 73 |
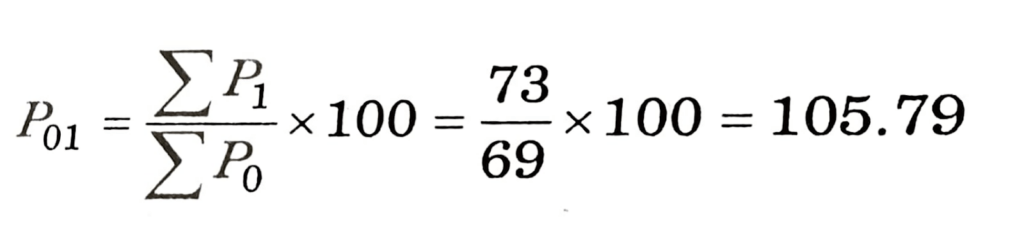
(b) Find the simple aggregate index for year 2 using year I as the base year.
Ans.
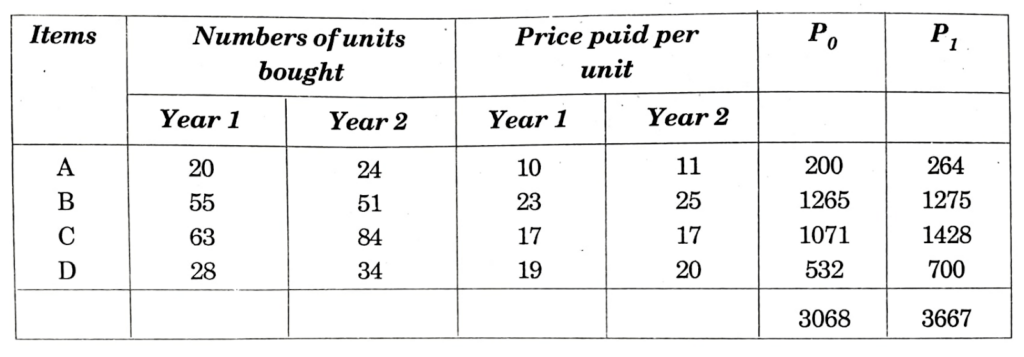
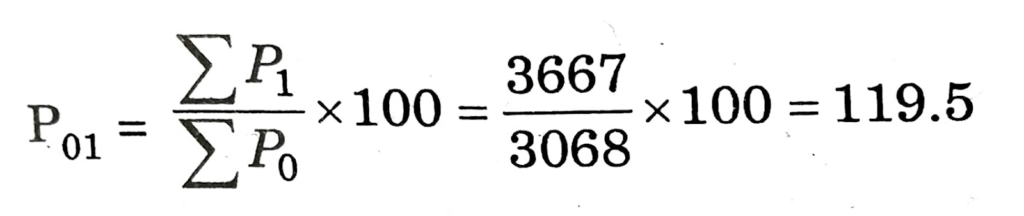
(c) Find the base-weighted aggregate index, the Laspeyres index for year 2 using year 1 as the base year.
Ans:
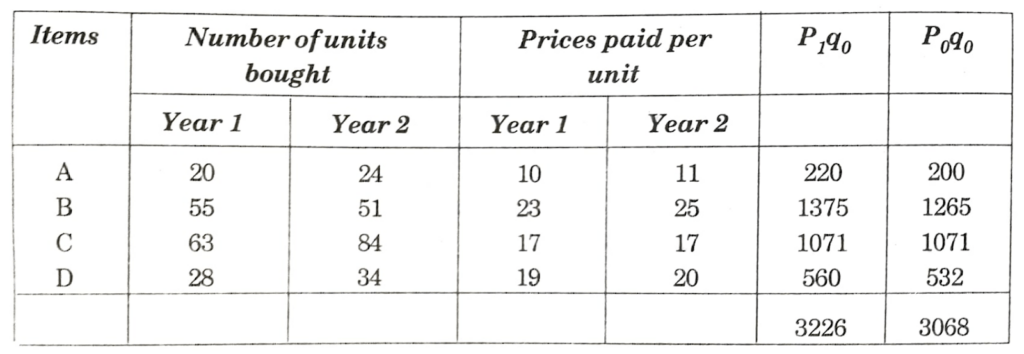
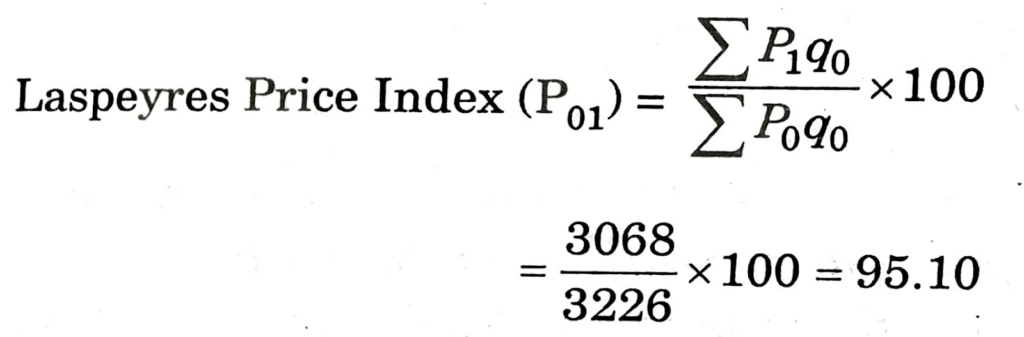
(d) Find the current period-weighted aggregate index, the paasche index, for year 2 using year 1 as the base year.
Ans.
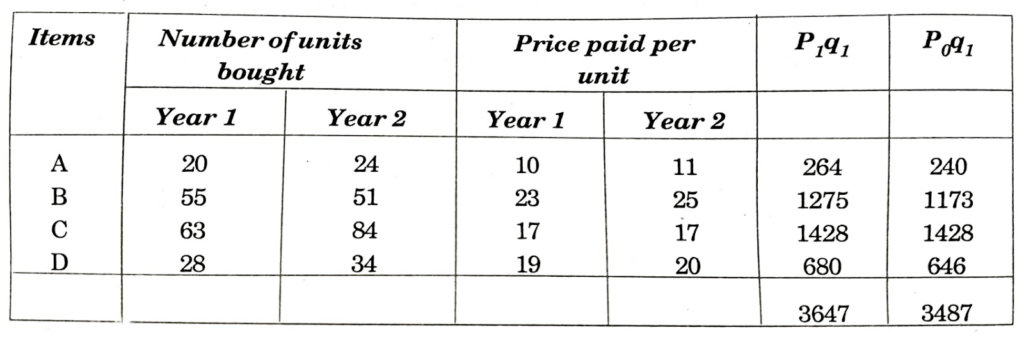
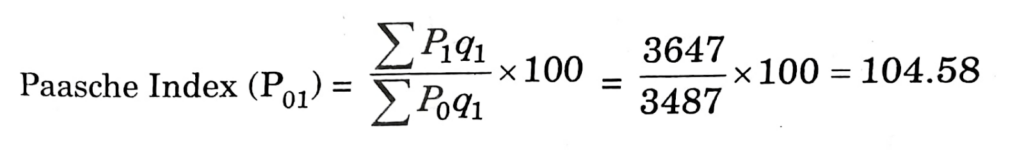
Q.2. During a certain year, Cost of Living Index Number goes up from 110 to 200 and the salary of worker is also raised from 3250 to 5000. Does the worker really gain?
Ans. Number goes up from 110 to 200
∴ 200 – 110/110 x 100 = 81.8% increase in cost of living index.
Percent increase in salary = 5000 – 3250/3250 x 100
= 53.84%
The worker has not gained since the increase in the cost of living index is more than the per unit increase in salary.
Q.3. The price relatives and weights of the set of commodities are given in the following table:
| Commodities | A | B | C | D |
| Price Relatives | 125 | 120 | 127 | 119 |
| Weights | W1 | 2W1 | W2 | W2+3 |
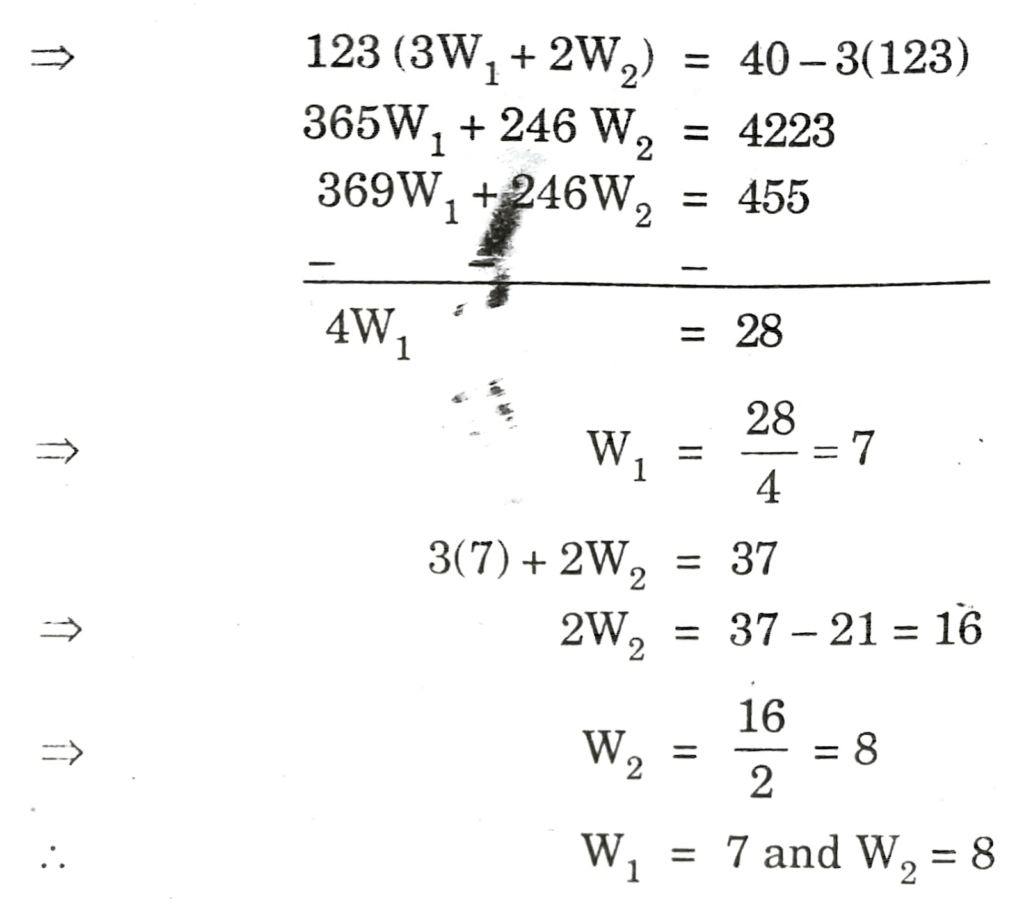
If the sum of weights is 40 and the index number for the set is 122, find the numerical value of W₁ and W₂
Ans.
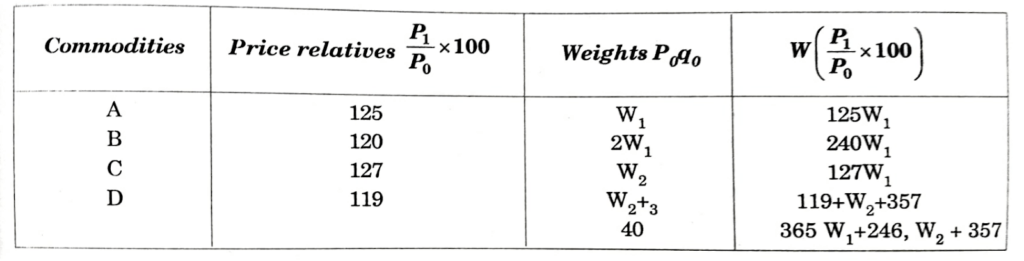
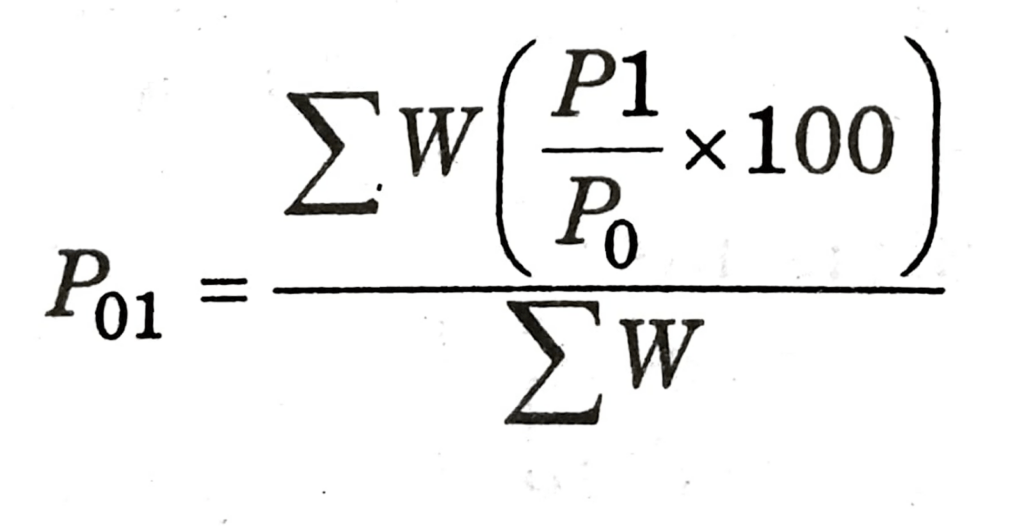
= 365W₁ + 246W₂ + 357/ 40 =122
= 365W₁ + 246 W₂ + 357 = 122 x 40
= 365 W₁ + 246 W₂ = 4880 – 357 = 4523
= 365 W₁ + 246 W₂ = 4523
= 123 (3W₁ + 2W₂) = 4523
Some Other Important Questions For Examinations
Very Short Answer Type Questions
Q.1. Define an index number.
Ans. An index number can be defined as a special type of average which measures changes in the value of such variables as prices of commodities. For example, Industrial production, cost of living etc.
Or
An index number is a statistical measure designed to show change in a variable or group of related variables with respect to time, geographic location or other characteristics.
Q.2. What is the difference between simple index number and weighted index number?
Ans. In the simple index number all items of the series are treated as of equal importance. In the weighted index, weights are accorded to different items depending on their relative importance.
Q.3. What should be the base year like?
Ans. The base year should be the year of economic stability. It should not to be too distant from the current year.
Q.4. Define price index number.
Ans. Price index number is that number which measures and permits comparison of the prices of certain goods.
Q.5. State the two types of price index numbers.
Or
Write down the widely used index numbers.
Ans. Widely used index numbers are whole-sale price index number, consumer price index, index for industrial production, agricultural production index and sensex.
Q.6. How is the rate of inflation calculated?
Ans. Rate of inflation is calculated through following formula:
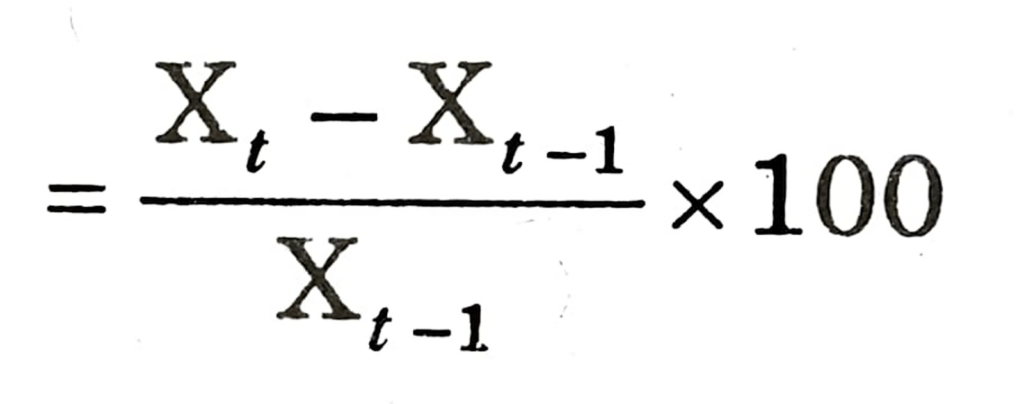
Q.7. Name the major groups for whom the consumer price index number are constructed in India.
Ans. Major groups for whom the consumer price index number are constructed in India are following:
1. The industrial workers.
2. The urban non-manual workers.
3. The agricultural labourers.
Q.8.What does the consumer price index for industrial workers measure?
Ans. The consumer price index for industrial workers measures general inflation.
Q.9. Define simple index number.
Ans. Simple Index Number: Index number in which all items of the series are accorded equal weightage or importance is called simple index number. It will be simple average of the price of different goods and services.
Q.10. Define weighted index numbers.
Ans. Weighted Index Numbers: These are the index numbers in which different items are given different weights. This index number is not a simple average of items and facilities but it is weighted average of the cost.
Q.11. What is price relative?
Ans. A price relative is the percentage ratio of the value of a variable in the current year to its value in the base year.
Price Relative, P₀₁
= Current Year Price (P₁)/Base Year Price (P₀) x 100
Q.12. Define wholesale price index.
Ans. Wholesale Price Index: It measure the relative changes in the price of commodities traded in the wholesale markets. It does not include services.
Q.13. Write down any two characteristics of index numbers.
Ans. 1. Index numbers are expressed in percentage.
2. They are specialised types of averages.
Q.14. What is the base year of the new series of wholesale price index numbers published by the Govt. of India?
Ans. 1993 -1994 Price Relative, P₀₁
Current Year Price (P₁)/Base Year Price (P₀) x 100
Short Answer Type Questions
Q.1. Define Price Index.
Ans. Index number is a statistical measure showing percentage or relative changes in variables like prices, production, exports etc.
According to Spiegel: An index number is a statistical measure designed to show changes in a variable or group of related variables with respect to time, geographic location or other characteristics.
Q.2. Give utility of index numbers.
Or
Why do we need an index number? State any four reasons.
Ans. Utility of Index Numbers:
1. Index numbers are helpful to policy makers.
2. Index numbers simplify the facts.
3. Comparative study become easy.
4. Index number help us to study the general trend.
5. They are helpful in the determination of real change in income.
Q.3. What are the limitations of index numbers?
Ans. In the construction of index numbers, there are some practical difficulties and theoretical limitations.
1. Index numbers are not fully true. They simply indicate arithmetical tendency of the temporal change in the variable.
2. Index numbers do not help in international comparison.
3. Index numbers are prepared with certain specific objectives.
4. It is difficult to collect retail prices. So index numbers based on wholesale prices may be misleading.
Q.4. State the qualities of a good base year.
Or
What are the considerations underlying the choice of base period in the construction of an index number?
Ans. Basic considerations underlying the choice of base period:
1. The base period should be a normal period. Abnormal periods like period of war or floods etc. should not be selected as a base year.
2. The base period should be neither too short nor too long.
3. It should not be the period for which actual data are not available.
4. It should not be too far back in the past.
Q.5. Discuss the simple Aggregative Price Index. What are its limitations?
Ans. A Simple Aggregative Price Index is defined as
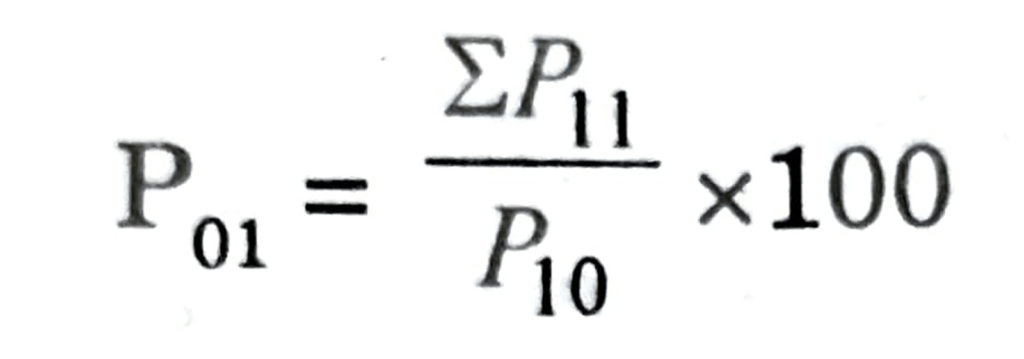
Which is the sum of prices of commodities in the current period. ‘I’ expressed as the sum of prices in the base year ‘0’.
Limitations:
1. It has limited applicability.
2. No weightage is given to the relative importance of items.
3. Index is influenced by the items with the large unit prices.
