NIOS Class 12 Economics Chapter 6 Collection And Classification of Data, Solutions to each chapter is provided in the list so that you can easily browse through different chapters NIOS Class 12 Economics Chapter 6 Collection And Classification of Data and select need one. NIOS Class 12 Economics Chapter 6 Collection And Classification of Data Question Answers Download PDF. NIOS Study Material of Class 12 Economics Notes Paper 318.
NIOS Class 12 Economics Chapter 6 Collection And Classification of Data
Also, you can read the NIOS book online in these sections Solutions by Expert Teachers as per National Institute of Open Schooling (NIOS) Book guidelines. These solutions are part of NIOS All Subject Solutions. Here we have given NIOS Class 12 Economics Chapter 6 Collection And Classification of Data, NIOS Senior Secondary Course Economics Solutions for All Chapters, You can practice these here.
Collection And Classification of Data
Chapter: 6
Module – III: Introduction to Statistics
TEXT BOOK QUESTIONS WITH ANSWERS
INTEXT QUESTIONS 6.1.
Q.1. Fill in the blanks with suitable word given in brackets against each:
(a) _______ data are original. (Primary, Secondary)
Ans. Primary.
(b) Primary data are collected by the ________ himself. (respondent, investigator)
Ans. Investigator.
(c) CSO publishes data on ________ (national income, population)
Ans. National income.
Q.2. State whether the following statements are true or false:
(a) Secondary data are collected by the investigator himself.
Ans. False.
(b) Reserve Bank of India Bulletin represents an unpublished source of data.
Ans. False.
(c) A person from whom an investigator tries to get information is called respondent.
Ans. True.
INTEXT QUESTIONS 6.2.
Q.1. Fill in the blanks with appropriate words from the brackets:
(a) A simple array is an arrangement of data in ________ (only ascending order, only descending order, either ascending or descending order).
Ans. Either ascending or descending order.
(b) Organising data in simple array is convenient if number of items are ________. (large, small)
Ans. Small.
(c) Arranging the data in the form of ________ array is more convenient if number of items are large. (simple, frequency).
Ans. Frequency.
(d) Frequency array ________ the idea of characteristics of a group. (gives, does not give).
Ans. Does not give.
INTEXT QUESTIONS 6.3.
Q.1. Fill in the blanks with appropriate word from the brackets:
(a) Frequency distribution _________ data into groups. (classifies, does not classify)
Ans. Classifies.
(b) The difference between two limits of a class is called ________ (class limit, class interval).
Ans. Class-interval.
(c) In the exclusive type frequency distribution an item having value equal to the upper limit is counted in the ________ class. (same, next)
Ans. Next.
(d) In the inclusive type frequency distribution an item having value equal to the upper limit is counted in the _______ class. (same, next)
Ans. Same.
(e) Preparing a frequency distribution by taking ‘successive totals’ of frequencies is called _________ frequency distribution. (open-end, cumulative)
Ans. Cumulative.
TERMINAL EXERCISE
Q.1. Distinguish between primary and secondary data. Describe the methods for collecting primary data.
Ans. Primary Data and Secondary Data: Statistical data may be classified as primary and secondary. Primary data are those statistical informations which are directly collected from the respondent. They are original because they are collected for the first time by the investigator himself. Secondary data are those which are already collected by someone for some purpose and are available for the study.
Difference:
1. Primary data are collected for specific objective by itself. The secondary data are collected by others for their objective.
2. The collection of primary data takes money and time both while secondary data is obtained without sacrificing money and time.
3. There is no need to take precaution while using primary data but secondary data cannot be used without precaution.
Methods for Collecting Primary Data: There are several methods for collecting primary data. Some of which are:
(i) Direct Personal Interview: In this method investigator (also called interviewer) has to be face-to-face with the person from whom he wants information. The persons from whom this information is collected is called respondent.
(ii) Questionnaire method: In this method a question booklet is prepared and sent to the respondent either through post or taken personally to him. This method is appropriate in case where informants are spread over a wide area.
(iii) Indirect and investigation: In this method data are collected through indirect sources. Under this methods questions relating to the inquiry are put to different persons and their answers are recorded. This method is most suitable when the person from whom the information is sought is either unavailable or unwilling.
Q.2. What is secondary data? Name some of its sources in India.
Ans. Secondary data are those which are collected by some other agency and are used for further studies. We can say that data which are not originally collected but rather obtained from published or unpublished sources are known as secondary data. It saves cost and time which is involved in collection of primary data. For example, if the investigator collects the information about the salaries of employees of National Open School from the salary register maintained by its accounts branch, then it is secondary data for him.
Sources of secondary data: Secondary data can be collected by the following two sources:
1. Published Sources: There are certain agencies which collect the data and publish them in the form of either regular journals or reports. These agencies/sources are known as published sources of data.
In India some of the published sources are:
(i) Central Statistical Organisation (CSO): It publishes data on national income, savings, capital formation, etc. in a publication called National Accounts Statistics.
(ii) National Sample Survey Organisation (NSSO): This organisation which is under Ministry of Finance provides data on all aspects of national economy, such as agriculture, industry and labour, etc.
(iii) Reserve Bank of India (RBI): It publishes financial statistics. Its publications are Report on Currency and Finance, Reserve Bank of India Bulletin and Statistical Tables relating to banks in India, etc.
(iv) Labour Bureau: Its publications are Indian Labour Statistics, Indian Labour Year Book and Indian Labour Journal.
(v) Population Census: It is undertaken by the office of the Registrar General, Census of India, Ministry of Home Affairs. It provides us statistics on population.
(vi) Papers and Magazines: Journals like ‘Capital’, ‘Commerce’, ‘Economic and Political Weekly’, ‘Economic Times’, etc. also publish important statistical data.
2. Unpublished Sources: Secondary data are also available from unpublished sources, because all statistical material is not always published. For example, information recorded in various government and private offices, studies made by research scholars etc. can be important sources of secondary data.
Q.3. Distinguish between simple array and frequency array with example.
Ans. Difference between simple array and frequency array:
(i) Simple Array: A simple array is an arrangement of data in ascending or descending order. Let us construct the simple arrays of the data about the marks of 40 students. The data in table A is arranged in ascending order and in table B in descending order.
Table A. Ascending Array of the Marks obtained by 40 students in class
| 20 | 35 | 42 | 47 |
| 25 | 36 | 43 | 48 |
| 27 | 37 | 43 | 48 |
| 28 | 38 | 43 | 49 |
| 30 | 38 | 43 | 50 |
| 31 | 39 | 43 | 51 |
| 32 | 40 | 45 | 53 |
| 33 | 40 | 46 | 54 |
| 34 | 40 | 46 | 56 |
| 35 | 42 | 47 | 58 |
Table B. Descending Array of the Marks obtained by 40 students in class
| 58 | 47 | 42 | 35 |
| 56 | 46 | 40 | 34 |
| 54 | 46 | 40 | 33 |
| 53 | 45 | 40 | 32 |
| 51 | 43 | 39 | 31 |
| 50 | 43 | 38 | 30 |
| 49 | 43 | 38 | 28 |
| 48 | 43 | 37 | 27 |
| 48 | 43 | 36 | 25 |
| 47 | 42 | 35 | 20 |
The above arrays reveal information on two points clearly. One, the highest marks obtained by any student are 58. Two, the lowest marks obtained by any student are 20.
Organising the data in the form of simple array is convenient if number of items is small. As the number of items increases the series becomes too long and unmanageable. As such there is need to condense data. Making a frequency array is one method of condensing data.
(i) Frequency Array: Frequency array is a series formed on the basis of frequency with which each item is repeated in series. The main steps in constructing frequency array are:
(i) Prepare a table with three columns-first for values of items, second for tally sheet and third for corresponding frequency. Frequency means the number of times a value appears in a series. For example in table 6.1 the mark 43 appears five times. So frequency of 43 marks is 5.
(ii) Put the items in first column in a ascending order in such a way that one item is recorded once only.
(iii) Prepare the tally sheet in second column marking one bar for one item. Make er blocks of five tally bars to avoid mistake in counting. Note that every fifth bar is shown by crossing the previous four bars like .
(iv) Count the tally bars and record the total number in third column. This column will represent the frequencies of corresponding items.
Let us now explain construction of frequency array of the marks obtained by 40 students. In table, data about the marks is arranged in an ascending order in first column. It helps find not only the maximum and minimum values but also makes it easy to draw bars.
Now for each mark level make one bar (/) in second column and cross the item from the data.
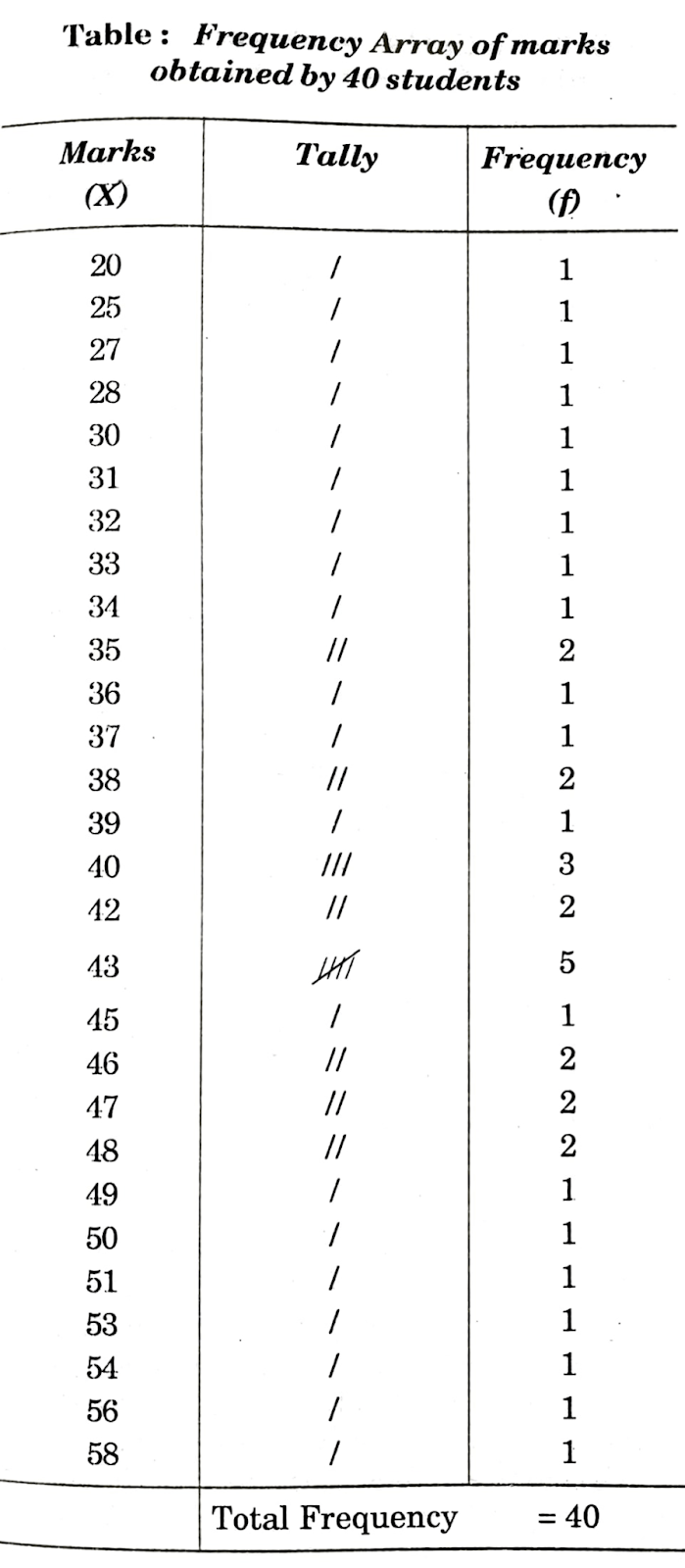
The main limitation of frequency array is that it does not give the idea of the characteristics of a group. For example, it does not tell us that how many students have obtained marks between 40 and 45. Therefore, it is not possible to compare characteristics of different groups. This limitation is removed by frequency distribution.
Q.4. On the basis of the following data about the wages of 20 workers in a factory, prepare a frequency array:
450, 580, 600, 480, 540, 620, 400, 475, 500, 480, 620, 480, 570, 600, 650, 410, 550, 600, 650, 450.
Ans. For preparing a frequency array, we should take the following two steps:
(i) Arrange the data in ascending order:
| 400 | 480 | 550 | 600 |
| 410 | 480 | 570 | 620 |
| 450 | 480 | 580 | 620 |
| 450 | 500 | 600 | 650 |
| 475 | 540 | 600 | 650 |
(ii) Prepare a tally sheet:
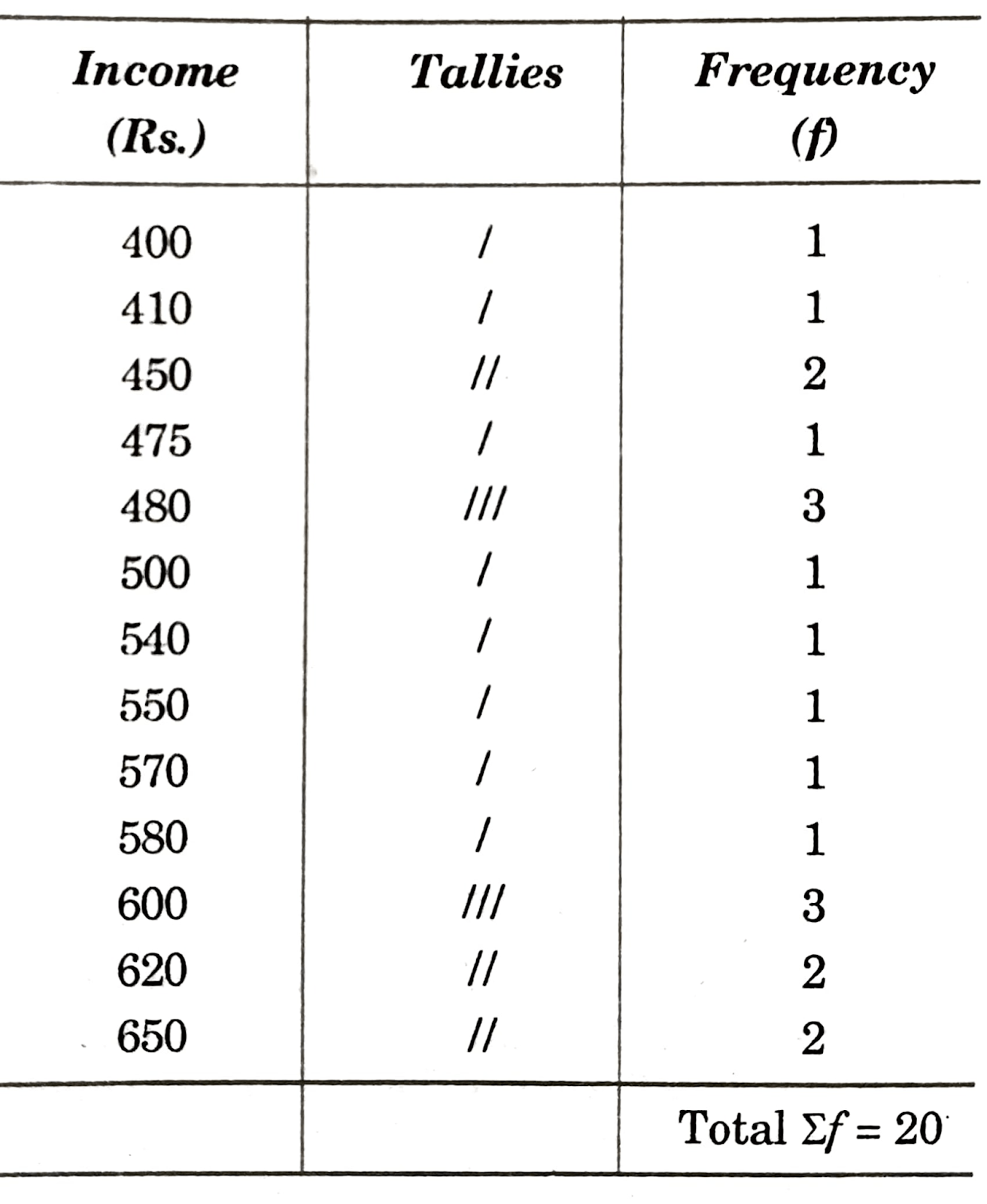
Q.5. Explain the concept of ‘frequency distribution’. How is it different from frequency array’?
Ans. An array is useful where the number of items is small but as the number of items increase, the series became to long and it becomes difficult to manage. Due to this problem, frequency distribution is used to process the data. In other words, the method of arranging the data through frequency array is not suitable, where the series of data is quite big To put the data in a precisely manner, there grouping is must and for this frequency distribution is required.
While constructing frequency distribution all steps remain the same as are taken in case of frequency array. The main and basic difference is this that we make and classify the data into group by forming classes like (10-15), (20-25), etc.
Some of the important concepts of frequency distribution are as follows:
(i) Class: Class is a group of magnitudes having two ends called class limits. For example, 20-25, 25-30, etc. or 20-24, 25-29, etc. as the case may be, each represents a class.
(ii) Class Limits: Every class has two boundaries or limits called lower limit (L₁) and upper limit (L₂). For example in the class (20-30) L₁ = 20 and L₂ = 30.
(iii) Class Interval: The difference of two limits of a class is called class interval. It is equal to upper limit minus lower limit. It is also called class width. Class interval = L₂ – L₁. For example in the class (20-30), class interval = L₂ – L₁ = 30 – 20 = = 10.
(iv) Class Frequency: Total number of items falling in a class that is having the values within L₁ and L₂ is class frequency. For example in table 6.1, class frequency in class (40-45) is 10. Similarly in class (50-55) the frequency is 4.
(v) Mid-Point/Mid-Value (M.V.): The mid-value of the class interval of a class also called as mid-point is obtained by dividing the sum of lower limit and upper limit of the class by 2. It is the average value of two limits of a class. It falls just in the middle of a class that is:
M.V. = L₁ + L₂ / 2
For example, the mid-value of the class
(20-30) is 20 + 30 / 2 = 25.
Q.6. On the basis of data in question 4, prepare a frequency distribution by exclusive method.
Ans. First two steps have already been explained in answer to question 4. The third step is as follows:
| Income group (Rs.) | Frequency (f) |
| 400 – 450 | 2 |
| 450 – 500 | 6 |
| 500 – 550 | 2 |
| 550 – 600 | 3 |
| 600 – 650 | 5 |
| 650 – 700 | 2 |
| Σf = 20 |
Q.7. Distinguish between ‘exclusive method’ and ‘inclusive method’ of frequency distribution with examples.
Ans. Exclusive method: Under this method upper limits are excluded. The upper limit of class interval is the lower limit of the next class. For example, if the students obtained marks are grouped as 5-10, 10-15, 15-20, 20-25, 25-30, etc. we include in first group the students whose marks are 5 or more but under 10. If the marks of a student is 10 he is not included in the first group but in the second, i.e. 10 to 15.
Inclusive method: Under this method upper class limits of classes are included in respective classes. For example, if the students obtained marks are grouped as 5-9, 10-14, 15-19, 20-24, 25-29, etc. in the group 5-9, we include in the first group of students whose marks is between 5-9. If the marks of students are 10 he is included in the next class i.e. 10 to 14.
Q.8. Write short notes on:
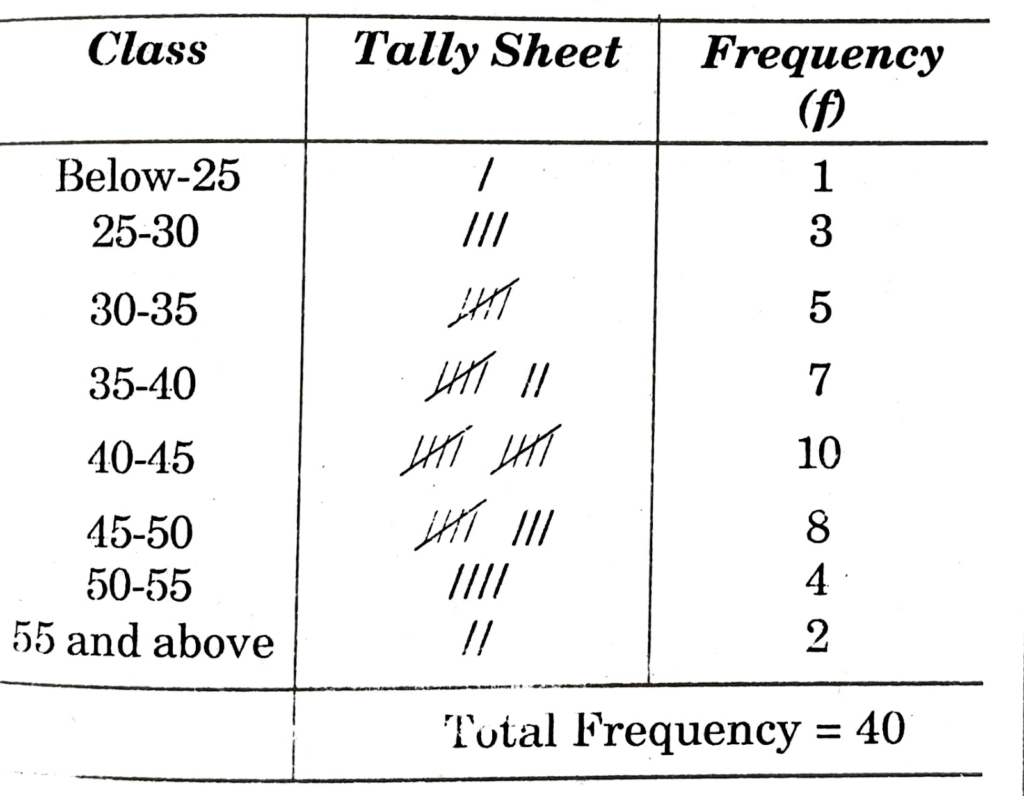
(a) Open-end frequency distribution.
Ans. Open-end frequency distribution: Open-end frequency distribution is one which has at least one of its ends open. It can be observed that either lower limit of first class or upper limit of last class or both are not given in such series. In table the first class and the last class, i.e., below 25 and 55 and above are open-end classes.
Table: Open-end Frequency Distribution
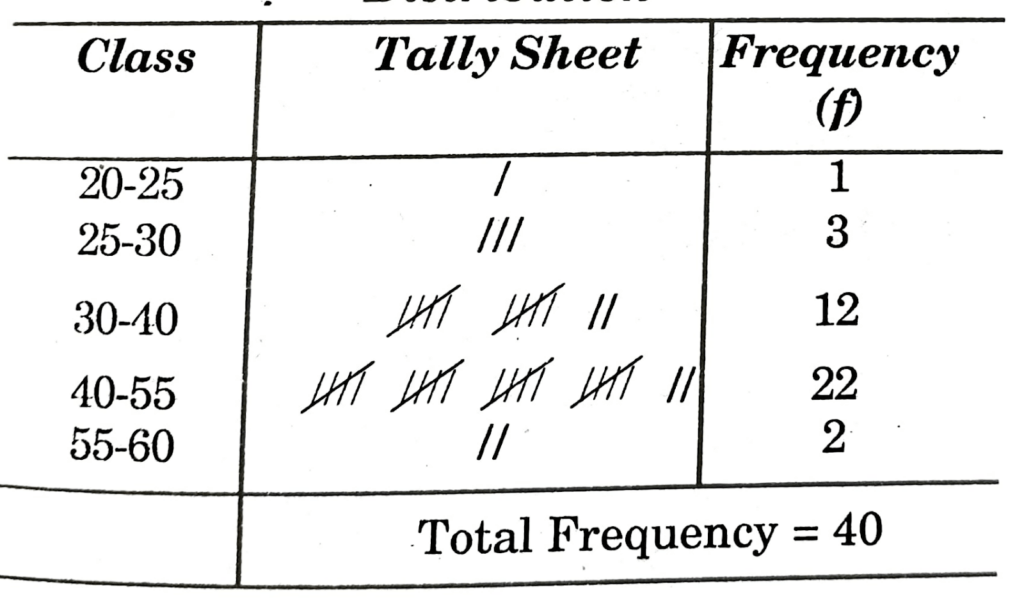
(b) Frequency distribution with unequal classes.
Ans. Frequency distribution with unequal classes: In case of unequal classes frequency distribution, the width of different classes (i.e., L₂ – L₁) need not be the same. In table, the class (30-40) has width 10 while the class (40-55) has width 15.
Table: Unequal Classes Frequency Distribution
(c) Cumulative frequency distribution.
Ans. Cumulative frequency distribution: A ‘cumulative frequency distribution’ is formed by taking successive totals of given frequencies. Such totalling can be done in two ways:
(i) From above, such as 1, 4 (i.e., 1 + 3), 9 (i.e. 4 + 5), 16 (i.e. 9 + 7), and so on. Such a distribution is called ‘Less-than’ cumulative frequency distribution. It shows the total numbers of observations (frequencies) having less than a particular value of the variable (here marks). For example, there are 4 (i.e. 1 + 3) students who got marks less than 30; 9 (i.e. 4+5) students who got marks less than 35 etc. Table gives the less-than cumulative frequency distribution.
Table: ‘Less-than’ Cumulative Frequency Distribution
| Marks | Cumulative Frequency |
| Less than 25 | 1 |
| Less than 30 | 4 (1+3) |
| Less than 35 | 9 (4+5) |
| Less than 40 | 16 (9+7) |
| Less than 45 | 26 (16+10) |
| Less than 50 | 34 (26+8) |
| Less than 55 | 38 (34+4) |
| Less than 60 | 40 (38+2) |
(ii) From below, such as 2, 6 (i.e., 2+4), 14 (i.e., 6 + 8), 24 (i.e., 14+ 10) and so on. Such a distribution is called ‘More-than’ cumulative frequency distribution. It shows the total number of observations (frequencies) having more than a particular value of the variable (here marks). For example there are 6 (i.e. 2 + 4) students who got marks more than 50, 14 (i.e. 2 + 4 + 8) students who got marks more than 45 etc. Table shows the more than cumulative frequency distribution.
Table: ‘More-than’ Cumulative Frequency distribution
| Marks | Cumulative Frequency |
| More than 20 | 40 |
| More than 25 | 39 (40-1) |
| More than 30 | 36 (39-3) |
| More than 35 | 31 (36-5) |
| More than 40 | 24 (31-7) |
| More than 45 | 14 (24-10) |
| More than 50 | 6 (14-8) |
| More than 55 | 2 (6-4) |

Hi! my Name is Parimal Roy. I have completed my Bachelor’s degree in Philosophy (B.A.) from Silapathar General College. Currently, I am working as an HR Manager at Dev Library. It is a website that provides study materials for students from Class 3 to 12, including SCERT and NCERT notes. It also offers resources for BA, B.Com, B.Sc, and Computer Science, along with postgraduate notes. Besides study materials, the website has novels, eBooks, health and finance articles, biographies, quotes, and more.



