Class 11 Economics Chapter 12 Measures of Dispersion, (Assam Higher Secondary Education Council) AHSEC Class 11 Economics Question Answer in English Medium each chapter is provided in the list of AHSEC so that you can easily browse through different chapters and select needs one. Assam Board Chapter 12 Measures of Dispersion Class 11 Economics Question Answer can be of great value to excel in the examination.
AHSEC Class 11 Economics Chapter 12 Measures of Dispersion
Class 11 Economics Chapter 12 Measures of Dispersion Notes cover all the exercise questions in SCERT Textbooks. The SCERT Class 11 Economics Chapter 12 Measures of Dispersion Solutions provided here ensure a smooth and easy understanding of all the concepts. Understand the concepts behind every chapter and score well in the board exams.
Measures of Dispersion
Chapter – 12
PART – B
VERY SHORT ANSWER QUESTIONS
1. What is meant by dispersion ?
Ans: It is the amount of degree of variations of the items around some average or among themselves.
2. What is absolute measure of dispersion ?
Ans: It is expressed in the same statistical unit in which the data is given.
3. What is relative measure of dispersion ?
Ans: These are expressed in pure numbers and are independent of the unit in which data is given.
4. Define Range.
Ans: It is the difference between the values of the largest and smallest item of a series.
5. Define quartile deviation.
Ans: It is the average of the difference between the two quartile.
6. Define Mean Deviation.
Ans: It is the average difference between the item in a series measured from its central tendency.
7. Define standard Deviation.
Ans: It is the square root of arithmetic mean of the squares of deviation of items from their mean.
8. What is meant by variance ?
Ans: It is the square of standard deviation.
9. Define Lorenz Curve.
Ans: The Lorenz Curve was given by Max O. Lorenz who was a popular economic statistician. It is graphic method of studying dispersion.
B. SHORT & LONG TYPES ANSWER QUESTIONS :
1. What is the relationship between quartile deviation (Q. D.) and standard deviation (S. D.)?
Ans: σ(S.D)=3/2Q.D
⇒Q.D. =2/3σ
2. What is the relationship between S. D. and M. D.? (MeanDeviation)
Ans: M.D=4/5σ
σ=5/4MD
3. Mention any one use of Lorenz Curve.
Ans: To compare the inequalities in the distribution of income and wealth.
4. Name the methods to measure dispersion.
Ans: The name of the methods to measure dispersion :
(a) Range
(b) Quartile deviation
(c) Mean deviation
(d) Standard deviation.
5. Write the objectives of dispersion.
Ans: The objectives of dispersion are:
(a) To determine the reliability of an average.
(b) Control over variability.
(c) Comparison of series.
6. Write the properties of a good measure of dispersion.
Ans: The properties of a good measure of dispersion are:
(a) Simple to understand.
(b) Easy to calculate.
(c) Should be rigidly distribution.
(d) Based on all items of distribution.
(e) Suitable for further algebraic treatment.
7. Mention the formulas for measuring coefficient of range, quartile deviation, mean deviation, standard deviation and co efficient of variation.
Ans: Co-efficient of Range = L-S/L+S
Coefficient of quartile deviation
= Q₃ -Q₁/Q₃+Q₁
Coefficient of standard Deviation =σ≡X
Coefficient of Variation =σ≡x×100
8. Explain the concept of coefficient of variation.
Ans: It is the percentage variation in mean, standard deviation being considered as the total variation in the mean. It is a relative measure of dispersion developed by Karl Pearson. It is used in such problems where we want to compare the variability of two or more than two series. Higher coefficient of variation means lower consistency and lower coefficient of variation means higher consistency. It is calculated is the following
manner – C.V.= σ≡x100
9. Write the merits and demerits of range.
Ans: Merits:
(i) It is very simple measure of dispersion.
(ii) It is used in calculating variation in stock and gold prices.
(iii) It is used as a quality control measure.
Demerits:
(i) It is not based on all items of a series.
(ii) In case of open and classes, it is difficult to determine.
10. Write the merits and demerits of mean deviation.
Ans: Merits:
(a) It is based upon each and every item of a series.
(b) It is less affected by extreme items of the series.
(c) It is relatively simple to understand and calculate.
Demerits:
(a) It ignores plus minus signs in deviation.
(b) When it is calculated from mode, it can’t be reliable because mode becomes indeterminate in some cases.
11. Write the merits and demerits of standard deviation.
Ans: Merits:
(a) In the normal distribution, standard deviation has played a central role.
(b) It is best measure of dispersion, because the sum of squares of deviation from the actual mean is the minimum.
(c) It has the merit of further algebraic treatment.
Demerits:
(a) It can’t be used for comparison of two phenomenon.
(b) The extreme items gain great importance.
12. Write the merits and demerits of quartile deviations.
Ans: Merits:
(a) It is easy to calculate, It is based on two values only ie. Q₁ and Q₃.
(b) It is mot affected by the extreme items.
(c) In open end distributions, quartile deviation is most suitable.
Demerits:
(a) It is not suited to algebraic treatment or manipulation as it ignores the first and last 25% items on both sides.
(b) It is very much effected by sampling fluctuations.
13. Write the formulas for range, inter quartile range, quartile deviations, mean deviations, standard deviations for individual series.
Ans: The formulas are listed below –
(a) Range
Where
(R)=L-S
L = largest value
S= Smallest value
(b) Inter Quartile Range
= Q₃-Q₁
(c) Quartile deviation (Q D)
=Q₃-Q₁/2
(d) Mean deviation (MD)
=MD/ χ or Md M₀
(e) Standard Deviation σ :
(i) Direst Method:
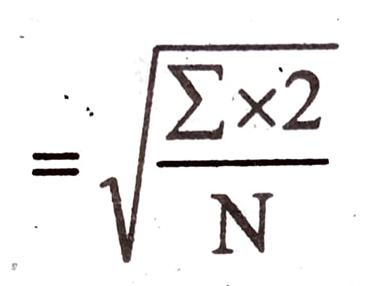
(ii) Short cut Method:
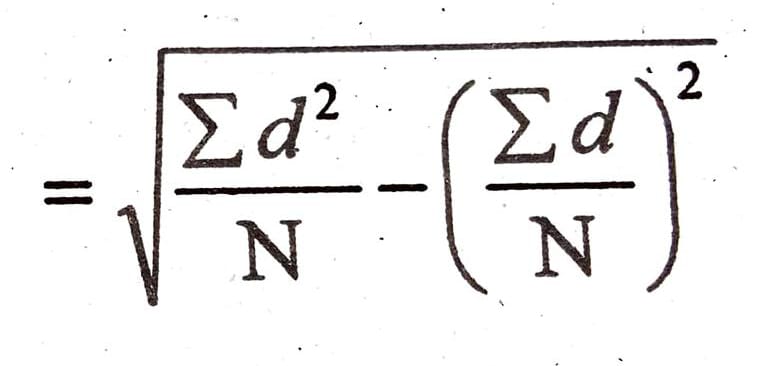
(ii) Step deviation Method:
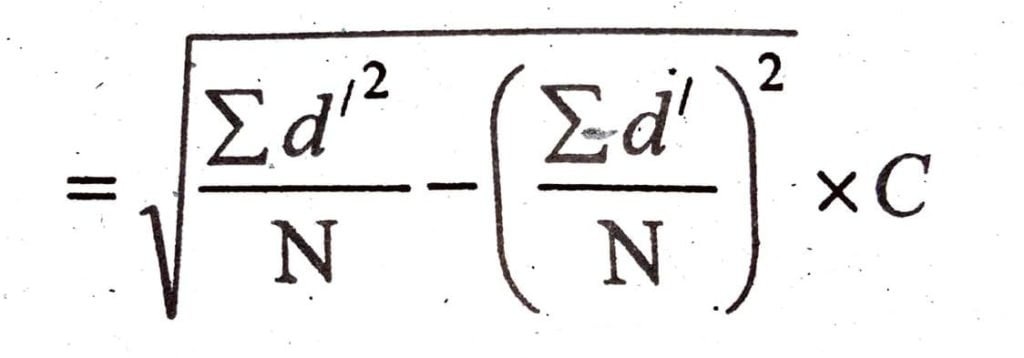
14. Write the formulas of range, inter quartile range, quartile deviation, mean deviation, standard deviation for continuous series.
Ans: The formulas are listed below:
(a) Range (R) = L-S
(b) Inter Quartile Range
= Q₃-Q₁
(c) Quartile deviation (Q D)
=Q₃-Q₁/2
(d)Mean deviation (MD)
=MD/ χ or Md M₀
(e) Standard Deviation σ :
(i) Direst Method:
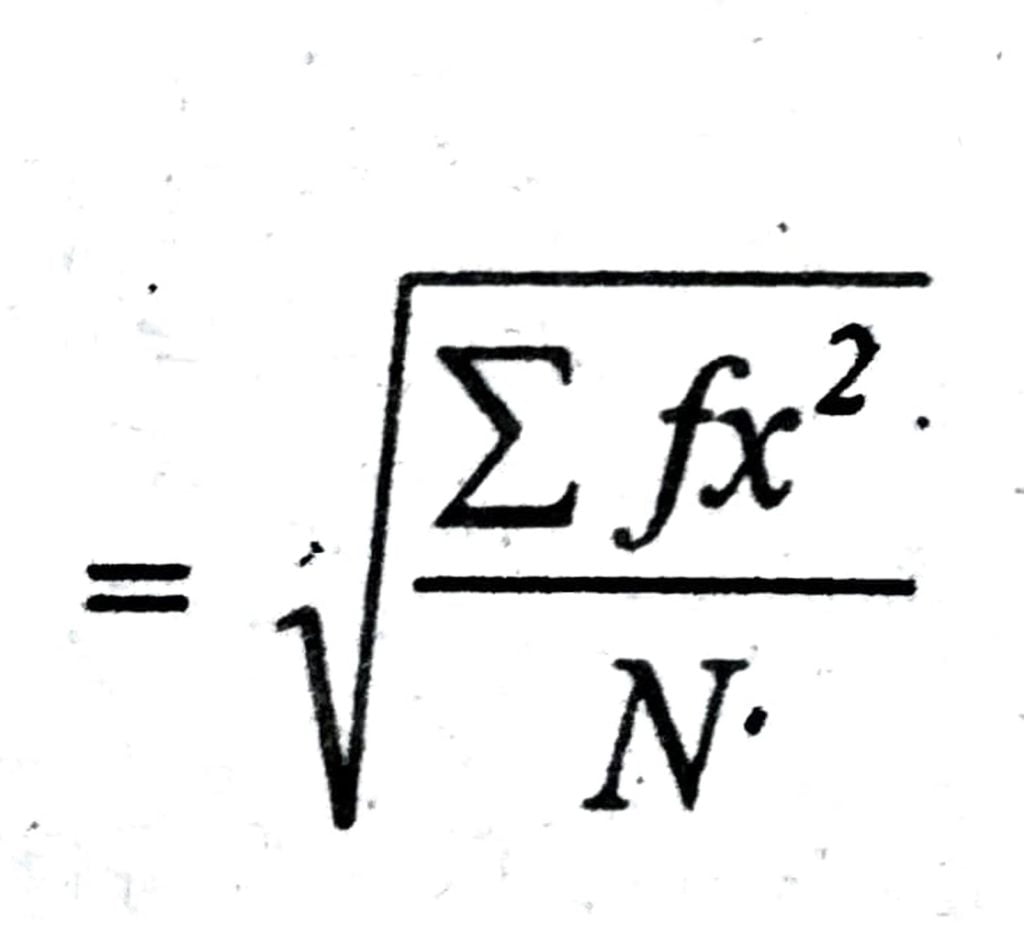
(ii) Short cut Method:
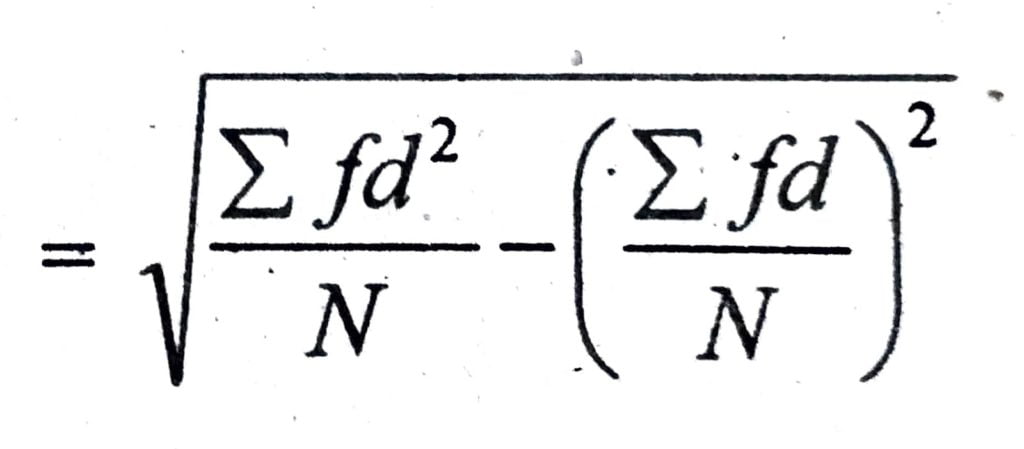
(ii) Step deviation Method:
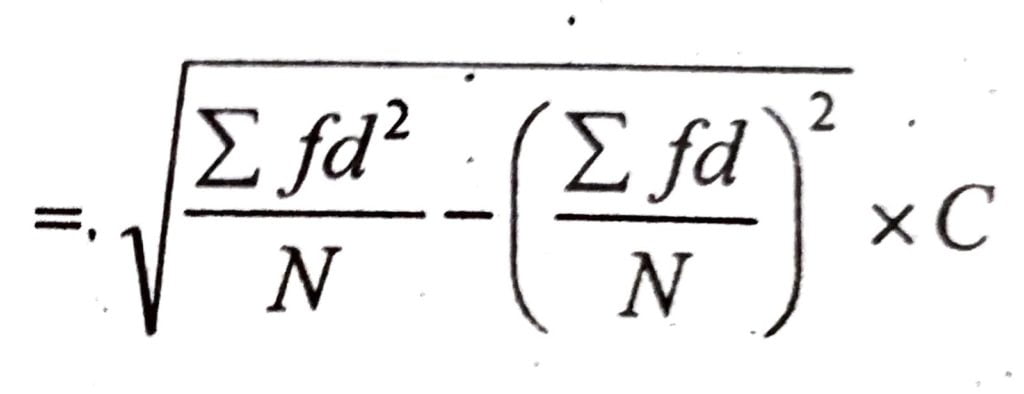
15. Which measure of dispersion is the best and why ?
Ans: Standard deviation is considered as the best measure the reasons are-
(a) It is based upon all the values of the series.
(b) It gives due regard to the deviations and their signs.
(c) It is independent of origin but not of scale,
(d) It is useful in advanced statistical problems.
16. Some measures of dispersion depend upon the spread if values, Where as some calculate the variation of values from a central value. Do you agree ?
Ans: Yes, measures of dispersion like range and quartile devotion are based upon spread of values. In range pure spread (largest-smallest values) is studied where as in quartile deviation consider 75% (Q3) and 25% (Q1) of value in a series. However, mean deviation and standard deviation are used with the help of central values like mean, median or mode. Standard deviation is calculated only with the help of arithmetic means. It is considered as the best of all measures.
17. A measure of dispersion is a good supplement to the central value in understanding a frequency distribution. Comment.
Ans: Central values of the series mean, median, mode provide central position of the series. It does not explain the amount of scatteredness of the series. The measure of central value can’t be a true representative of the series without the analysis of measures of dispersion. Dispersion minutely studies the spread or variation in a series. Therefore, it supplements the study made by central value for a series. An example would clarify it in more details-
Following data relates to salary of 5 warders in three towns leaving same
arithmetic mean:
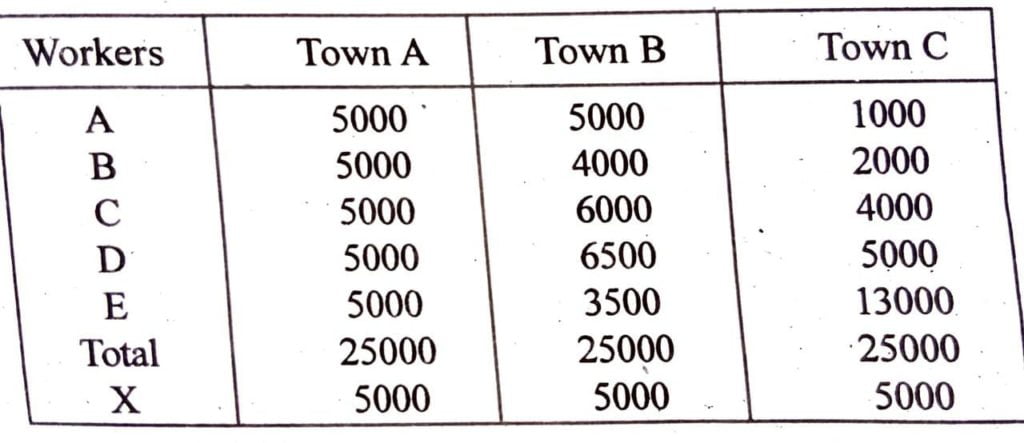
Here central value (AM) is Rs. 5000 for each worker, but it is not the true representative in case of C. It means, the analysis of central value must be supplemented with measure of dispersion.
18. In a town 25% of persons earned more than Rs. 45000 where 75% earned more than Rs 18000. Calculate the absolute out relative values of dispersion.
Ans: Here,
Q₁ = Rs. 18000
Q₂ = Rs. 45000
Now Quartile Deviation (Absolute Measure).
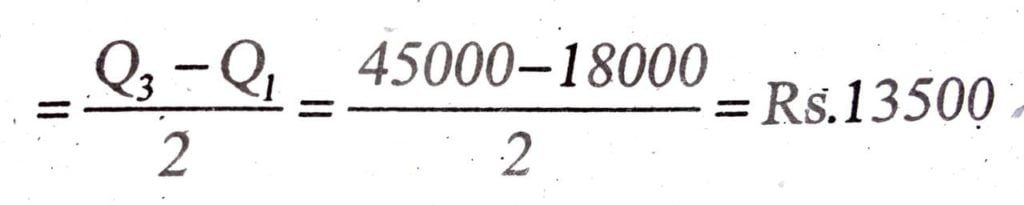
Again Coefficient of quartile Deviation (Relative Measure)
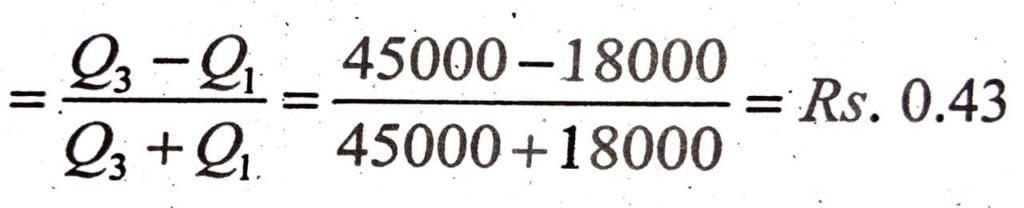
19. The yield of wheat and rice per acre for 10 distinct of state is as under-
| District: | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| Wheat: | 12 | 10 | 15 | 19 | 21 | 16 | 18 | 9 | 15 | 10 |
| Rice: | 22 | 29 | 12 | 23 | 18 | 15 | 12 | 34 | 18 | 12 |
Calculate for each crop-
(i) Range
(ii) Q. D
(iii) Mean Deviation about Mean
(iv) Mean Deviation about Median.
(v) Standard Deviation.
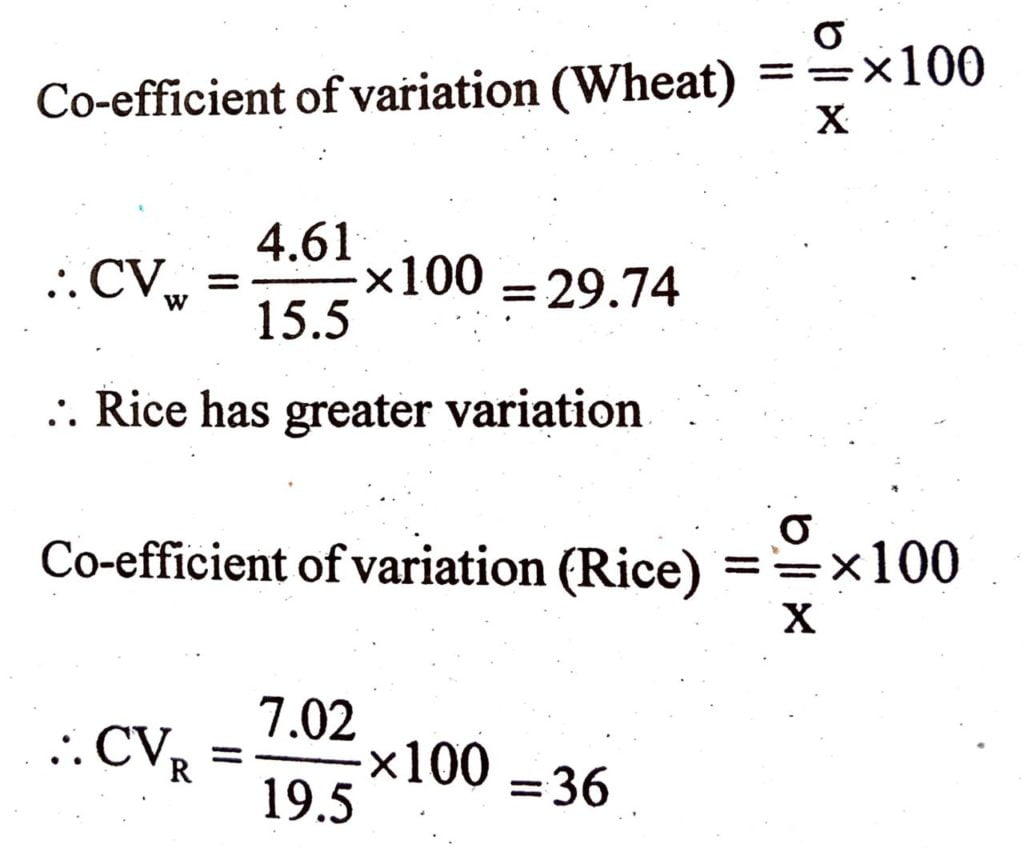
(vi) Which crop has greater variation ?
(vii) Compare the values of different measures for each crop.
Ans: (i) Range (for wheat)
= Largest Value – Smallest Value
= 25-9
= 16
Range (for Rice)
=Largest Value – Smallest Value
=34-12-22
(ii) QD = Q₃-Q₁/2
Now, Q(for Wheat) =
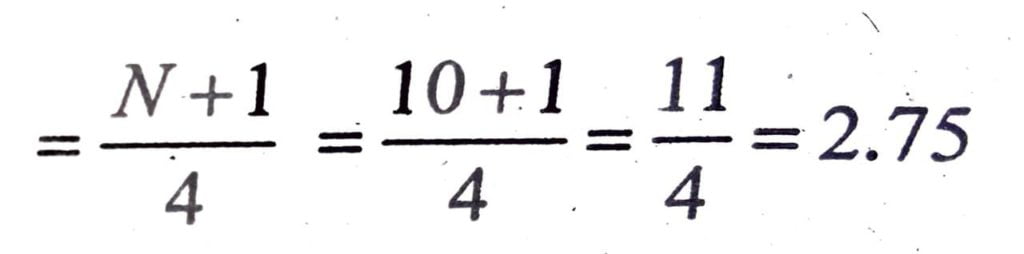
∴ 10+0.75 (10-10)-10
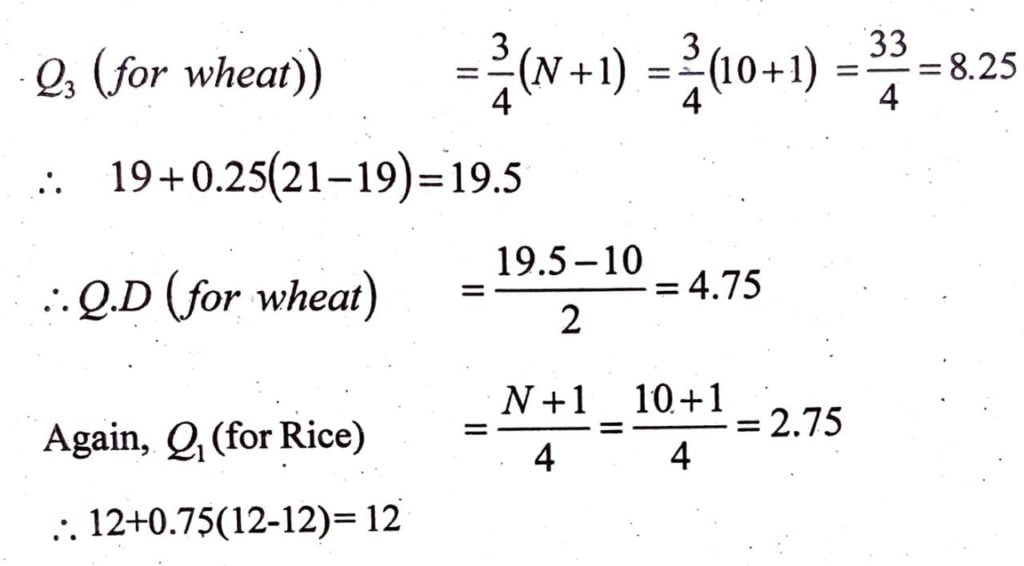
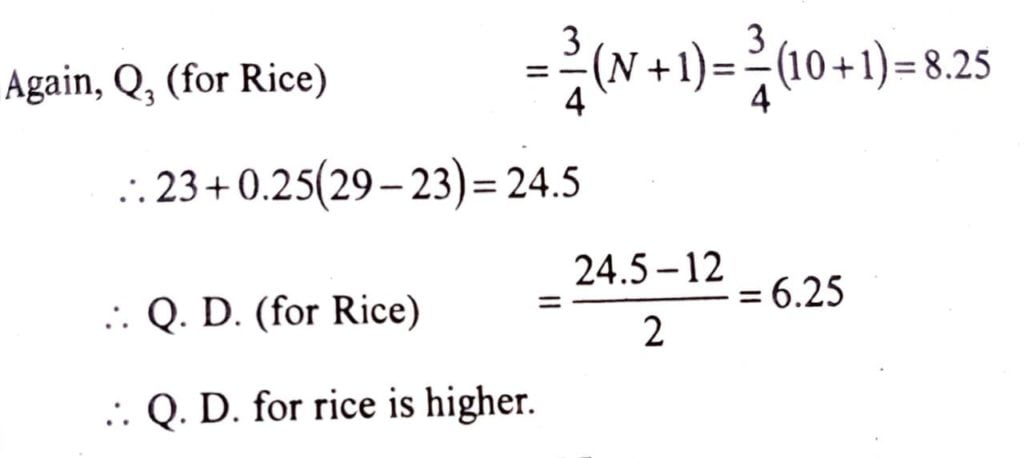
(iii) Mean Deviation about Mean
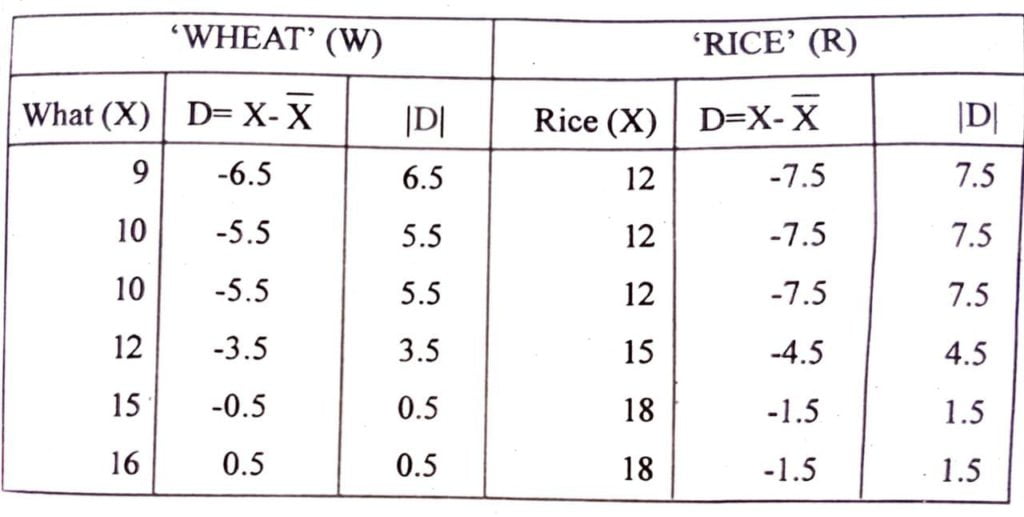
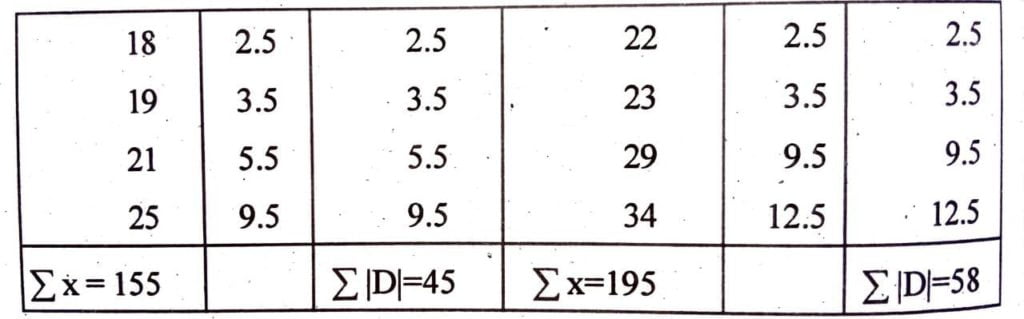
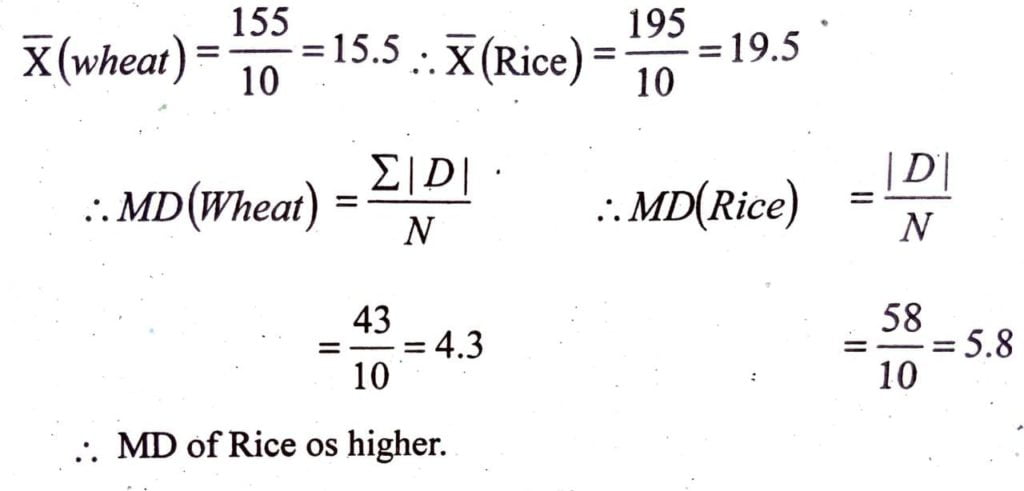
(iv) Mean Deviation about Median.
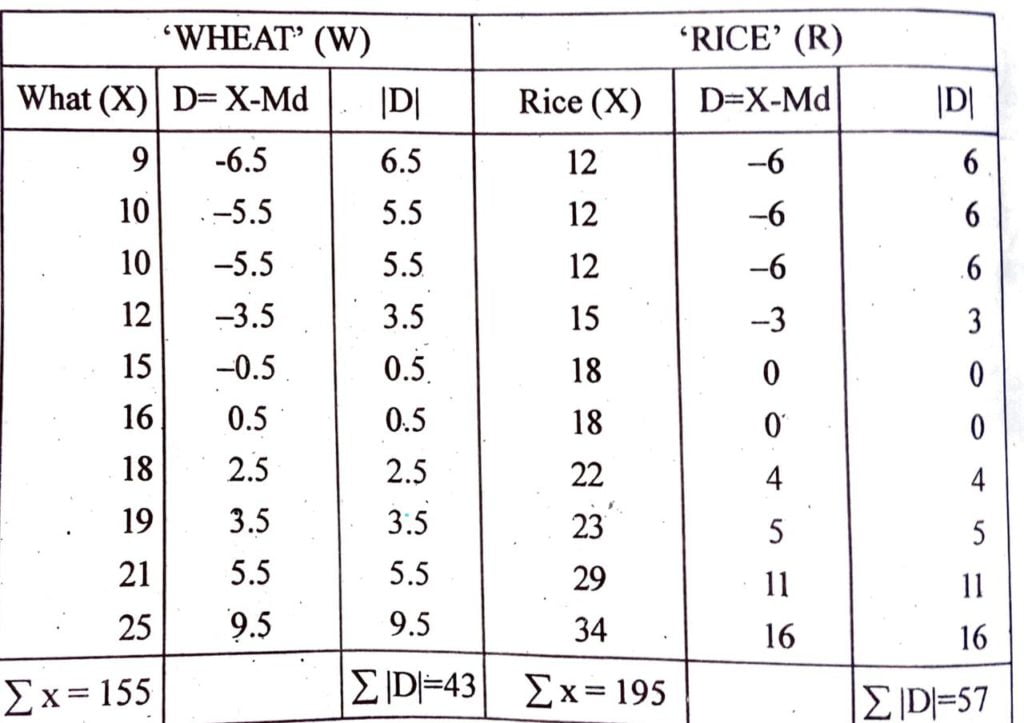
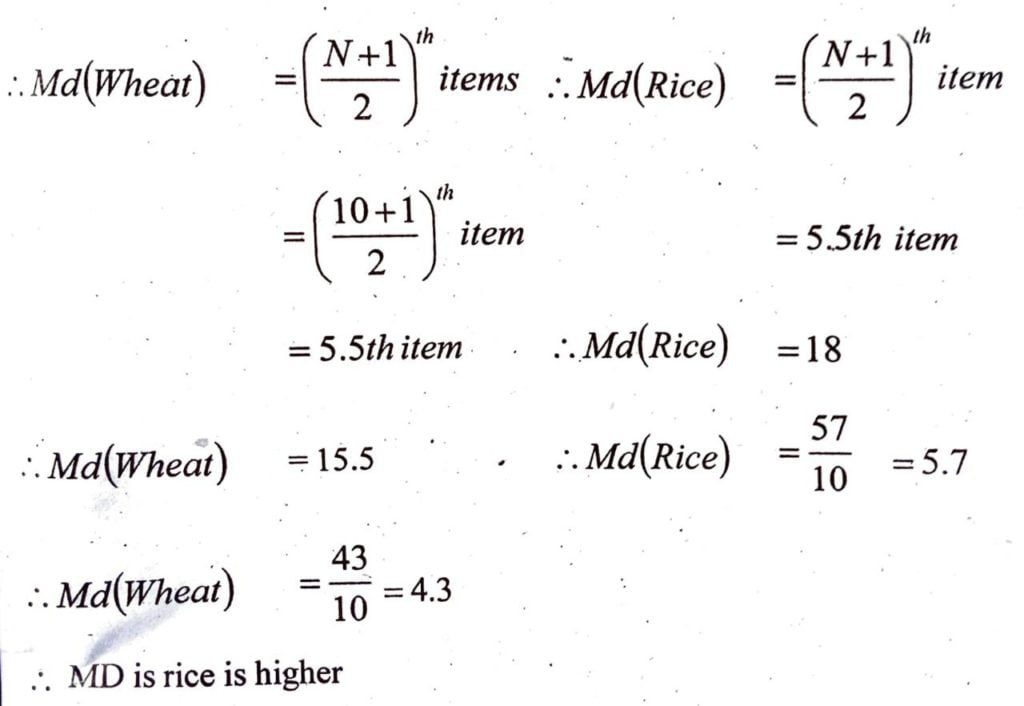
(v) Standard Deviation.
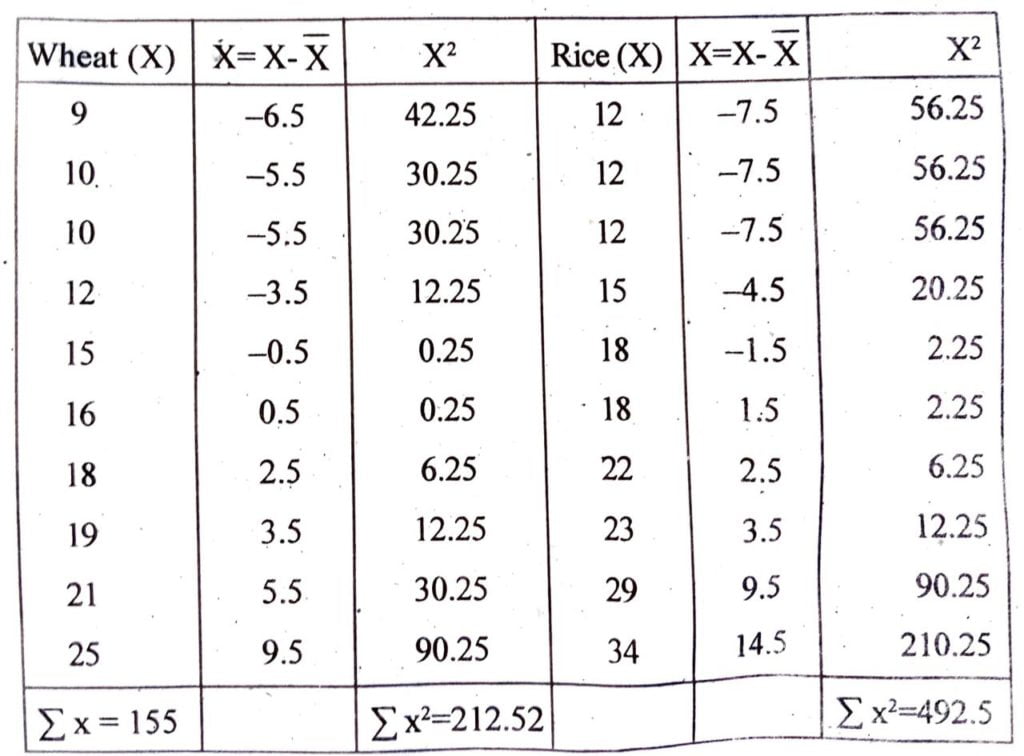
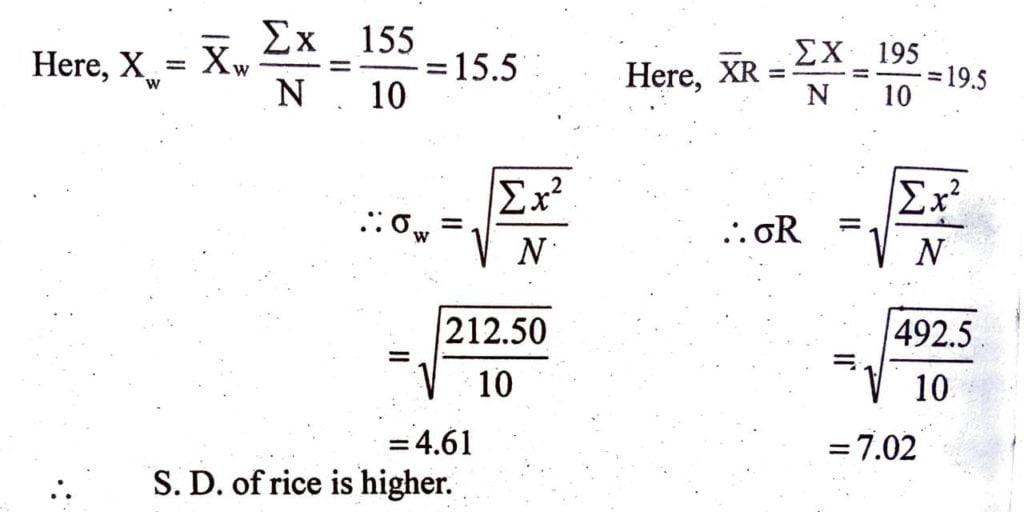
(vi) Which crop has greater variation
(vii) Compare the values of different measures for each crop.
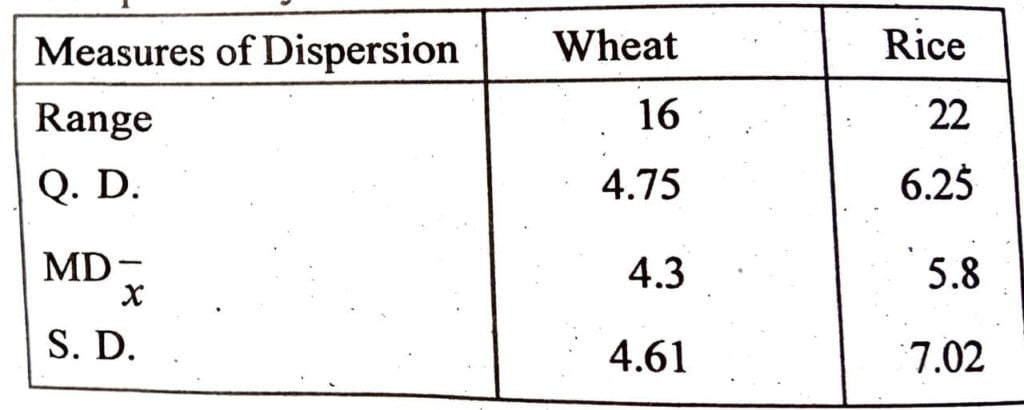
From this overall analysis we find that variation in Rice comparatively greater from all the measures.
20. To check the quality of two brands of light bulbs, their life in burning hours was estimated as under for 100 bulbs of each brand-
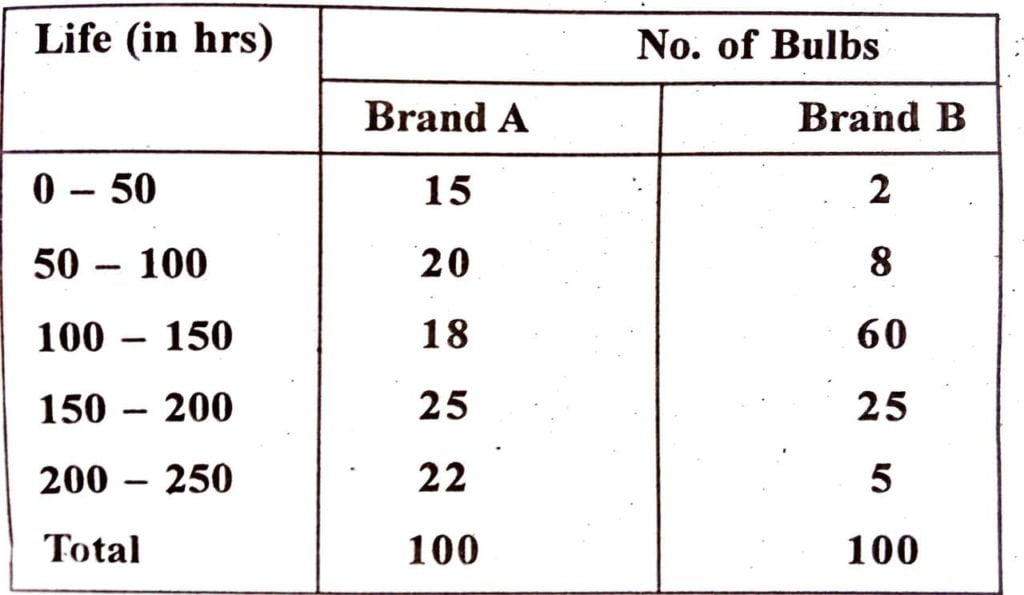
Ans: To Check higher life, we calculate mean and dependability is calculated on the basis of coefficient of variation.
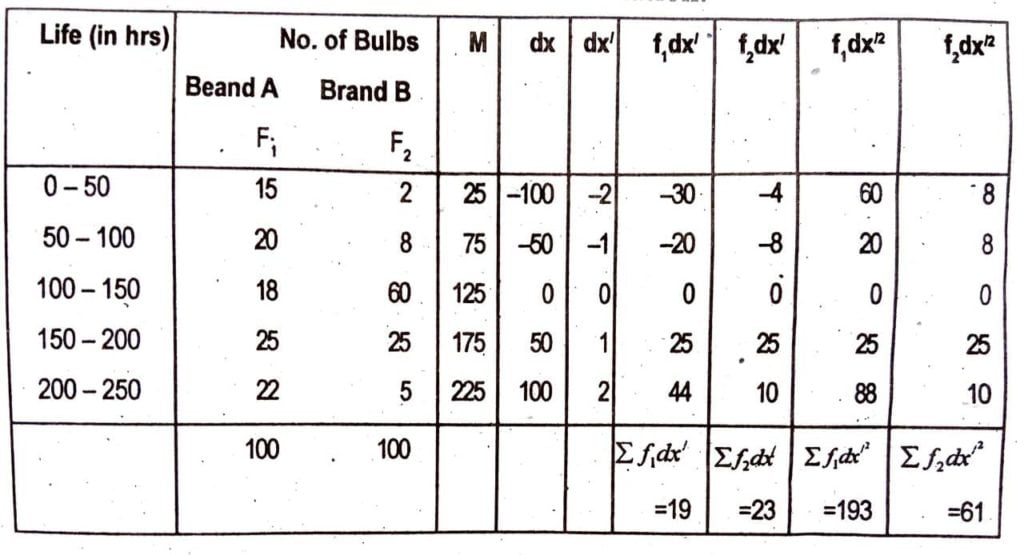
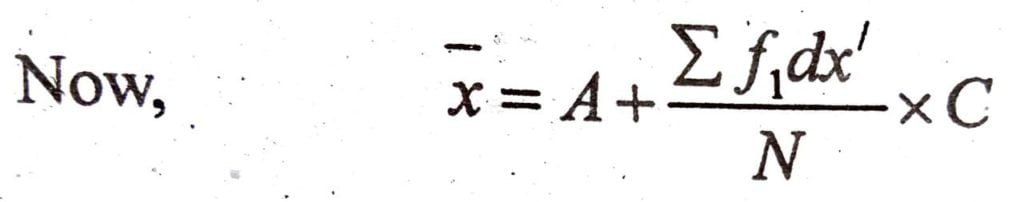
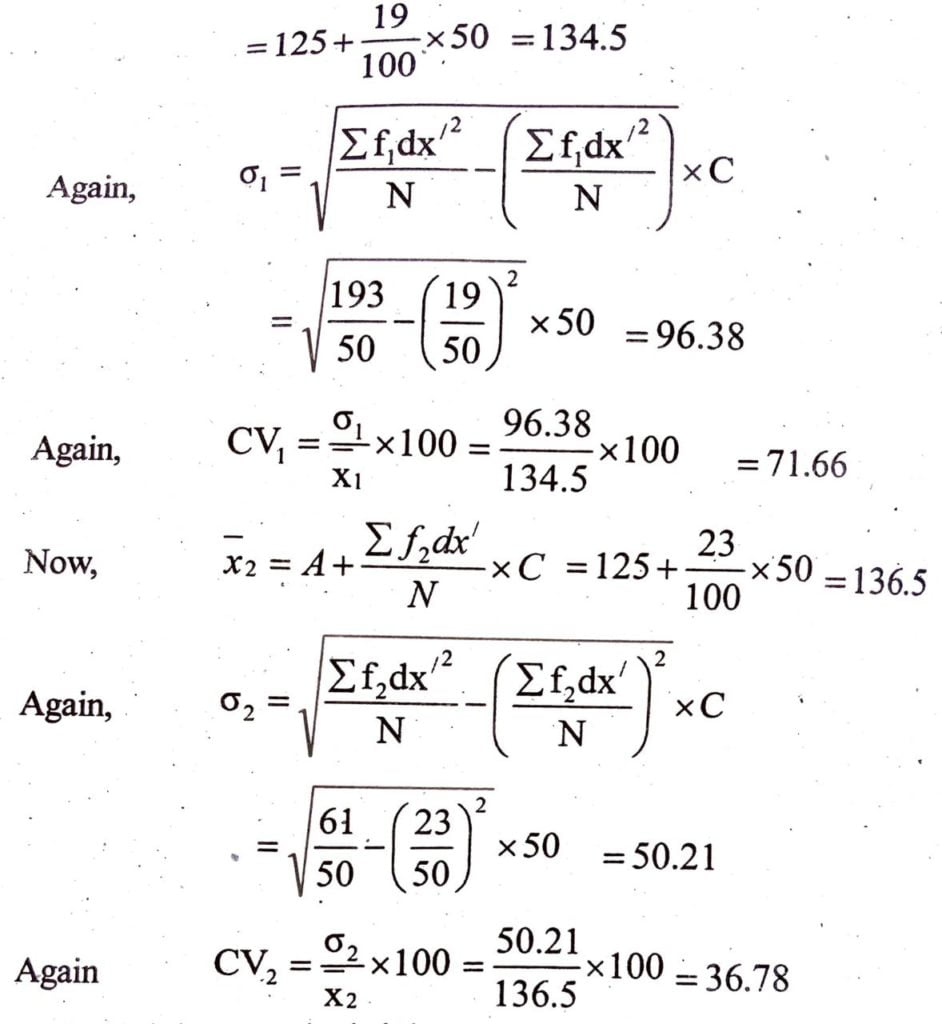
From, the above analysis it is seen that –
Brand B has higher mean life (ie, x2 = 136.5)
∴ It is better..
Again,
Brand B has low CV (ic, CV₂ = 36.78)
∴ It is also consistent
21. Average daily wage of 50 workers of a factory was Rs 200 with a S. D. of Rs. 40. Each worker is given a raise of what is the new daily wage and standard deviation Have wages become more and less uniform ?
Ans: Given, x=200
∴ Wage of each worker is raised with Rs 20
New Wage (average) will became = Rs 200+ Rs 20 = Rs 220 However, there will be no change upon S. D. It remains constant as 20. Again,
To know uniformity we calculate CV before and after the increase of wages.
Before
CV₁ = 40/200× 100=20
After
CV₁ = 40/200× 100=16.67
∴ CV, has fallen
∴ The wages have become more uniform.
22. The sum of 10 values is 100 and sum of their squa.es is 1090. Find out its coefficient of variation.
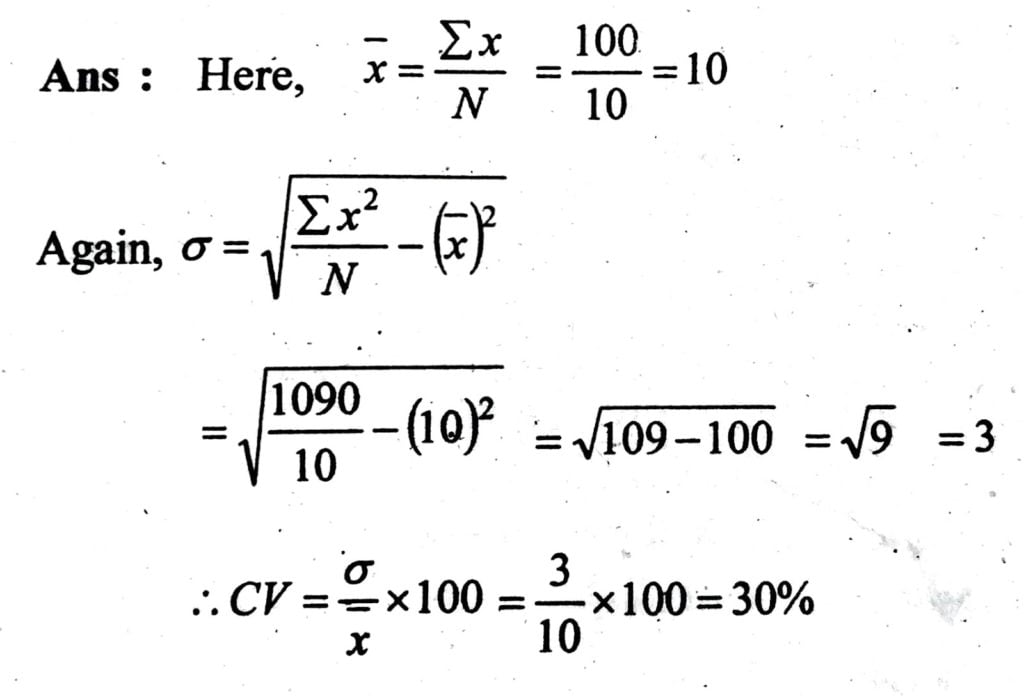

Hi, I’m Dev Kirtonia, Founder & CEO of Dev Library. A website that provides all SCERT, NCERT 3 to 12, and BA, B.com, B.Sc, and Computer Science with Post Graduate Notes & Suggestions, Novel, eBooks, Biography, Quotes, Study Materials, and more.



