Class 11 Economics Chapter 11 Measures of Central Tendency, (Assam Higher Secondary Education Council) AHSEC Class 11 Economics Question Answer in English Medium each chapter is provided in the list of AHSEC so that you can easily browse through different chapters and select needs one. Assam Board Chapter 11 Measures of Central Tendency Class 11 Economics Question Answer can be of great value to excel in the examination.
AHSEC Class 11 Economics Chapter 11 Measures of Central Tendency
Class 11 Economics Chapter 11 Measures of Central Tendency Notes cover all the exercise questions in SCERT Textbooks. The SCERT Class 11 Economics Chapter 11 Measures of Central Tendency Solutions provided here ensure a smooth and easy understanding of all the concepts. Understand the concepts behind every chapter and score well in the board exams.
Measures of Central Tendency
Chapter – 11
PART – B
VERY SHORT ANSWER QUESTIONS
1. Which average would be most suitable in following cases ?
(i) Average size of readymade garments.
Ans: Mode.
(ii) Average intelligence of students in a class.
Ans: Median.
(iii) Average production in a factory per shift.
Ans: Mean.
(iv) Average wages in an industrial concern.
Ans: Mean.
(v) When the sum of absolute deviations from average is least ?
Ans: When deviation are taken from mean.
(vi) When Quantities of the variable are in ratios.
Ans: Geometric Mean.
(vii) In case of open ended frequency distribution.
Ans: Median.
2. Indicate the most appropriate alternative from the multiple choices provided against each question.
(i) The most suitable average for qualitative measurement is-
(a) Arithmetic Mean.
(b) Median.
(c) Mode.
(d) Geometric mean. none of the above.
(e) none of the above.
Ans: (b) Median.
(ii) Which average is affected most by the presence of extreme items ?
(a) median.
(b) mode.
(c) arithmetic mean.
(d) geometric mean.
(e) harmonic mean.
Ans: (c) arithmetic mean.
(iii) The algebraic sum of deviation of a set of a values from A. M. is
(a) n
(b) 0
(c) 1
(d) none of the above.
Ans: (b) 0 (Zero)
3. Comment the following statement are true or false ?
(i) The deviations of items from median is Zero.
Ans: False.
(ii) An average alone is not enough to compare series.
Ans: True.
(iii) Arithmetic mean is a positional average
Ans: False
(iv) Upper quartile is the lowest value of top 25% items.
Ans: True.
(v) Median is unduly affected by extreme observations.
Ans: False
4. Define central tendency.
Ans: Any statistical measure which gives an idea about the position or the point around which other observations cluster io called measures of central tendency. It to a central value around which all other values revolve.
5. What is a statistical average ?
Ans: It is a statistical device which condense a huge unwieldy set of data into a single numerical value which represent the whole group.
6. What are the kinds of statistical averages ?
Ans: The kinds of statistical averages are:
(a) Mathematical averages, like, arithmetic mean, geometric
(b) Positional averages, like, median, mode etc.
(c) Commercial averages, like, moving averages etc.
7. Define arithmetic mean.
Ans: It is a common average. It is a measure of central value and provides a single representative figure to the whole data by taking into consideration all the items.
8. Mention the formula for combined mean.
Ans:
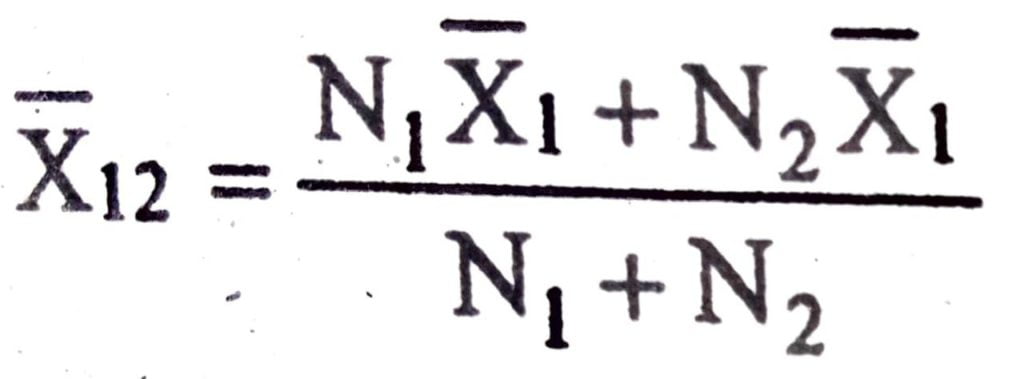
9. Mention the formula of weighted arithmetic mean.
Ans:
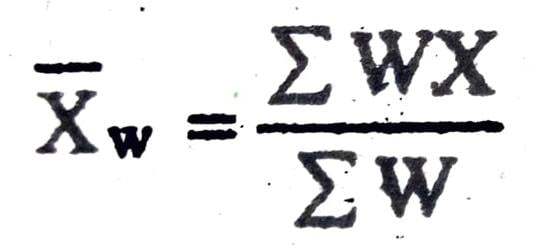
10. Define median.
Ans: It refers to the middle value of the distribution, when the distribution is arranged either in ascending or descending order.
11. What is a positional average ?
Ans: An average is known as positional average whose value is affected by the position of certain items in the distributions.
12. How median is located graphically
Ans: By constructing ogive.
13. Define mode.
Ans: Mode is distribution and is the value around which most value heavily concentrates. It is the most repeated value of the series.
14. Mention the graph for calculating mode.
Ans: Histogram.
15. Mention the formula for calculating mode from mean an median.
Ans: Z=3 Median-2 Mean.
SHORT & LONG TYPES ANSWER QUESTIONS:
1. Write the advantages of Arithmetic Mean.
Ans: (a) It is simple to understand, easy to calculate and can be determined in most of the cases.
(b) Of all the types of averages, it is readily understood even by person of mediocre intelligence.
(c) It can further be treated mathematically and as such helps in further analysis and interpretation of data.
(d) It is based upon all the observations.
(e) It satisfies most of the conditions laid down for an ideal average.
2. Write the disadvantages of Arithmetic Mean.
Ans: The disadvantages of Arithmetic Mean are:
(a) It can be calculated only if all the frequencies and the exact size of all amounts are known. Even if in single item is missing out of a series, average can’t be accurately determined.
(b) It is affected by the value of extreme items. Any abnormal variation in the form of a single item can make the mean unrepresentative.
(c) In the absence of actual figures, it may lead to wrong conclusions.
(d) It can’t be used in qualitative data as it is not subject to numerical expression.
3. What are the essential features of an average ?
Ans: Essential features of an average are-
(a) It should be rigidly defined.
(b)It should be based on all the observations.
(c) It should be easy to understand.
(d) It should be easy to compute.
(e) It should be capable of further algebraic treatment.
4. Explain the main properties of an average.
Ans: The properties are:
The sum of deviation from actual mean are always zero. It can be should as,
Σ(X-X̅₁)=0
(b) The sum of squared deviations from arithmetic average is always i.e,
Σ(X-X̅)² is always the best.
(c) If two mean and their numbers are given, then, Combined mean can be calculated as-
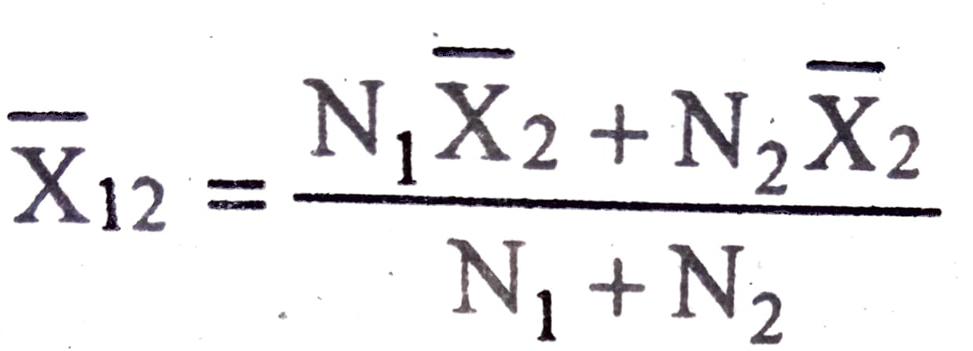
(d) If we know two values out of N,Σx and, X̅ we can calculate the third value using the following formula-
X̅=Ex/N and N.X̅=EX
5. Write the advantages of median.
Ans: Following are the merits:
(a) Like an ideal average, median is also rigidly defined.
(b) It can be understood and calculated easily.
(c) It can be located graphically with the help of ogive
(d) It can be calculated even if the value of the extremes is not known.
6. Write the disadvantages of median.
Ans: The disadvantages of median are:
(a) Median is not suitable for further algebraic treatment.
(b) In many cases, median may not be representative of a series. It happens when there is big variations between the values of different items.
(c) Median is more likely to be affected by fluctuations of sampling than the arithmetic average.
(d) Median ignores the valued of extreme items.
7. Write the merits of mode.
Ans: The merits of mode are:
(a) It is easy to calculate and understand. In many cases, it can be located merely by inspection.
(b) It can be determined by graphic method.
(c) It is not affected by extreme items.
(d) It can be calculated in case of open end classes.
8. Write the demerits of mode.
Ans: The demerits of mode are:
(a) It is not capable of algebraic treatment.
(b) It is not rigidly defined. We may come across distributions with more than two modes.
(c) It is not based on all items in the series.
(d) As compared the other averages mode is affected to a large extent by fluctuations of sampling.
9. If the arithmetic mean of the given below is 28, find
(a) The missing frequency
(b) the median of the series.
| Profit per retail shop (in Rs.): | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 |
| Number of retail shop: | 12 | 18 | 27 | – | 17 | 6 |
Ans: For calculation we make the following table-
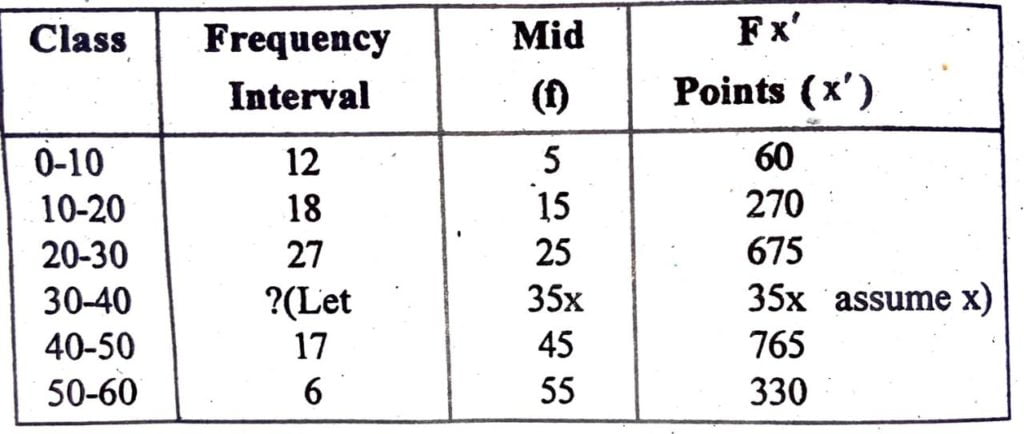
Here, Given
Σf=N=80+x AM=28.
Σfx’= 2100+35x
(a) Now,. we know the formula for AM is-
X̅(AM)= Σfx’/ Σf
⇒28=2100+35x/80+x
⇒ 28 (80+x)=2100+35x
⇒ 2240 +28x = 2100+35x
⇒2240-2100=35x-28x
⇒140=7x ⇒x=20
∴ The value of missing frequency (x) = 20
(b) For calculation of median we rewrite the table as follows
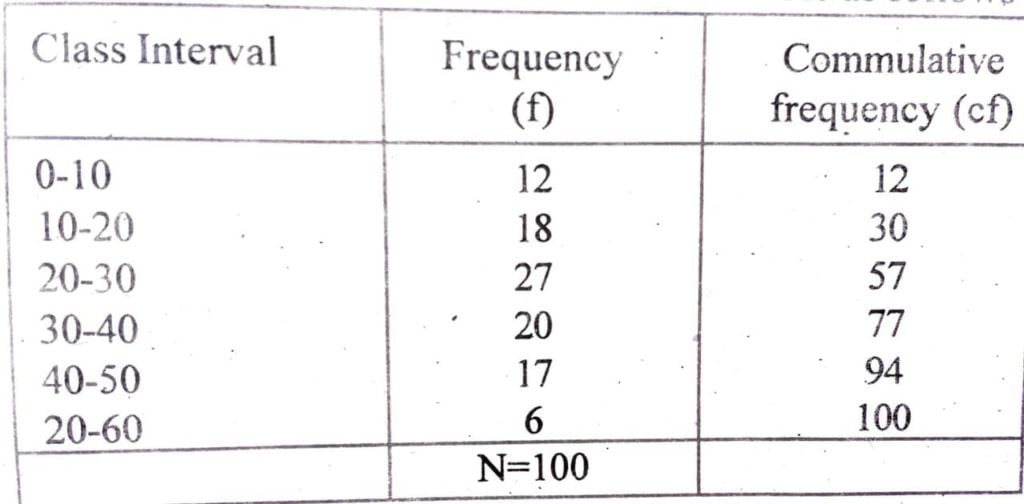
Here,
N/2=100/2 =50
Now, the formula for calculating median is-
Md = 1₁ + N/2-cf /f-xi
Here, Median class=40-50
1₁ = 40
ᶜf=77
f=17
i=10
∴ Md=40+ 50-77 /17 x10
=40+ (-20)/17 x10
= 40- 200/17
= 28.23
10.The following table gives the daily income of ten workers in a factory. Find the arithmetic mean.
Workers: A B C D E F G H I J
Daily Income (in Rs.): 120 150 180 200 250 300 220 350 370 260
Ans: Let, daily income (in Rs.) be X
i.e. X
120
150
180
200
250
300
220
350
370
∴ Σx=2400
We know that, arithmetic mean is given by
X̅=ΣX/N
=2400/10
= 240
∴ The average income is Rs. 240
11. Following information pertains to the daily income of 150 families. Calculate the arithmetic mean
Income (in Rs.) : 75 85 95 105 115 125 135 145
No. of Families: 150 140 115 95 70 60 40 25
Ans: For Calculation we make the following table
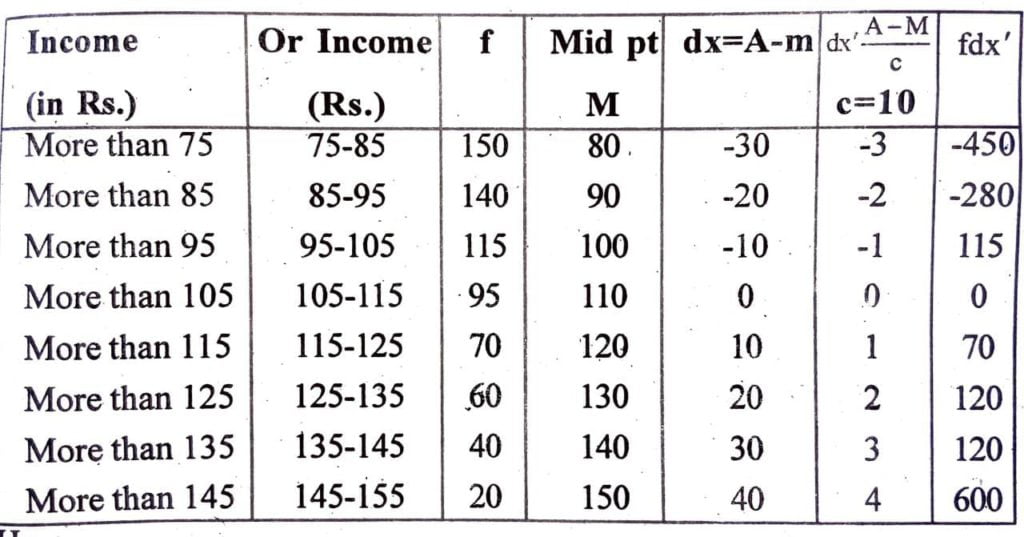
Here,
Σf=N=695
Σfdx’ = 65
A = 110
C=10
∴ Arithmetic Mean
(X̅) = A + Σfdx’/ N×C
= 110+65/695 ×10
= 110+650/695
= 110+0.93
= 110.93
12. The size of land holdings of 380 families in a village is given below. Find the median size of land holdings- Size of land Hold ings (in acres)
Less than 100 100-200 200-300 300-400 400 and above
Number of families 40 89 148 64 39
Ans: For calculation we make the following table-
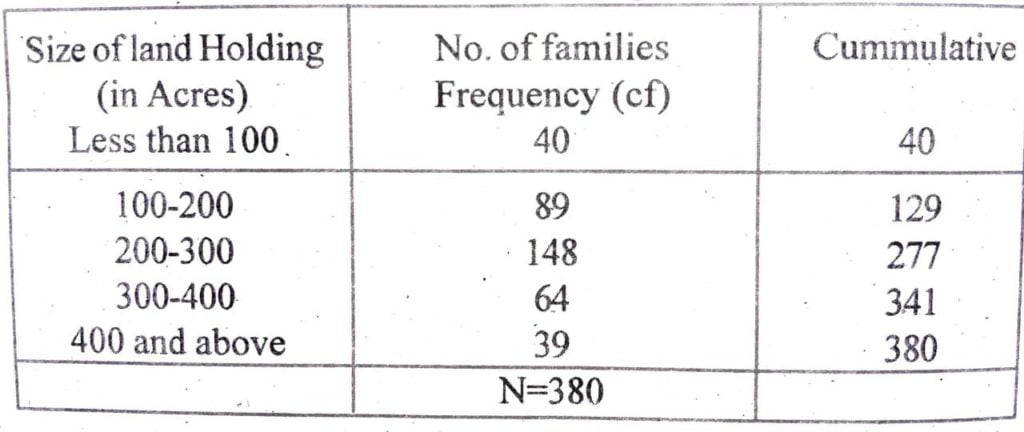
Here, Median = The size of (N/2)ᵗʰ item
= The size of [380/2]ᵗʰ. item
= The size of 190th item, which is 200-300 class. By interpolation:
Median
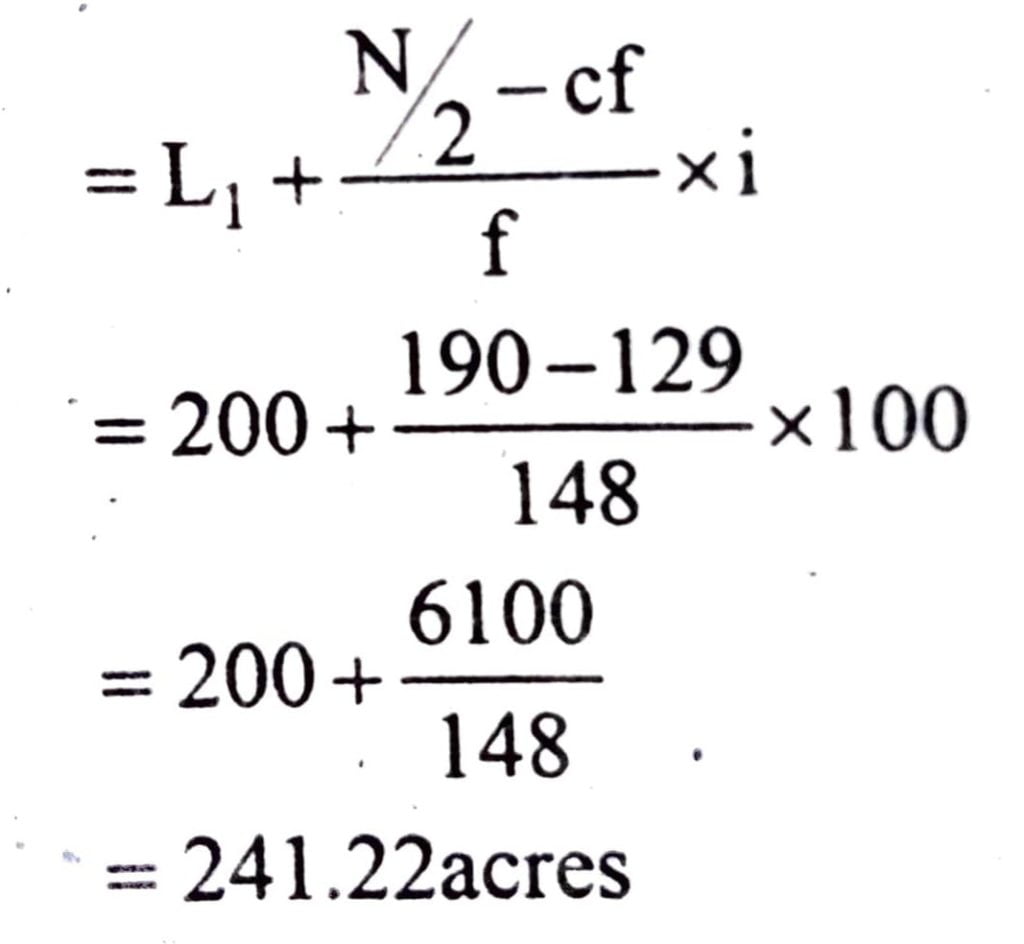
13. The following series relates to the daily income of workers employed in a firm. Compute-
(a) highest income of lowest 50% workers.
(b) minimum income learned by the lowest 25% workers
(c) maximum income earned by the lowest 25% workers.
Daily Income (in Rs.) :10-14 15-19 20-24 25-29 30-34 35-39
Number of workers: 5 10 15 20 10 5
Ans: We have to find,
Median (for highest income of lowest 50%
Q₃(for minimum income by top 25%)
Q₁(for maximum income by lowest 25%) For calculation we make the following table-
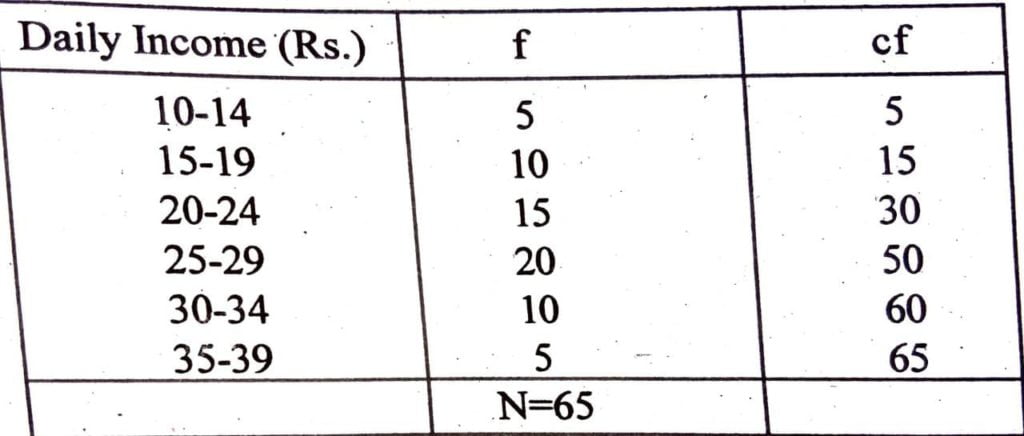
Q₁=The size of N/4 the item
= The size of 65/4 the item i.e. 16.25
∴ Q₁ class in 20-24 and adjusting the class with correction factor it is 19.5-24.5
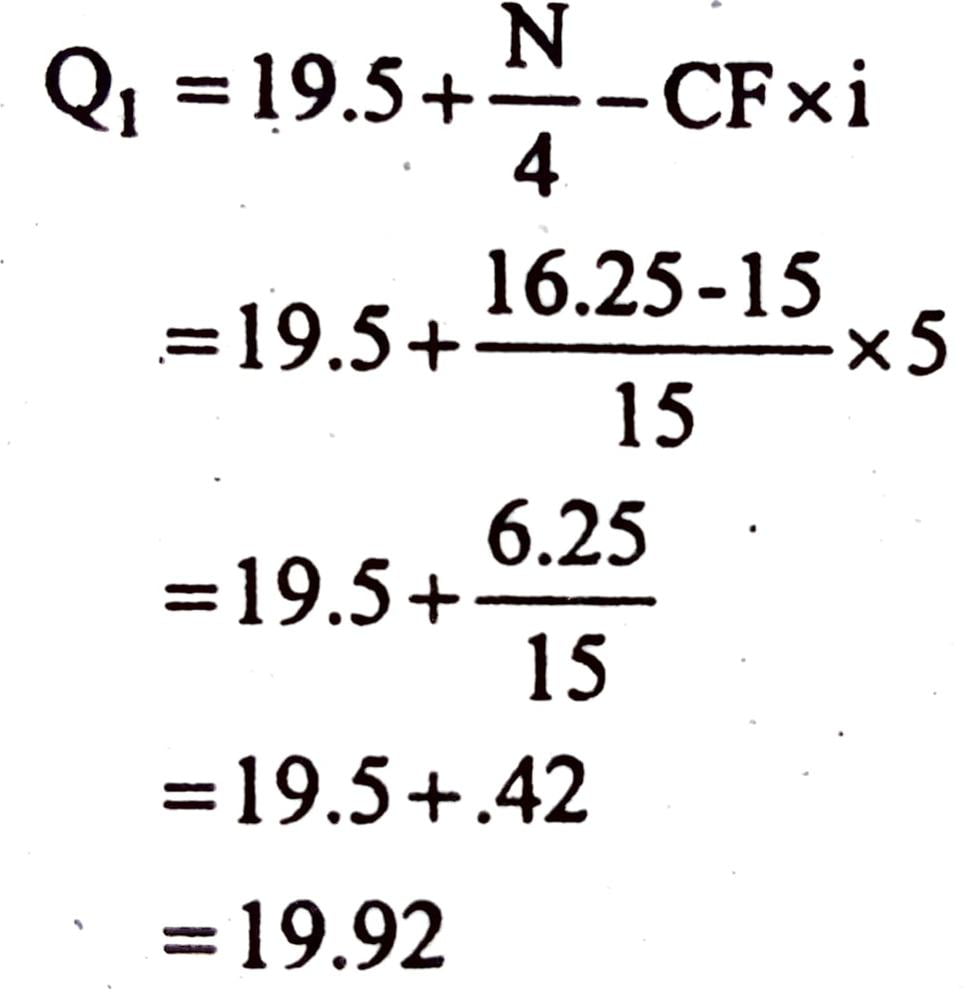
Median = The size of N/2 the item
=The size of 65/2 the item is 32.5; it lies in 25-29 class. By adjusting with correction factor it is 24.5-29.5
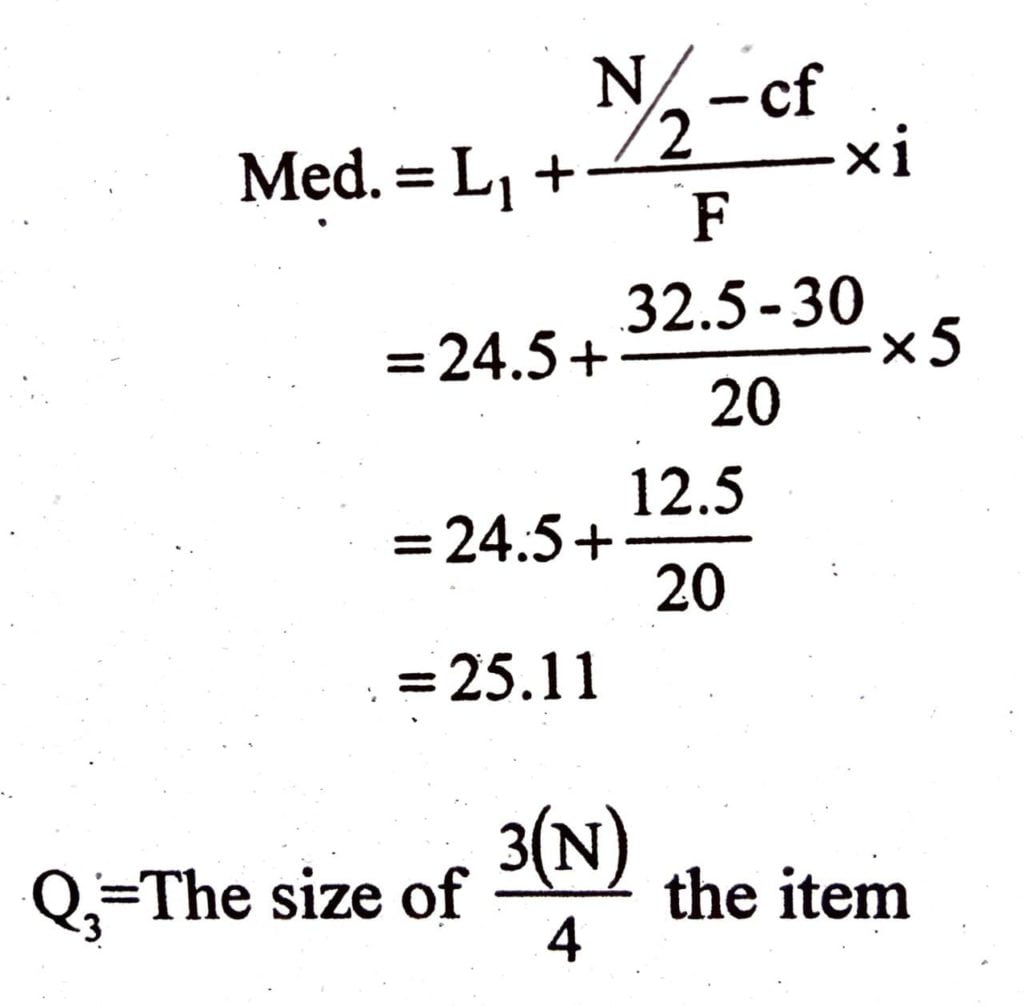
= The size of 3(65)/4 the item, i.e. 48.75 which lines in 50 and Q₃ class in 25-29. By adjusting the class with correction factor it would be 24-5-29.5
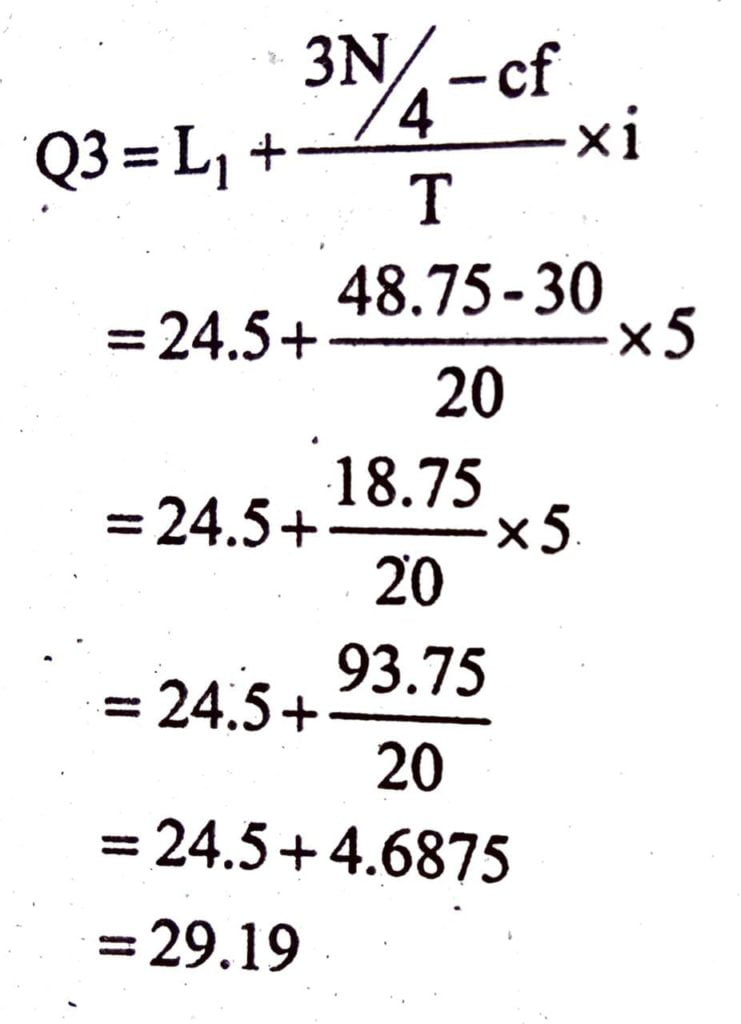
14. The following table give production yield in kg. per hectare of wheat of 150 farms in village. Calculate the mean, Median and Production yield.
Production (kg. per hectare) : 50-53 53-56 56-59 59-62 62-65 65-68 68-71 71-74 74-77
Number of farms: 3 8 14 30 36 28 16 10 5
Ans: For Calculation we make the following table.

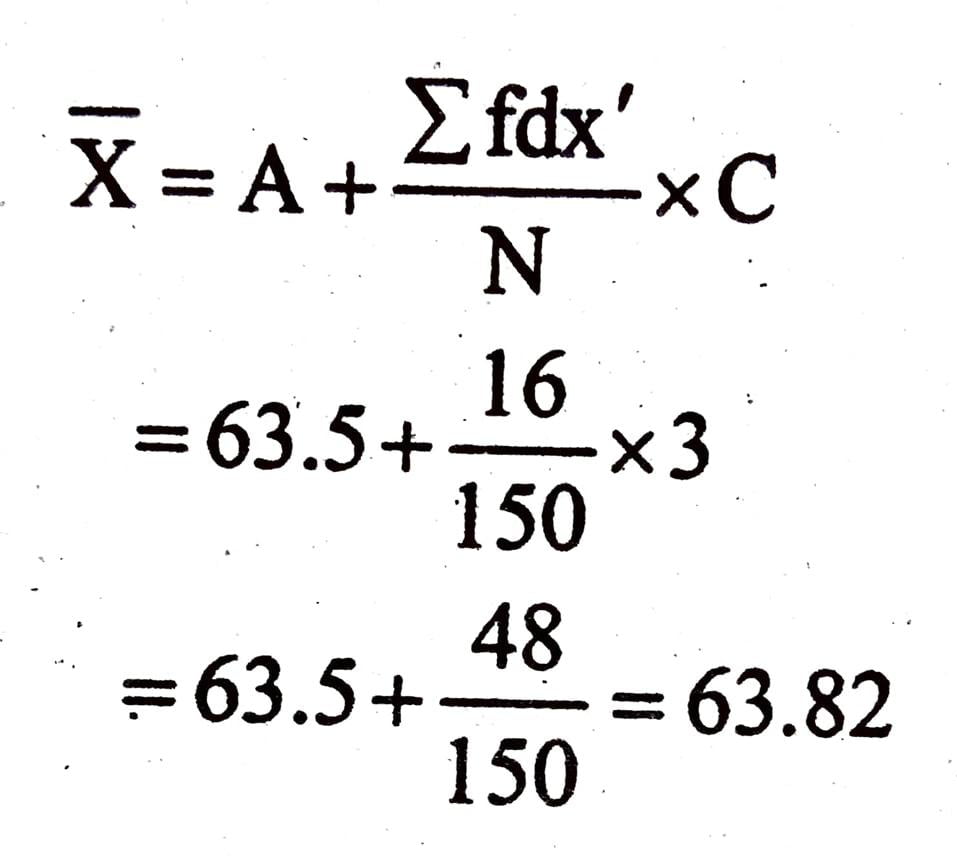
Med = The size of N/2 the class
= The size of 150/2 the class
=The size of 75ᵗʰ class, 75 lies in 91 ∴ Median class is 62-65
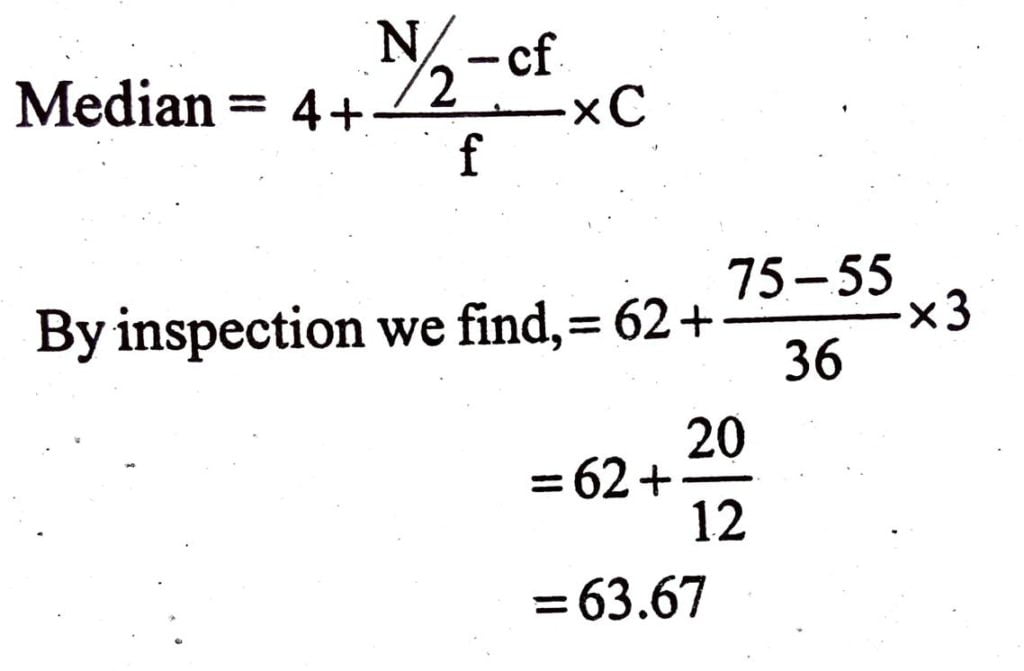
Maximum frequency lies in 62-65
∴ it is mode class
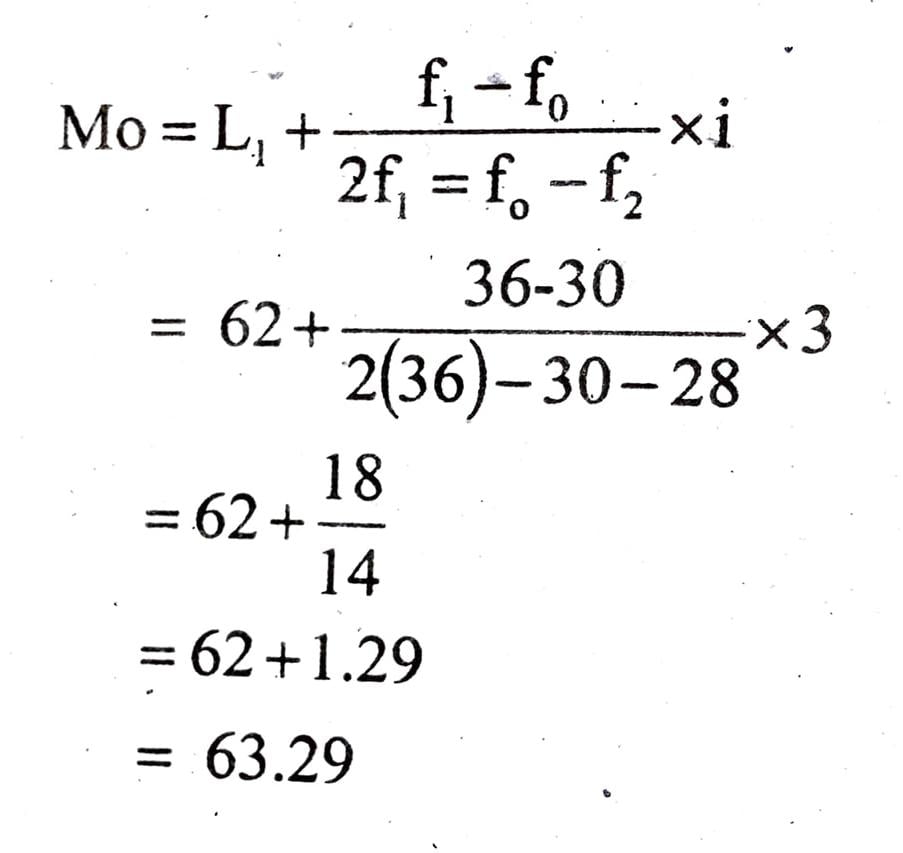
15. Write the formula of mean in case of individual series.
Ans: The formulas are-
(a) Direct /Method :
X̅= ΣΧ/N,
Where,
N=member of items.
ΣX=Sum of the Size of items.
(b) Short Cut Method:
X̅=A+ Σdx/,N
Where,
A = assumed mean
N = number of items.
Ed=X-A-Sum of deviations
(c) Step Deviation Method:
X̅ = A + Σdx’/N×C
Where,
X`=mid points
C-difference in class interval.
16. Write the formula of mean in case of discrete series.
Ans: The formulas are
(a) X̅=Σfx/N (Direct Method)
(b) X̅=A+Σfd/N (Short Cut Method)
(c) X̅=A+Σfd/N×C (Step Deviation Method)
17. Write the formula of mean in case of continuous series.
Ans: The formulas are-
(a) X̅=A+Σfx/N (Direct Method)
(b) X̅=A+Σfd/N (Short Cut Method)
(c) X̅=A+Σfd/N×C (Step Deviation Method)
18. Write the formula of median in case of individual observations.
Ans: Median = Size of[N+1/2] item (if the series is odd)
and Median = Size of [N/2]ᵗʰ item (if the series is even)
19. Write the formula of median in case of continuous series.
Ans: Mediam (Md)= 1₁+N/2-cf/f×i
Where
L₁= lower limit of the class interval.
cf = Cumulative frequency before the median class.
f = frequency of the median class.
i = difference in the class interval.
20. Define Quartiles ?
Ans: It is measure which divides the series into four equal parts. [Each part contain equal number of items. The first point is termed as first quartile (Q₁), second point is termed as second quartile or median (Q₂) aval third point is termed as third quartile (Q₃)
first quartile is given as, Q₁=L₁+N/4-cf/fxi
The third quartile is given as, Q₁ = L₁ + 3N/4-cf/fxi
21. Write the formula for calculation of Mode in continuous series.
Ans: Made
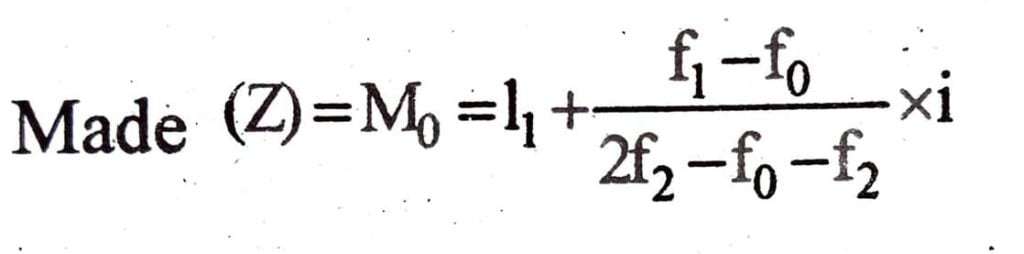
Where,
1₁=lower limit of the model class
f₁= frequency of the model class
f₀=frequency of the class preceding the model class
f₂= frequency of the class succeeding the model class
i= difference between upper and lower limits.
OR
Alternative Formula
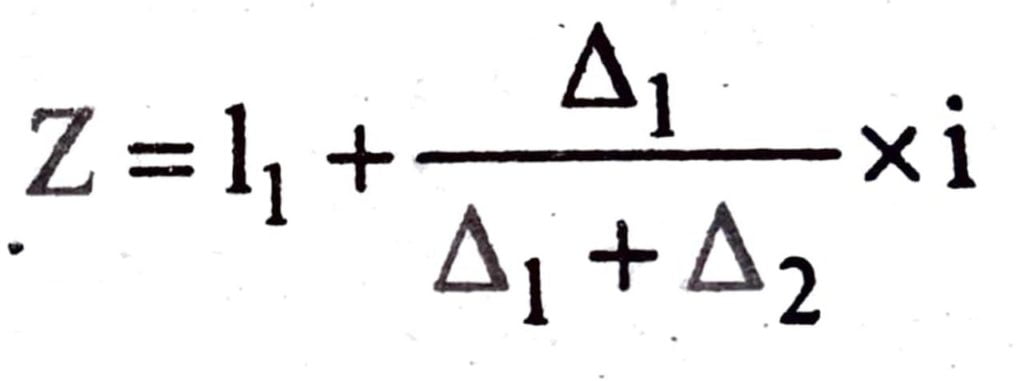
Where, Δ₁=|f₁-f₀|
Δ₂=|f₁-f₂|

Hi, I’m Dev Kirtonia, Founder & CEO of Dev Library. A website that provides all SCERT, NCERT 3 to 12, and BA, B.com, B.Sc, and Computer Science with Post Graduate Notes & Suggestions, Novel, eBooks, Biography, Quotes, Study Materials, and more.



