NIOS Class 10 Economics Chapter 18 Analysis of Data Solutions to each chapter is provided in the list so that you can easily browse through different chapters NIOS Class 10 Economics Chapter 18 Analysis of Data and select need one. NIOS Class 10 Economics Chapter 18 Analysis of Data Question Answers Download PDF. NIOS Study Material of Class 10 Economics Notes Paper 214.
NIOS Class 10 Economics Chapter 18 Analysis of Data
Also, you can read the NIOS book online in these sections Solutions by Expert Teachers as per National Institute of Open Schooling (NIOS) Book guidelines. These solutions are part of NIOS All Subject Solutions. Here we have given NIOS Class 10 Economics Chapter 18 Analysis of Data, NIOS Secondary Course Economics Solutions for All Chapters, You can practice these here.
Analysis of Data
Chapter: 18
MODULE 6: PRESENTATION AND ANALYSIS OF DATA IN ECONOMICS
TEXTBOOK QUESTIONS (SOLVED)
INTEXT QUESTIONS 18.1
Q.1. Give an example of central tendency.
Ans. Arithmetic mean.
Q.2. Give basic formula to calculate arithmetic mean.
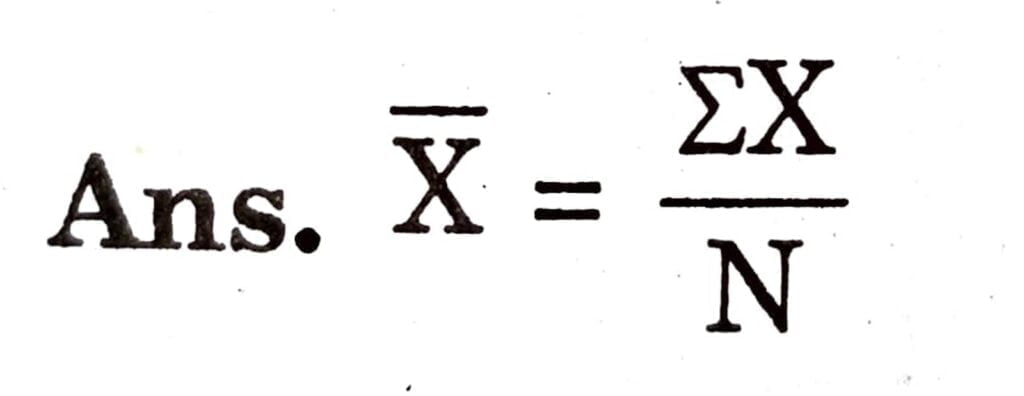
Q.3. Of sum of items is 40 and mean is 4, find out N (number) of items.
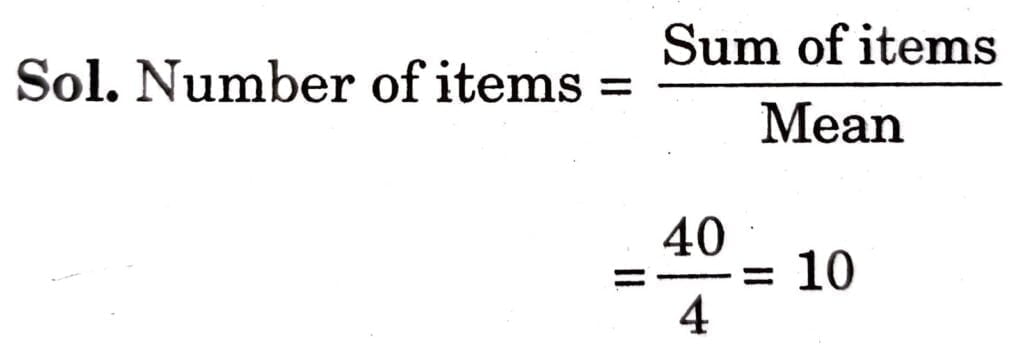
Q.4. Weekly consumption of sugar of Mr. Raja is 35 kg, what is the average daily consumption?
Q.5. If average marks of 10 students is 50, if one more student is added to group who secured 5 marks, what would be the new average?
INTEXT QUESTIONS 18.2
Q.1. Calculate arithmetic mean from the following data:
4, 6, 3, 7, 8, and 5
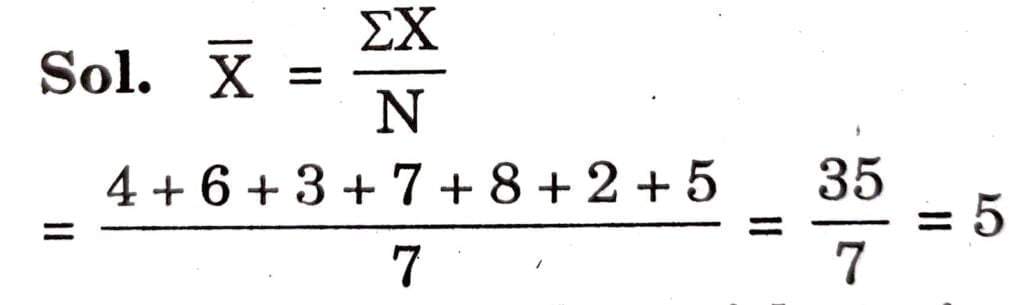
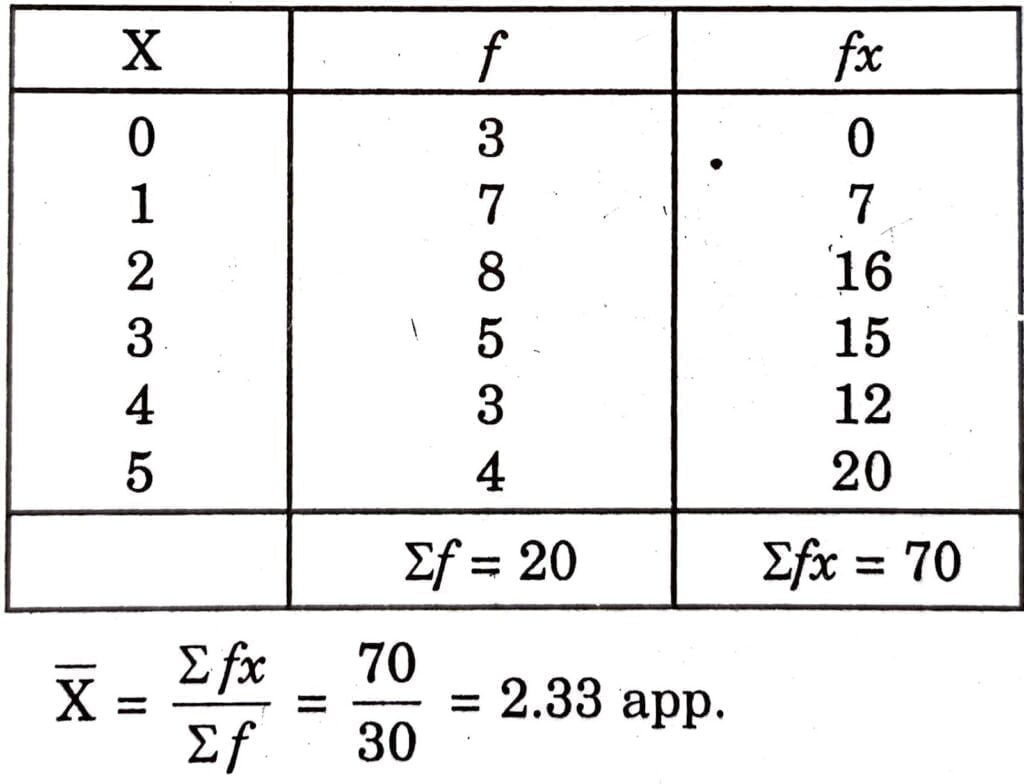
Q.2. Calculate the arithmetic mean from the following data:
Sol.
Q.3. If sum of the deviation measured from the assumed mean of a group of 10 is + 50 and assumed mean is 20, what will be the actual mean?
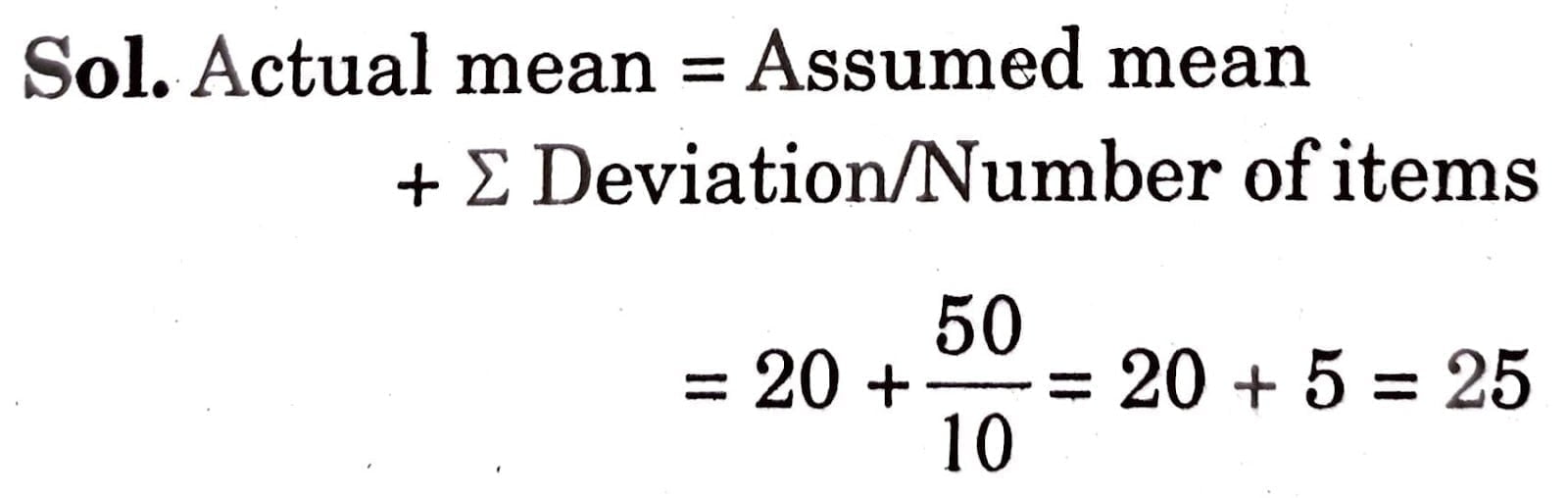
TERMINAL EXERCISE
Q.1. What do you mean by analysis of data?
Ans. Analysis of data is a technique through which significant facts from the numerical data are extracted.
Q.2. What is meant by descriptive analysis?
Ans. Descriptive analysis means getting one single value that describes the characteristics of the whole data.
Q.3. Describe the concept of central tendency.
Ans. Central tendency means tendency of data to cluster towards the central location or value.
Q.4. What do you mean by arithmetic mean? How is it calculated?
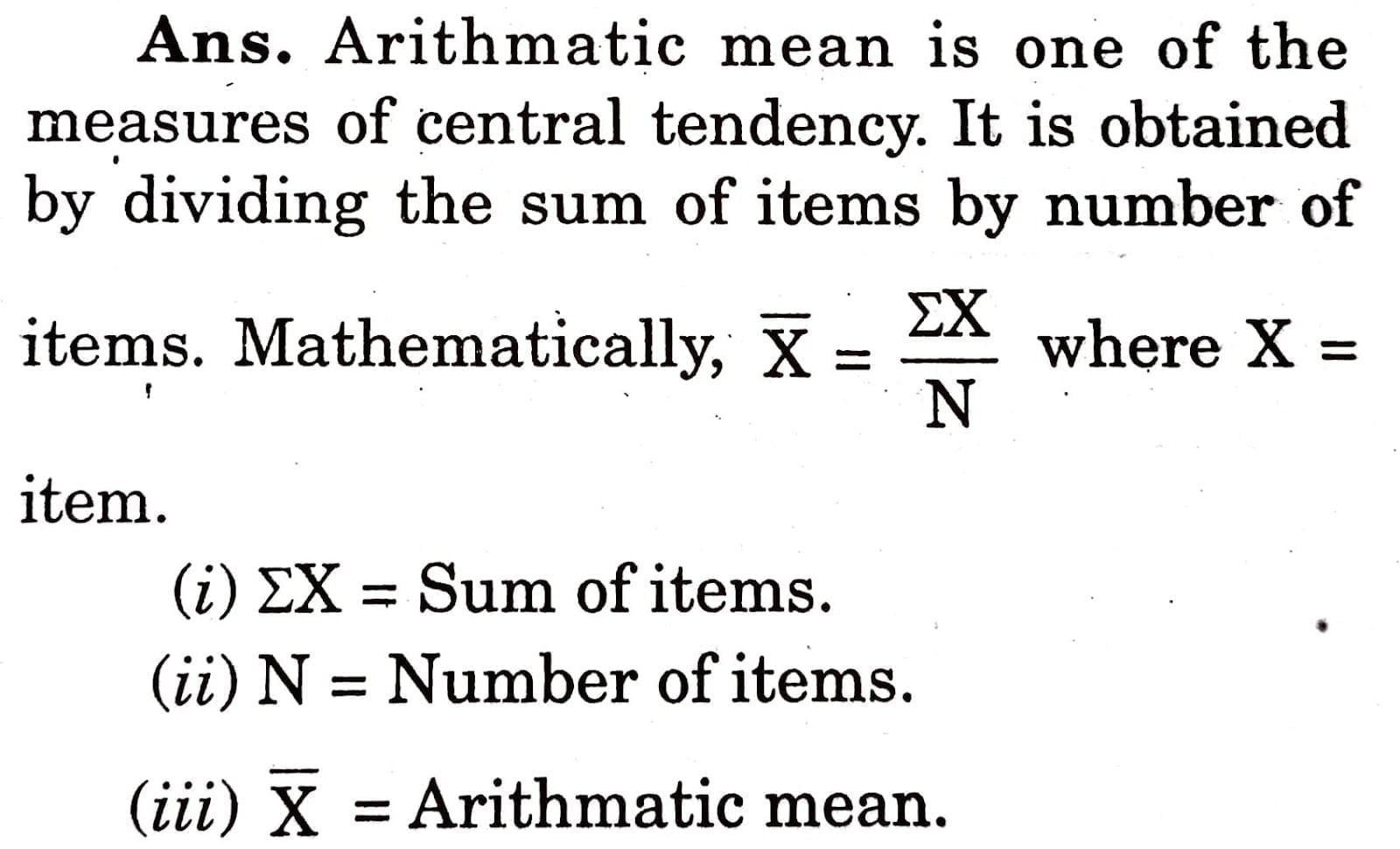
Q.5. Define arithmetic mean.
Ans. Arithmetic mean a measure of central tendency. It represents all the data in a series.
Q.6. What do you mean by central tendency?
Ans. Tendency of data to cluster towards the central location or value is called central tendency.
Q.7. From the following data, find out the mean:
7, 4, 17, 19, 11, 16, 14, 9 and 11.
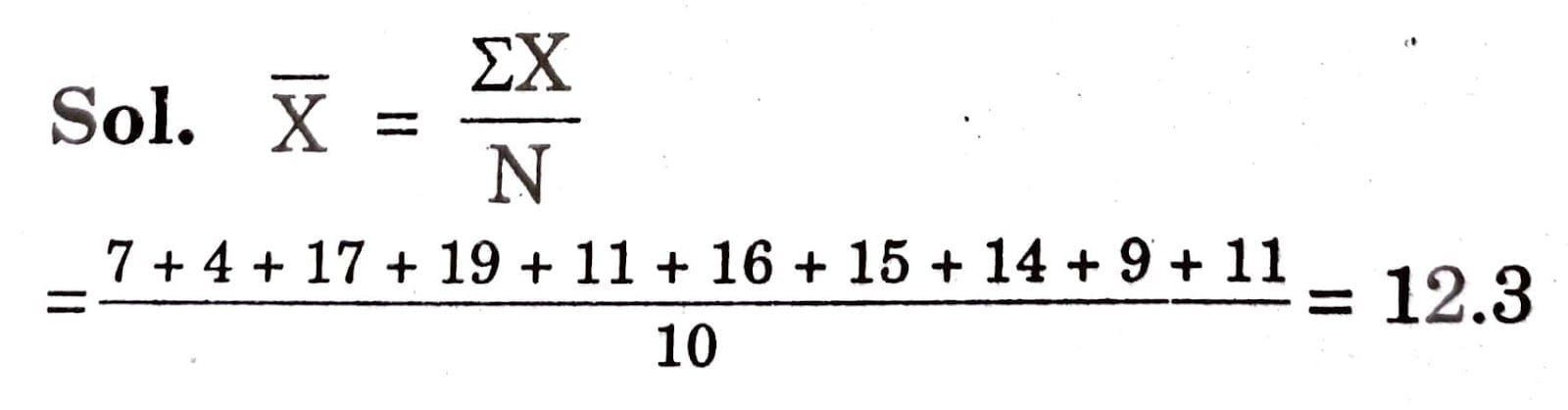
Q.8. If the following items are also added to above set of data (given in Question 7) what would be the revised mean? 18, 14, 14, 8, 10 and 21.
Ans. Sum of 16 items = 123 + 18 + 14 + 14 + 8 + 10 + 21 = 208
No. of items = 10 + 6 = 16
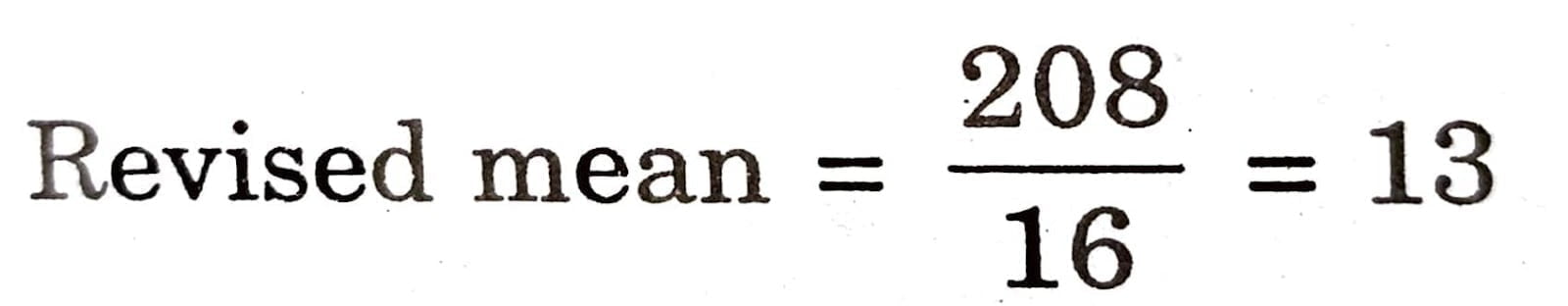
Q.9. Calculate mean from the data given below:
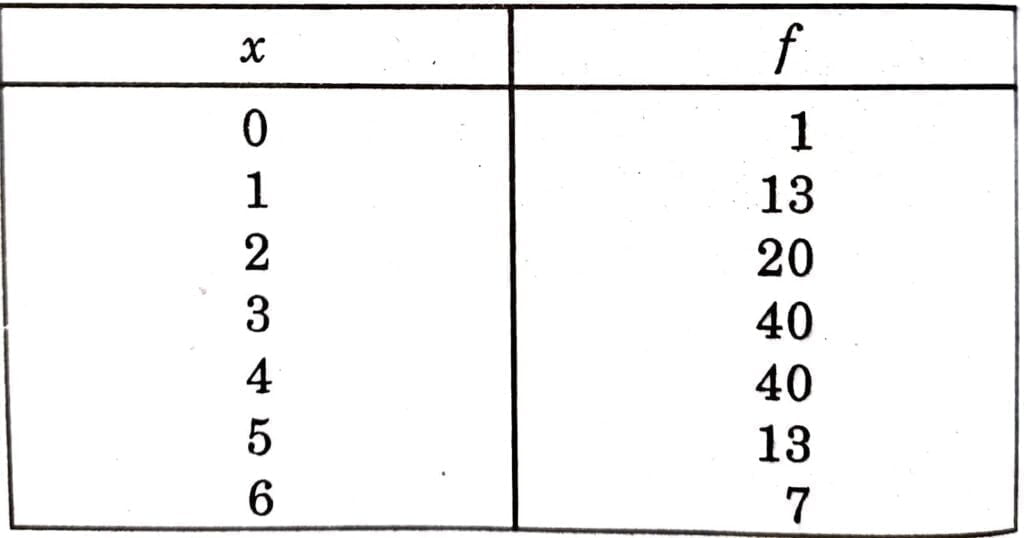
Ans.
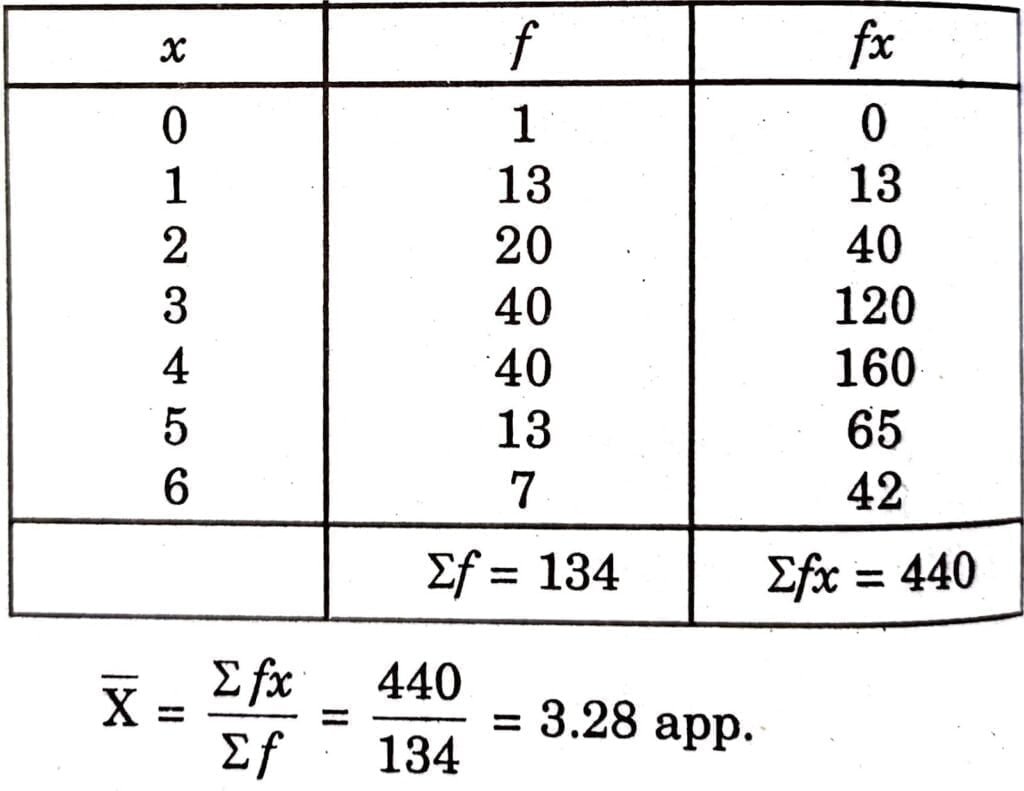
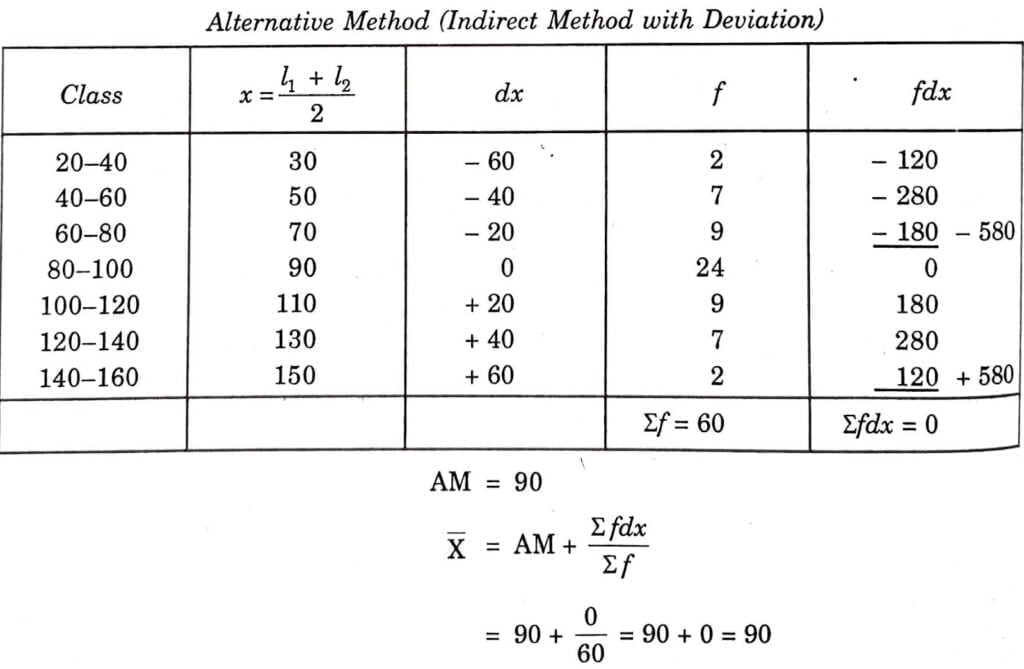
Alternative Method
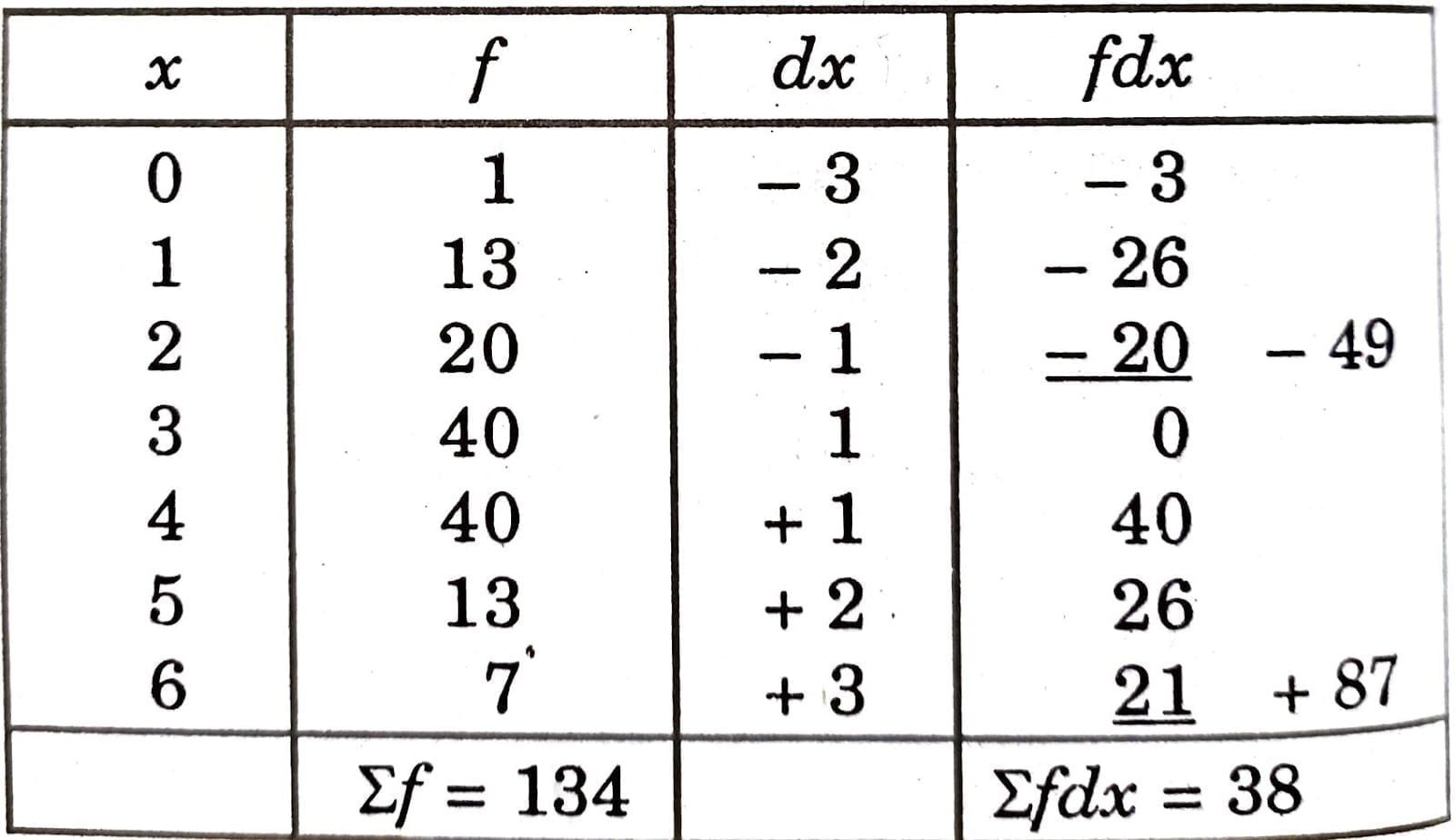
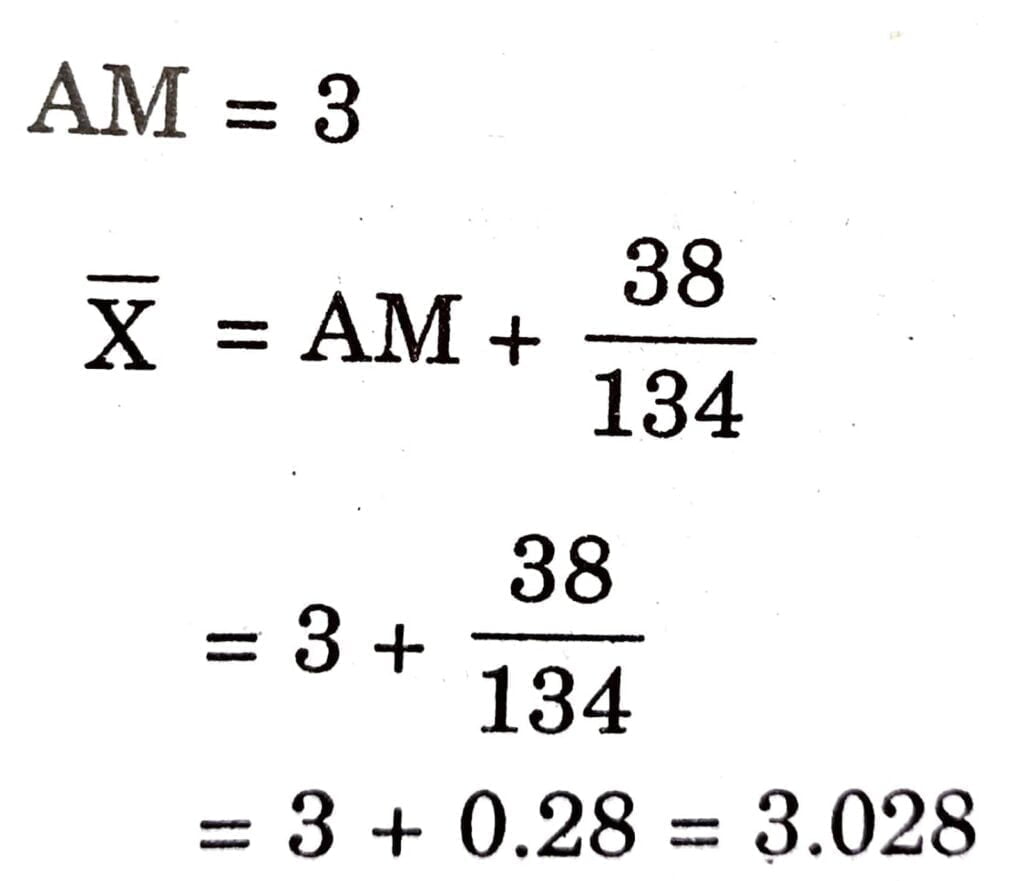
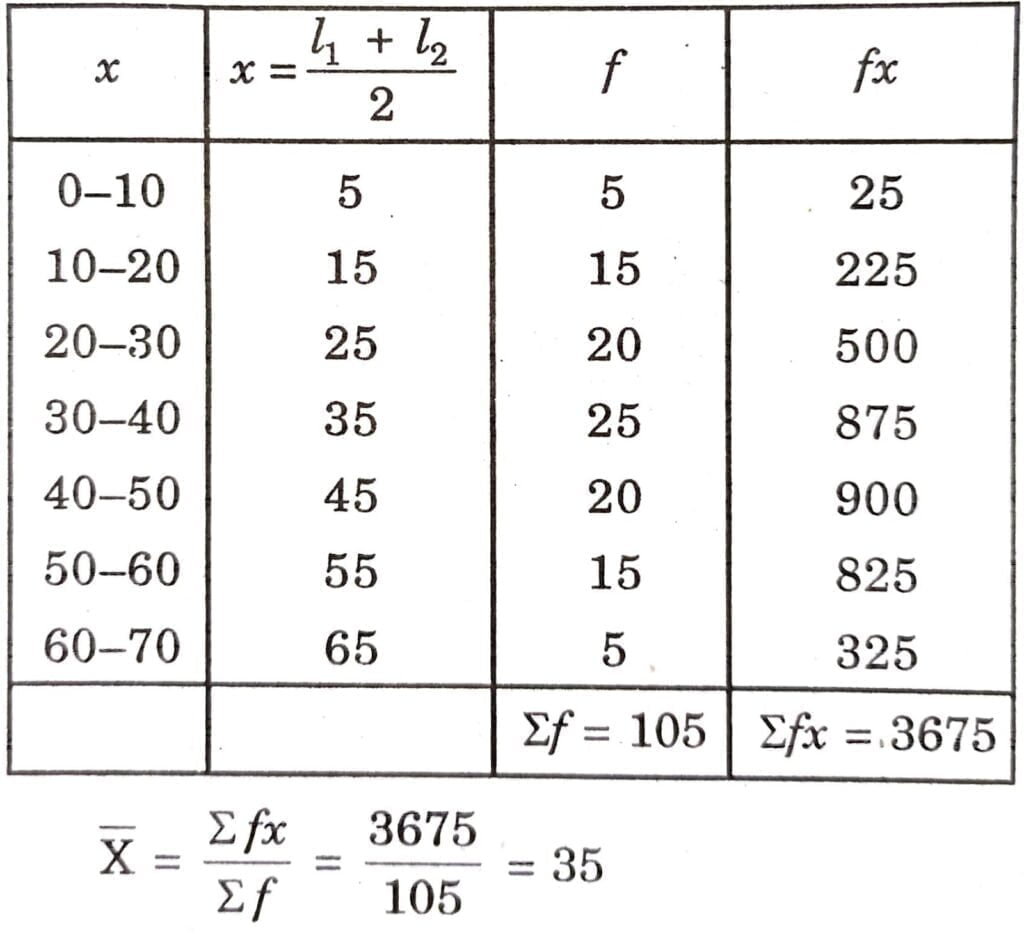
Q.10. Calculate arithmetic mean from the data given below:
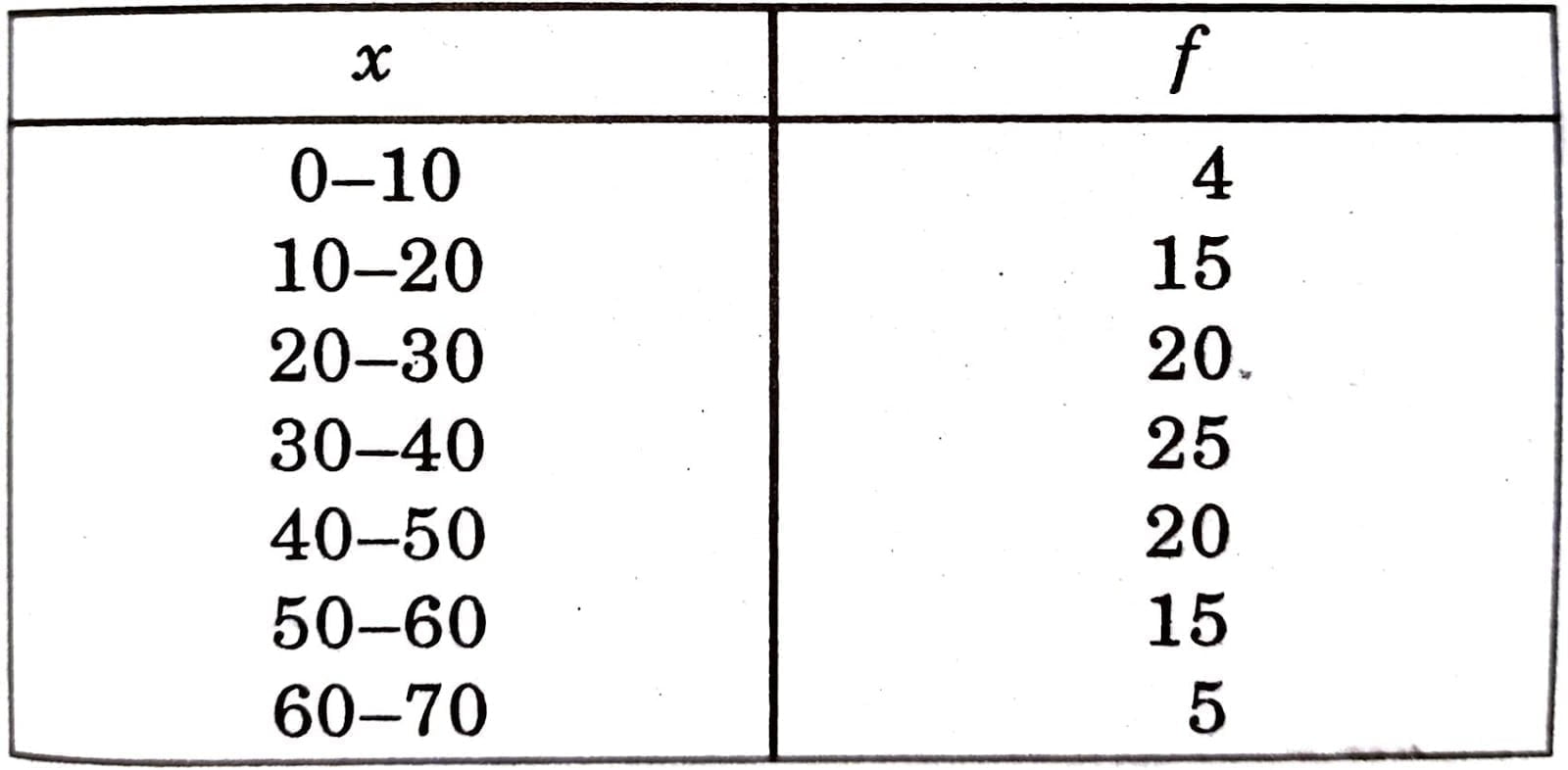
Ans. Direct Method
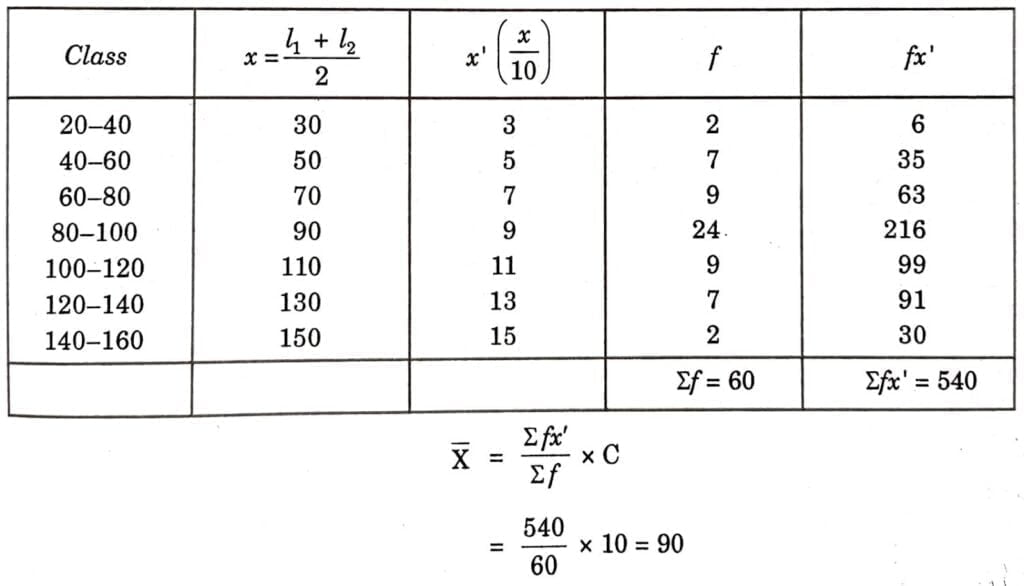
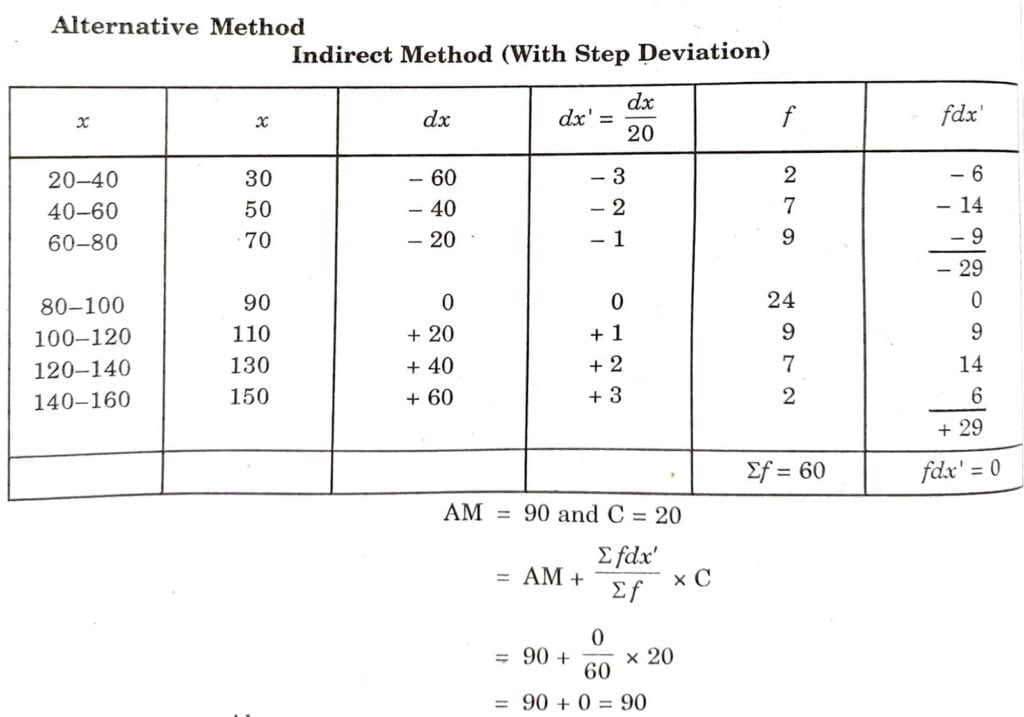
Q.11. From the data given below find out mean:
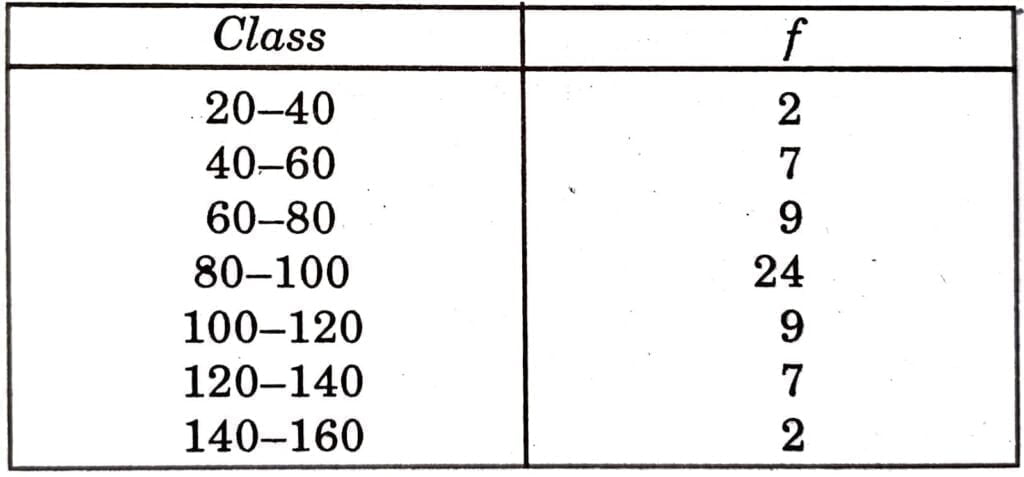
Ans. Direct Method (With Step Deviation)
SOME IMPORTANT QUESTIONS FOR EXAMINATIONS
VERY SHORT ANSWER TYPE QUESTIONS
Q.1. What is central tendency?
Ans. Tendency of data to cluster towards the central location or value is called central tendency.
Q.2. What is the first step in the analysis of statistical data?
Ans. The first step in the analysis of statistical data is to determine the representative value (average or measure of central tendency) from the raw data.
Q.3. What is the other name of the measure of central tendency?
Ans. Average or statistical measure.
Q.4. State measures of central tendency.
Ans. (i) Arithmetic Mean.
(ii) Median. and
(iii) Mode.
Q.5. What is arithmetic mean?
Ans. Arithmetic mean is that value which is obtained by dividing the sum of items by the number of items.
Q.6. Pocket allowances of six students are ₹ 6, 12, 18, 24, 30 and 36. Calculate arithmetic mean.
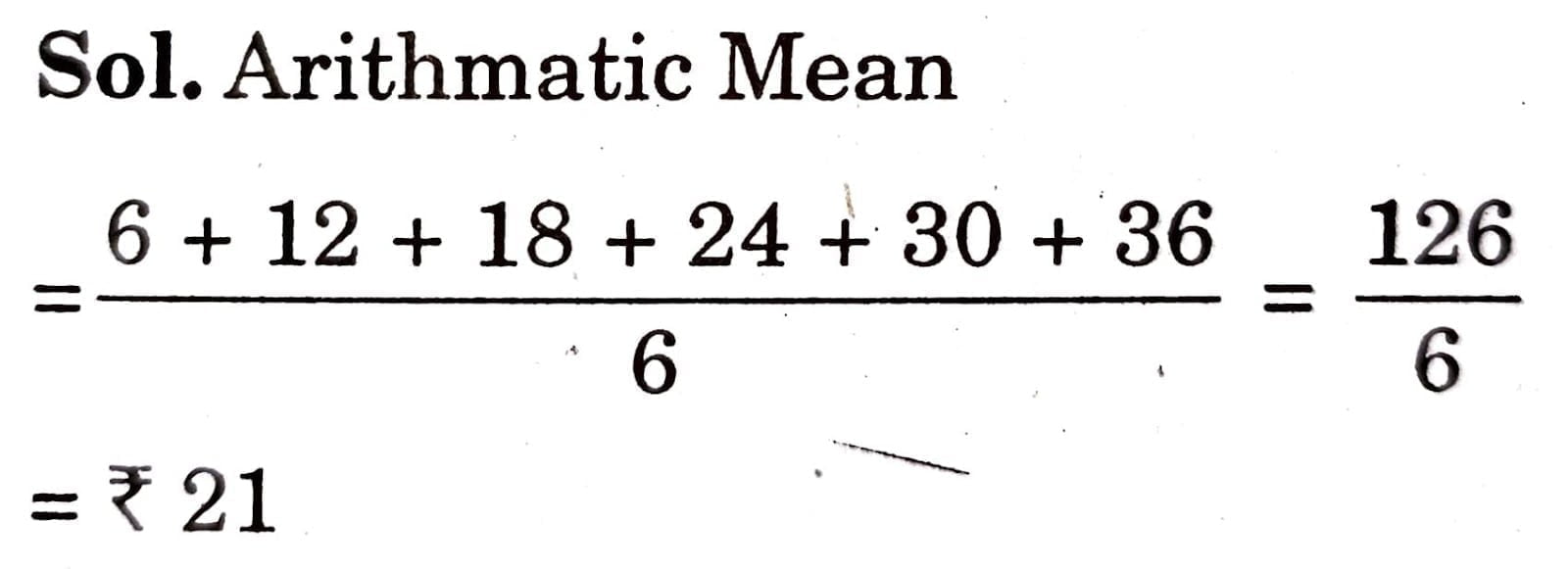
Q.7. What is the purpose of computing an average value for a set of data?
Ans. The purpose of computing an average value for a set of data is to obtain a single value which is representative of all the items and which the mind can graph simply and quickly.
Q.8. Mohan says that he has secured average 76 marks in six subjects Calculate the total marks secured by him.
Ans. Total Marks
= Average Marks × No. of Subjects
= 76 × 6 = 456
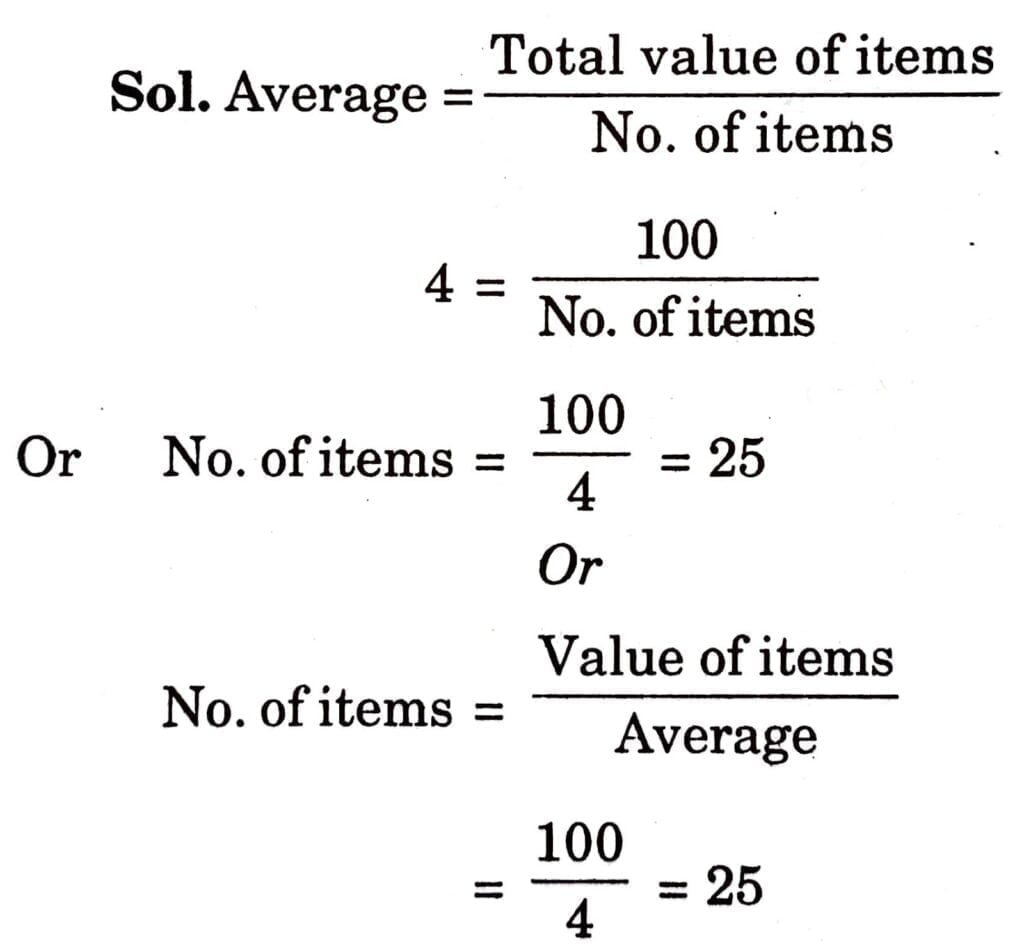
Q.9. Total value of items is 100 and their average is 4. Calculate the number of items.
Q.10. Write the formula for computing arithmetic mean in individual series by direct method.
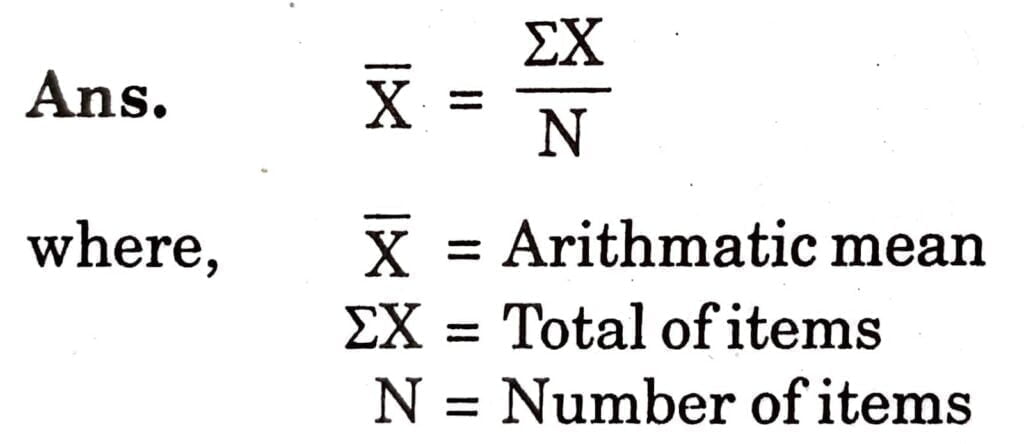
Q.11. Write down the formula for individual arithmetic mean in individual series by indirect Method (without step deviation).
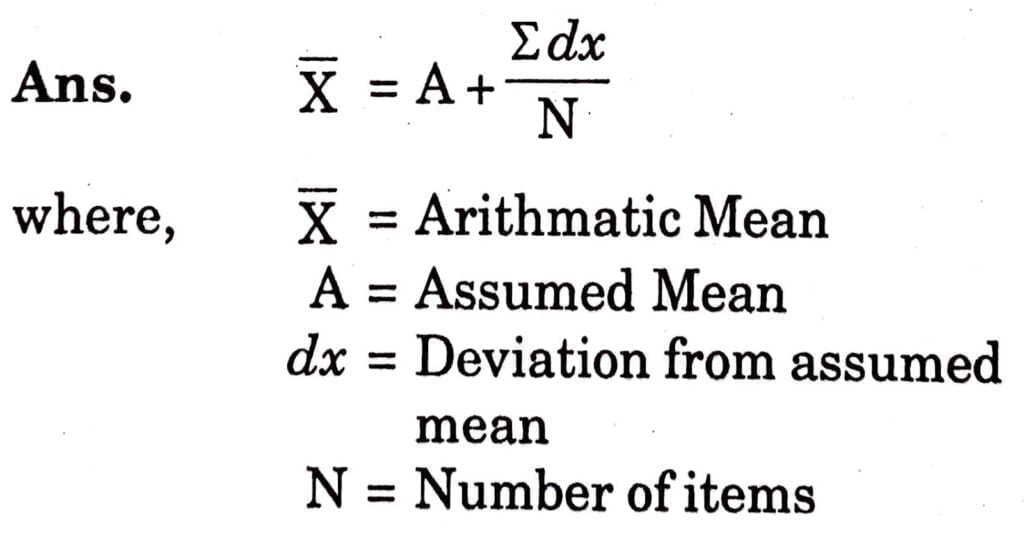
Q.12. Write down the formula for computing arithmetic mean in discrete series by method.
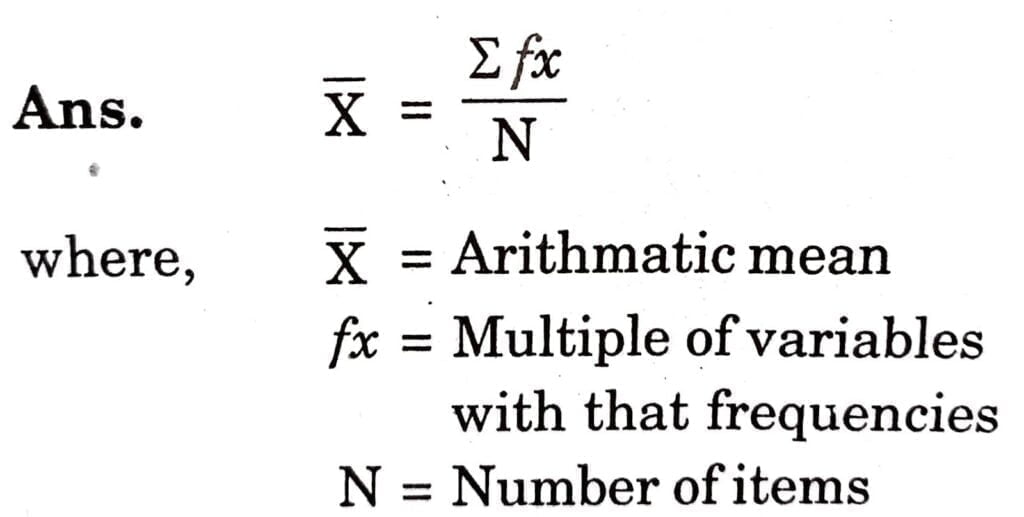
Q.13. Write down the formula of calculating arithmetic mean in direct series by indirect method (without step deviation).
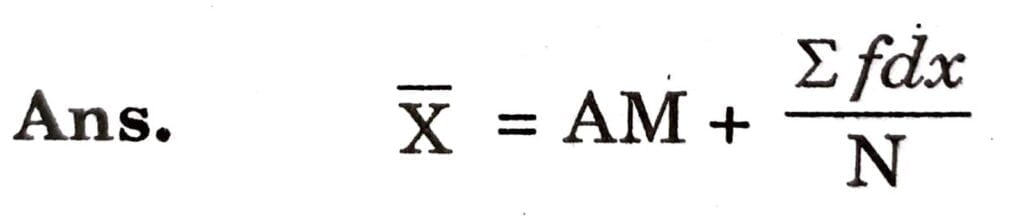
Q.14. Write down the formula of calculating arithmetic mean in discrete series by indirect method (with step deviation).
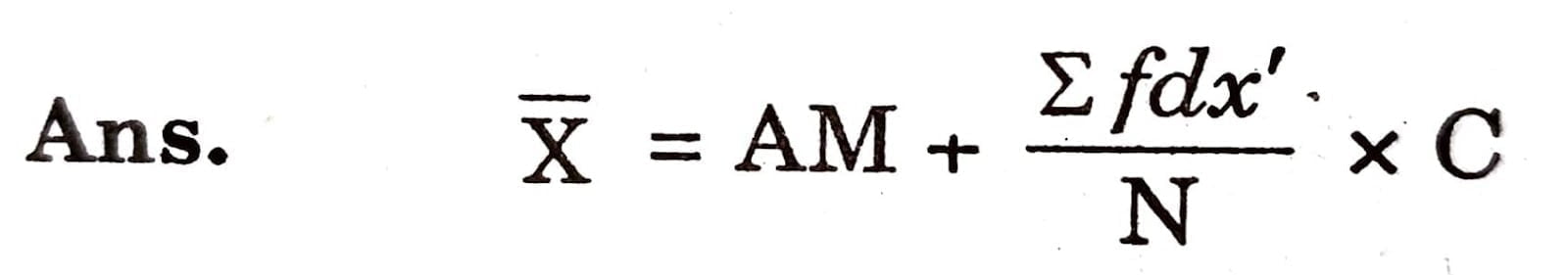
Q.15. Total marks secured by students of school are 800 and arithmetic mean of marks is 32. Calculating the number of students.
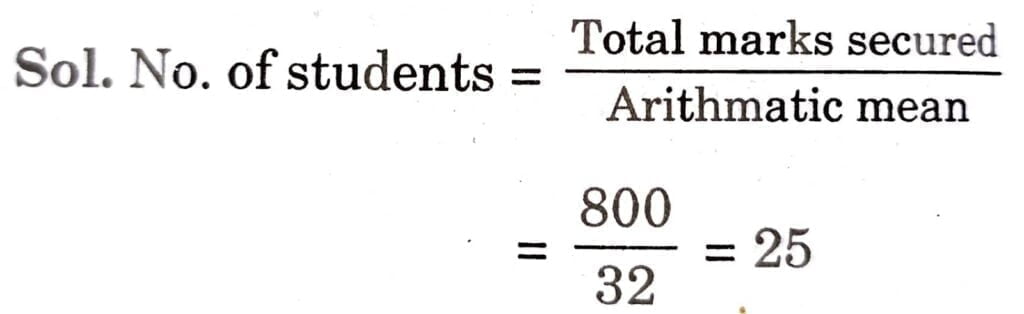
Q.16. State the steps involved in the computation of arithmetic mean in individual series.
Ans. (i) Obtain the total of variables and donate it by ΣΧ.
(ii) Denote the number of items as N.
(iii) Divide ΣΧ by N for calculating arithmetic mean.
Q.17. Write down the steps involved in calculating arithmetic mean by indirect method (without step deviation) in individual series.
Ans. Steps: (i) Take any variable as assumed mean and denote it by AM.
(ii) Calculate deviation from the assumed mean and denote it as dx.
(iii) Obtain the total deviation.
(iv) Use the formula.
SHORT TYPE QUESTIONS ANSWER
Q.1. Calculate the steps involved in the calculation of arithmetic mean by short-cut method (without step deviation) in discrete series.
Ans. Steps: (i) Take assumed mean and denote it by AM.
(ii) Calculate deviations from assumed mean and denote by dx.
(iii) Multiply deviations by frequency and denote it by fdx.
(iv) Obtain the total of fdx and denote it by Σfdx.
(v) Use the following formula:
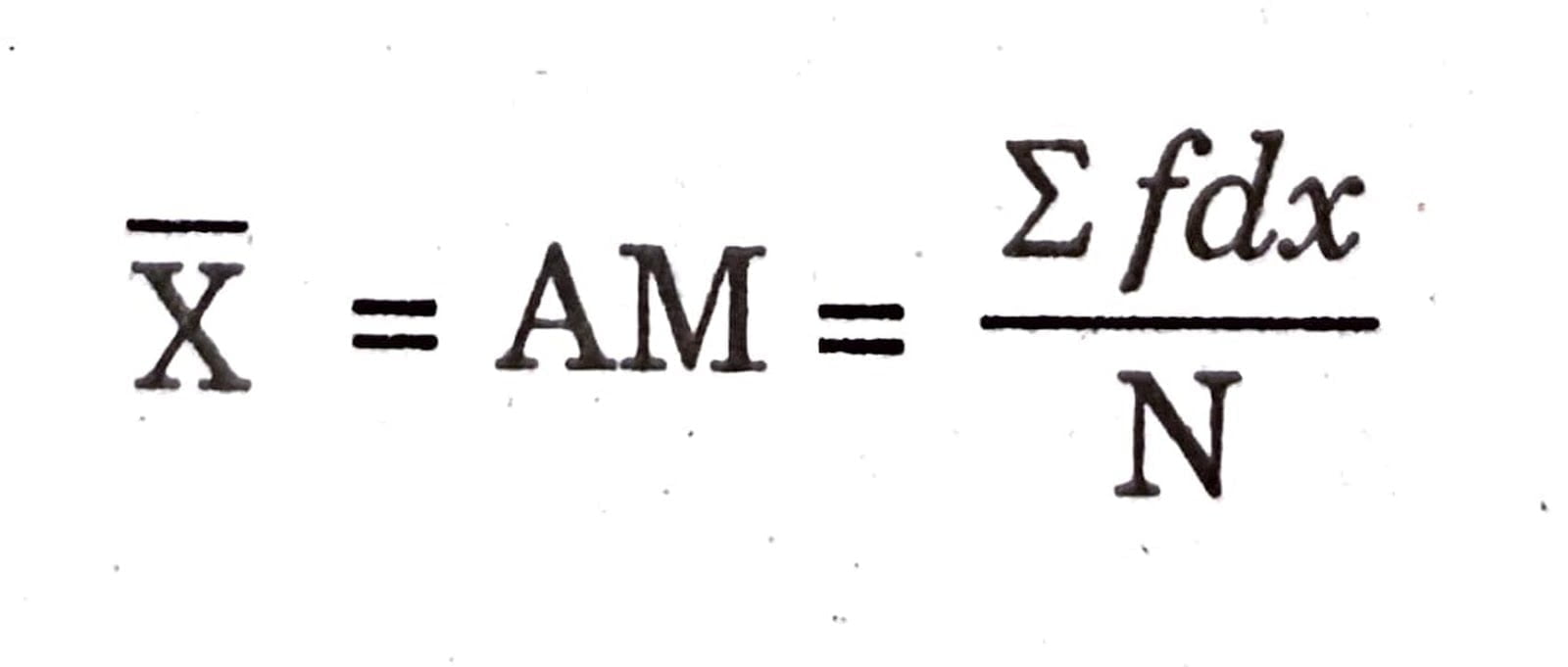
Q.2. Write down the steps for calculating arithmetic mean by short- cut method (with step deviation) in discrete series.
Ans. Steps: (i) Take assumed mean and denote by AM.
(ii) Calculate deviation from assumed mean and denote it as dx.
(iii) Divide deviation by common factor and denote it d’x.
(iv) Multiply step deviation by frequency and obtain fd’x.
(v) Calculate the total of frequency and denote it by N (Σf).
(vi) Use the following formula:
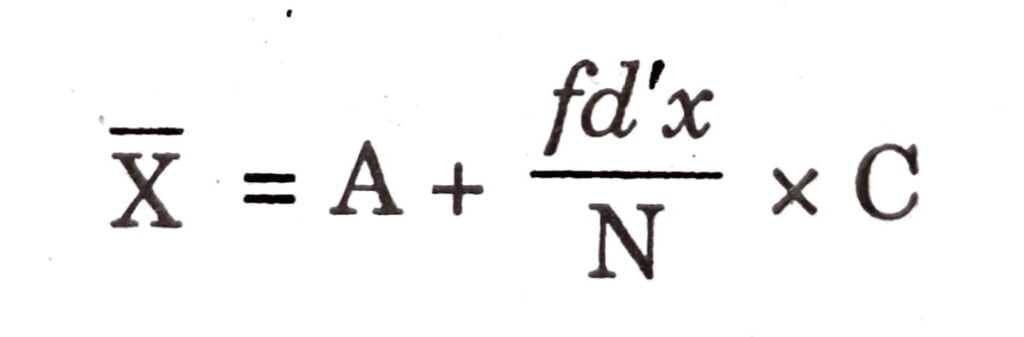
Q.3. Write down the steps for calculating arithmetic mean by indirect method (without step deviation) in continuous series.
Ans. Steps: (i) Ascertain mid-points of every class-interval by applying the following formula:
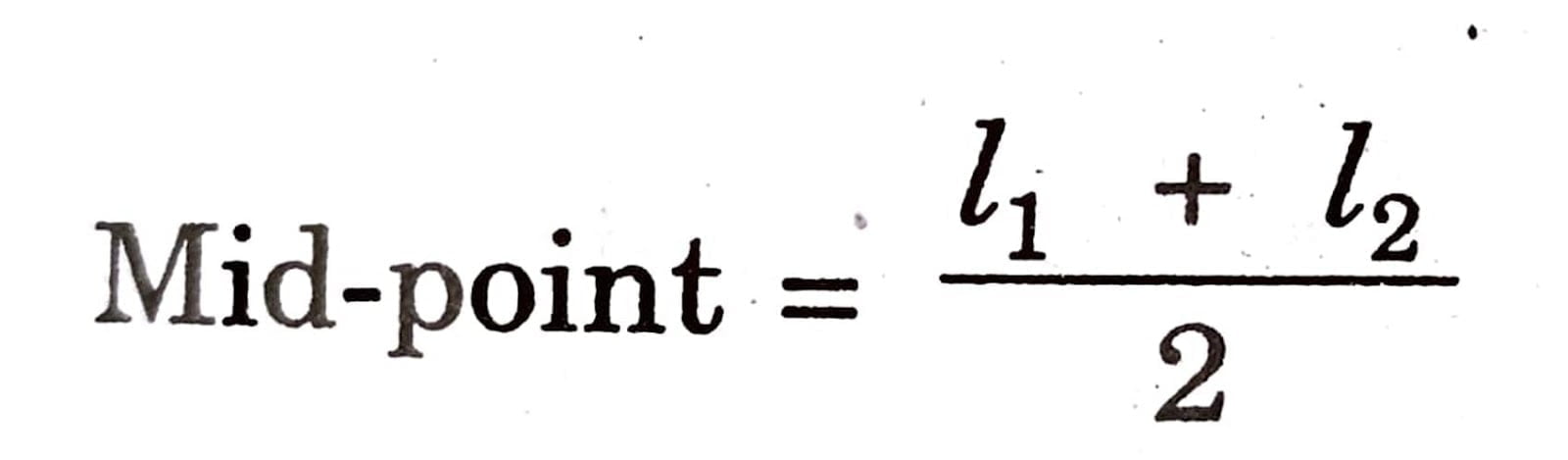
(ii) Multiply mid-points by frequency and find fx of each class.
(iii) Sum up the frequency.
(iv) Divide the fx by number of frequency for calculating arithmetic mean.
Q.4. Write down the steps involved in calculating arithmetic mean by indirect method (with step-deviation) in continuous series.
Ans. Steps: (i) Obtain mid-points of class- intervals by applying following formula:
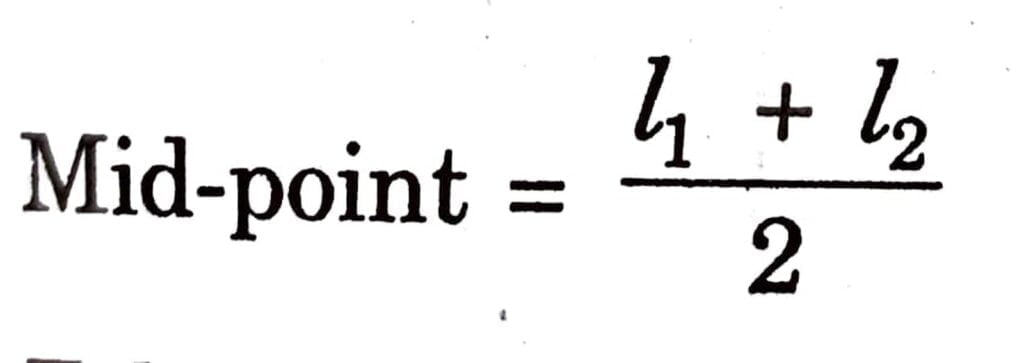
(ii) Take assumed mean.
(iii) Take deviations from assumed mean.
(iv) Divide deviation by common factor.
(v) Multiply step deviation by frequency.
(vi) Apply following formula:
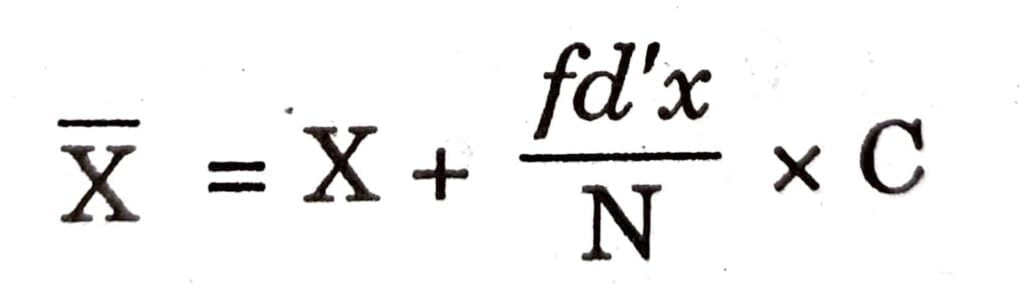
Q.5. Average height of 50 students in a class is 61 inches and that of 70 students of another class is 58 inches. Calculate average height of 120 students.
An. Total height of 50 students = 61 × 50 = 3050 inches
Total height of 70 students = 58 × 70 = 4060 inches
Total height of 120 students = (3050+4060) inches
= 7110 inches
Average heights
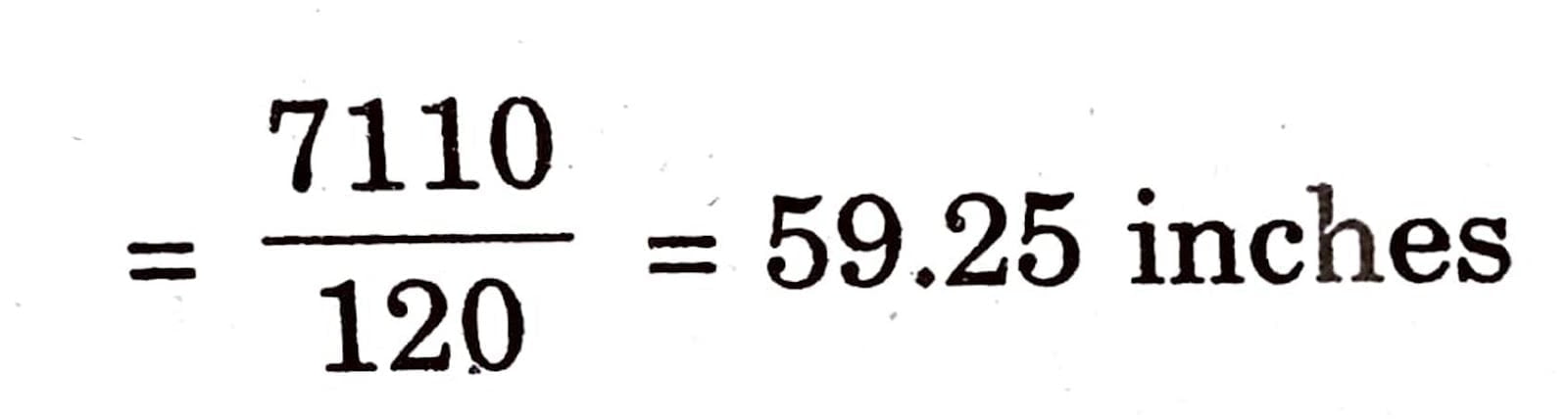
Q.6. Average income of 50 families is ₹ 3000. Out of these families, average income of 12 families is ₹ 1800. Calculate average income of remaining families.
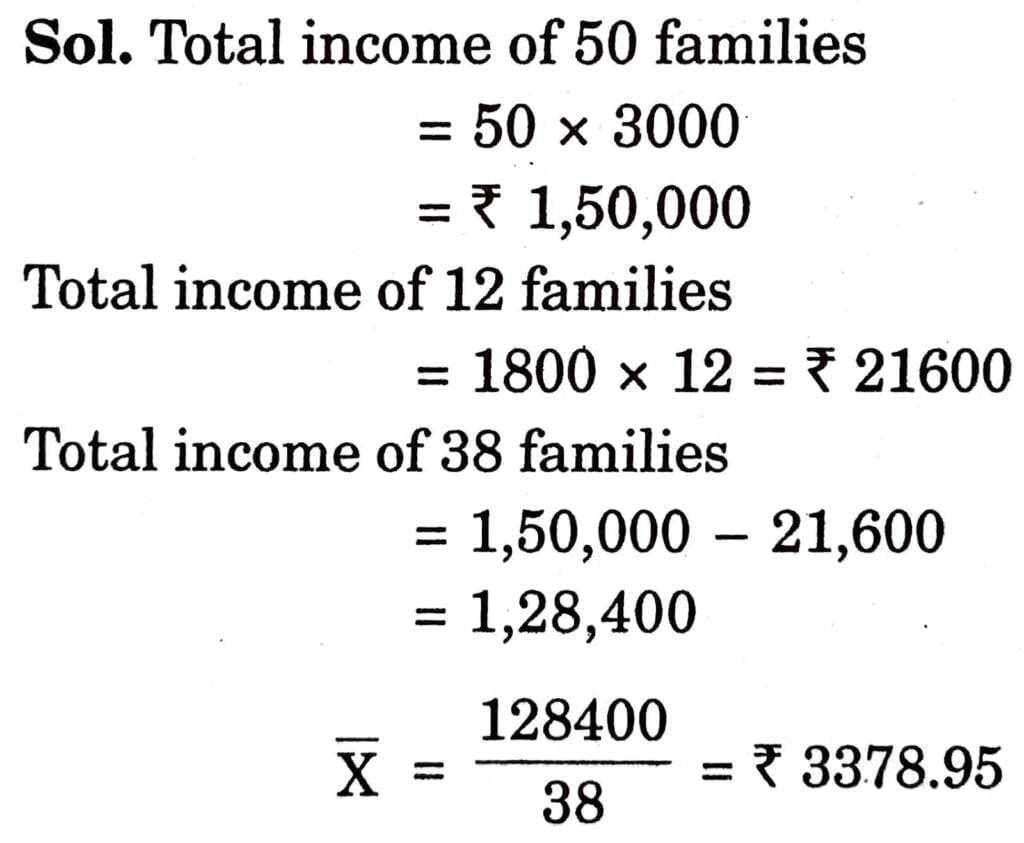
Q.7. Daily wages of 10 workers in a factory are given below. Calculate their arithmetic mean.
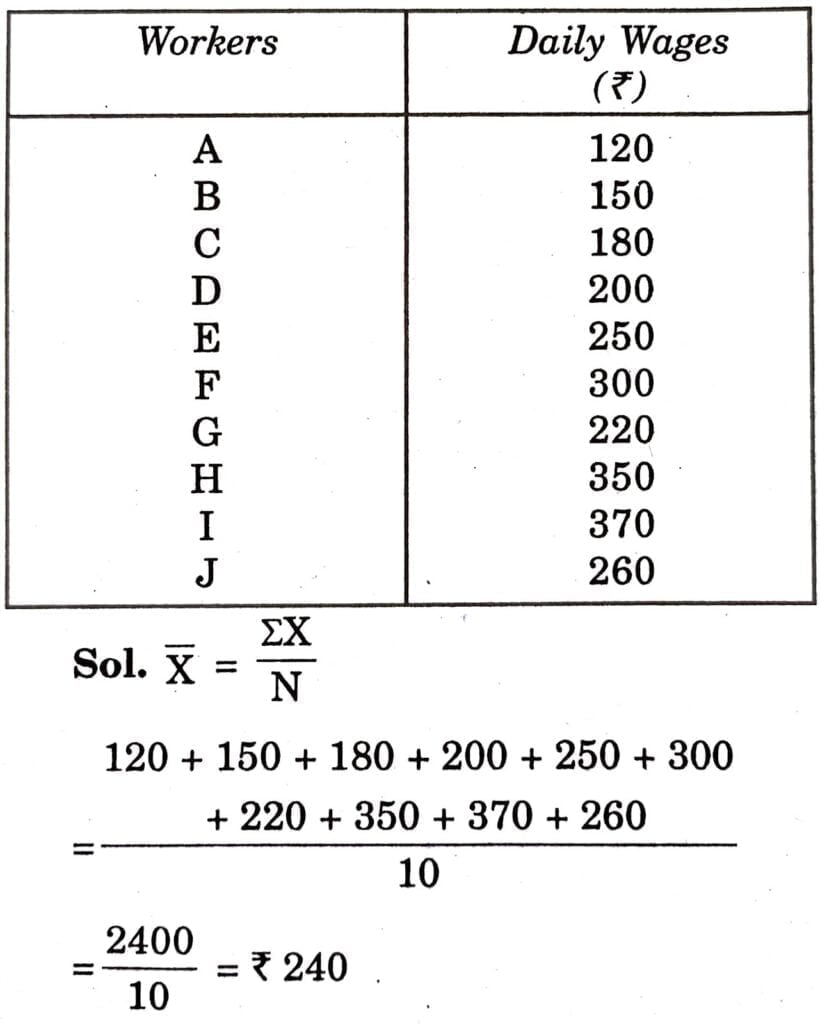
LONG ANSWER TYPE QUESTIONS
Q.1. Calculate arithmetic mean of the following distribution:
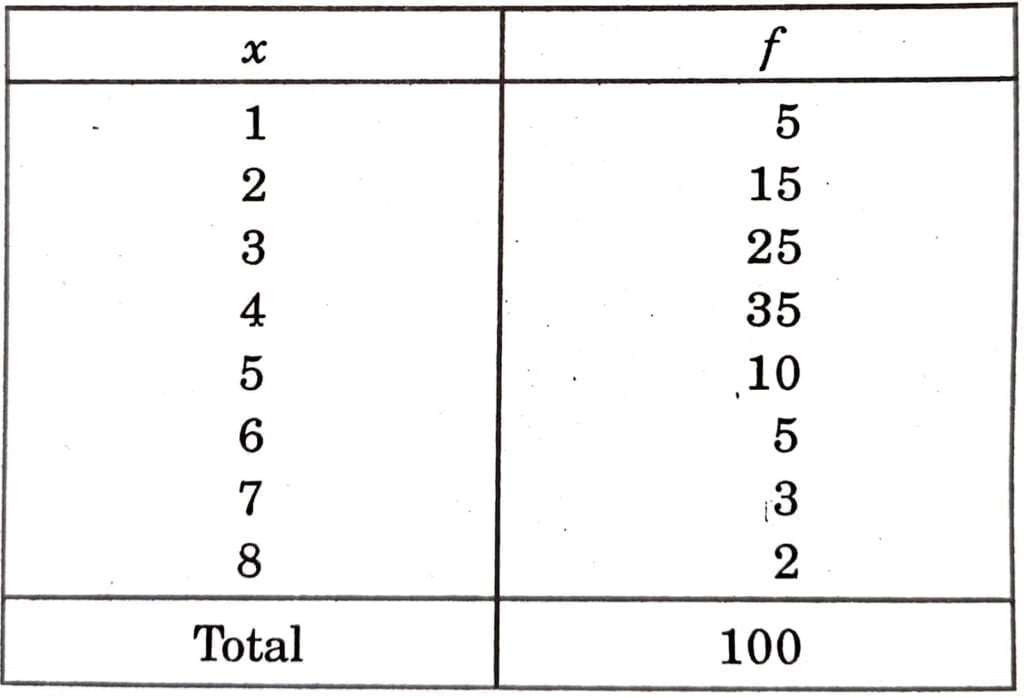
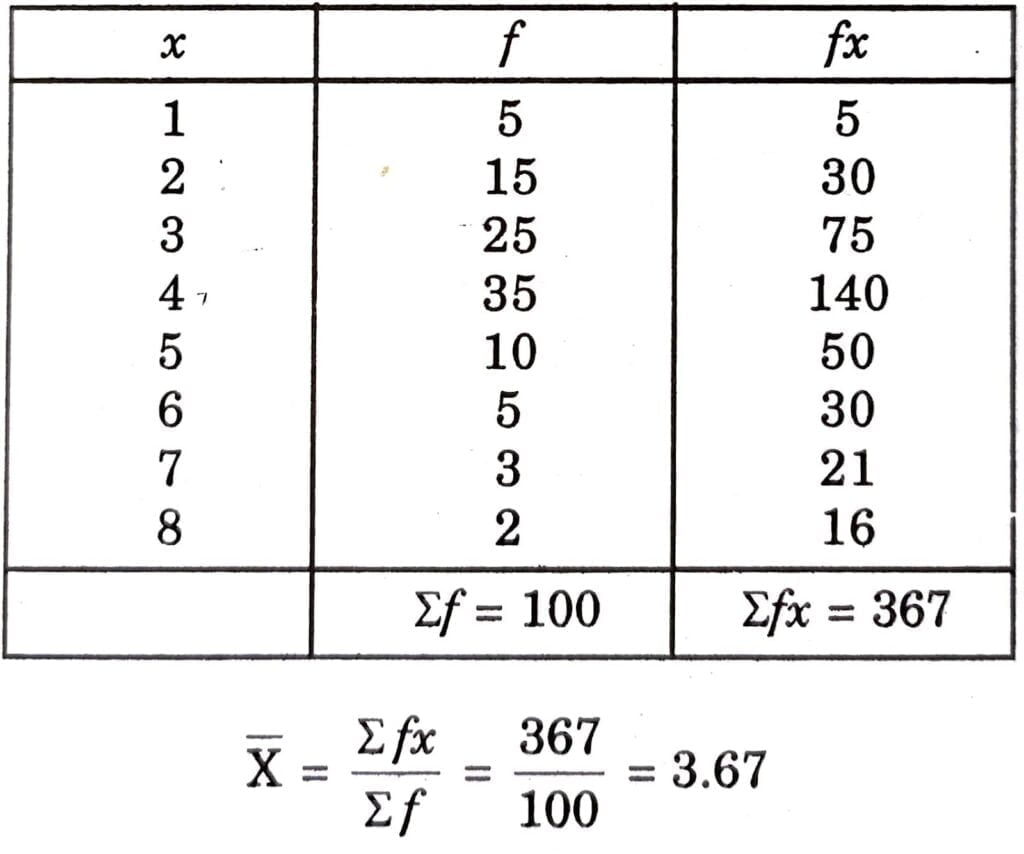
Ans.
Q.2. Find the mean of the following data by direct method, step deviation and assumed mean methods:
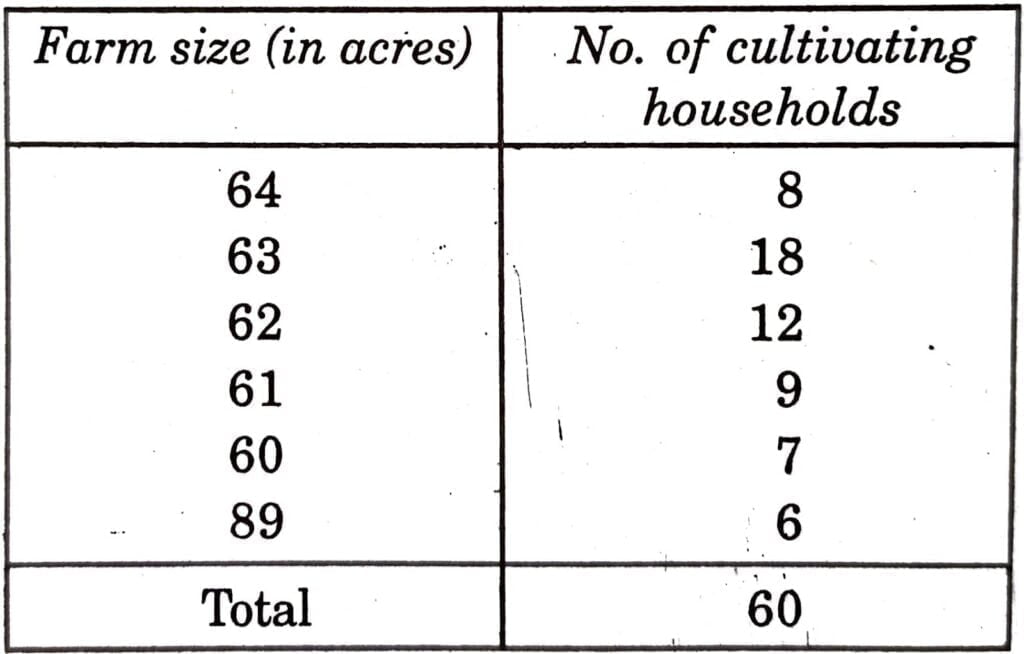
Ans. Arithmetic mean by Direct Method
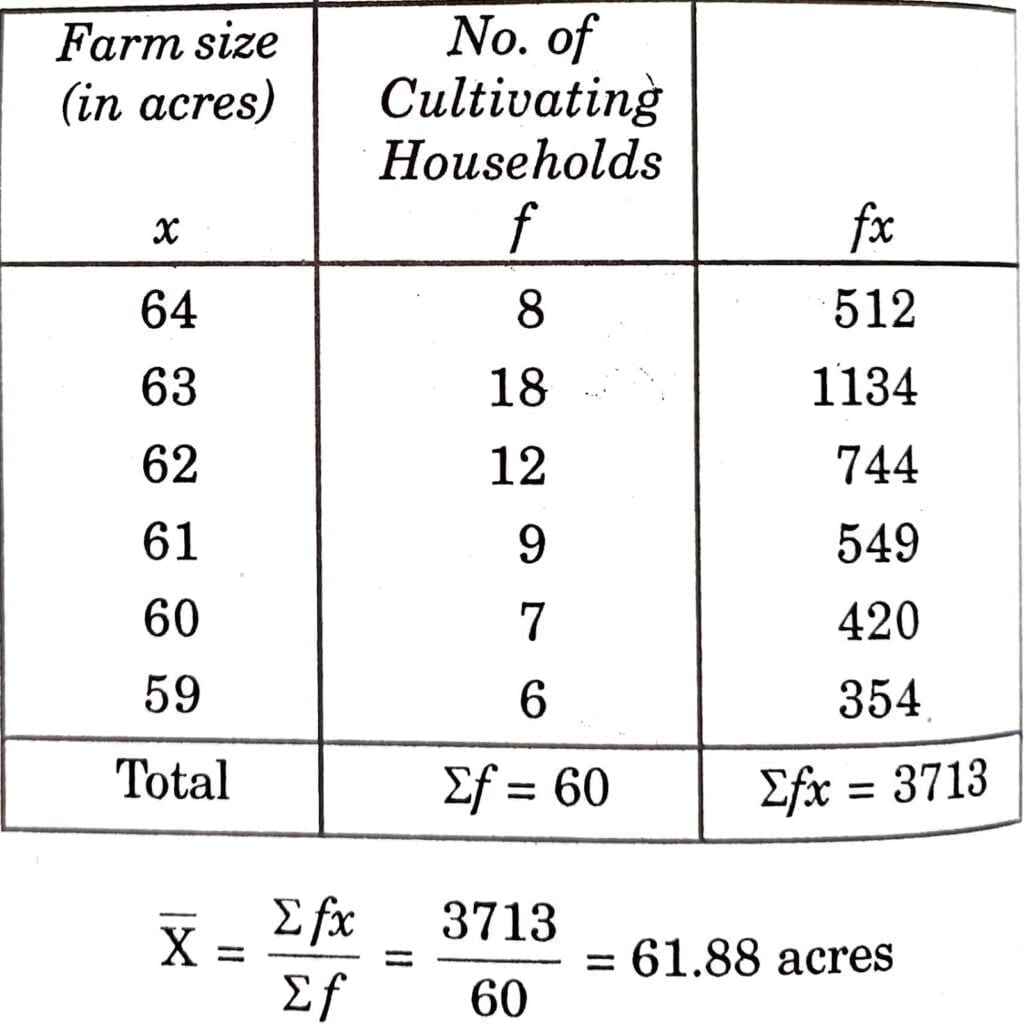
Calculation of Arithmetic Mean by Assumed Mean
