NIOS Class 12 Economics Chapter 8 Measures of Central Tendency, Solutions to each chapter is provided in the list so that you can easily browse through different chapters NIOS Class 12 Economics Chapter 8 Measures of Central Tendency and select need one. NIOS Class 12 Economics Chapter 8 Measures of Central Tendency Question Answers Download PDF. NIOS Study Material of Class 12 Economics Notes Paper 318.
NIOS Class 12 Economics Chapter 8 Measures of Central Tendency
Also, you can read the NIOS book online in these sections Solutions by Expert Teachers as per National Institute of Open Schooling (NIOS) Book guidelines. These solutions are part of NIOS All Subject Solutions. Here we have given NIOS Class 12 Economics Chapter 8 Measures of Central Tendency, NIOS Senior Secondary Course Economics Solutions for All Chapters, You can practice these here.
Measures of Central Tendency
Chapter: 8
Module – IV Statistical Tools
TEXT BOOK QUESTIONS WITH ANSWERS
INTEXT QUESTIONS 8.1.
Q.1. A researcher has collected the following simple individual data:
5, 12, 6, 8, 5, 6, 7, 5, 12, 4.
The mean of the data is:
(a) 5
(b) b
(c) 7
(d) 8
Ans. (c) Mean of the data = 5 + 12 + 6 + 8 + 5 + 6 + 7 + 5 + 12 + 4/ 10 = 70/10 = 7
Q.2. Find the mean of the set of number below:
3, 4, -1, 22, 14, 0, 9, 18, 7, 0, 1
Ans. Mean = 3 + 4 – 1 + 22 + 14 + 0 + 9 + 18 + 7 + 0 + 1/11 = 77/11 = 7
INTEXT QUESTIONS 8.2.
Q.1. Find the mean of the set of ages in the table below:
| Age (Years) | 10 | 11 | 12 | 13 | 14 |
| Frequency | 0 | 8 | 3 | 2 | 7 |
Ans.
| Age (Years) x | Frequency (f) | fx |
| 10 | 0 | 0 |
| 11 | 8 | 88 |
| 12 | 3 | 36 |
| 13 | 2 | 26 |
| 14 | 7 | 98 |
| Total | 20 | 248 |
Mean = Σf(x)/Σf = 248/20 = 12.4
Q.2. Find the mean average weekly earnings for the data given in example 3, by using step deviation method.
Ans.
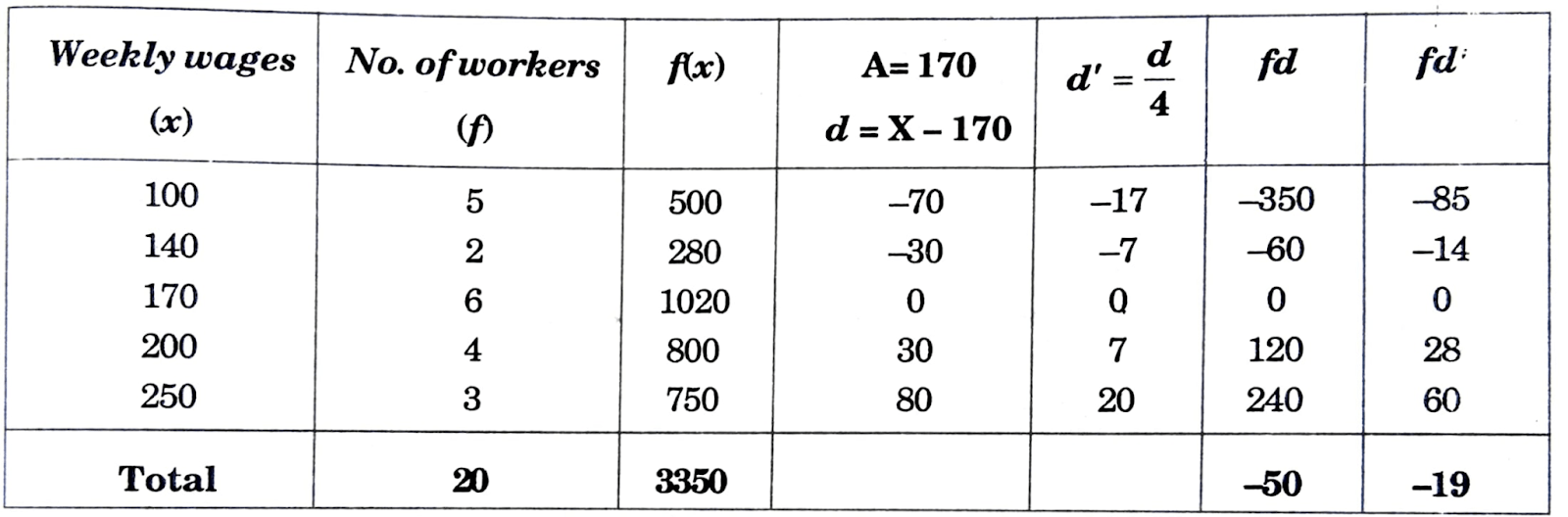
Step deviation method,
X̅ = A + Σfd/Σf × C
= 170 + -19/ 20 × 4 = +170 + (-19/ 5)
= 850 – 19/12 = 831/12 = 68.2
INTEXT QUESTIONS 8.3.
Q.1. The following distribution gives the pattern of overtime worker month done by 180 employees of a company. Calculate the arithmetic mean.
| Overtime (in hrs.) | 0-10 | 10-30 | 30-40 | 40-50 | 50-60 |
| No. of employees | 10 | 60 | 50 | 40 | 20 |
Ans.
| Overtime (in hrs.) | No. of employees (f) | Mid point (M) | fm | d’=m-45/10 | fd’ |
| 0-10 | 10 | 5 | 50 | -4 | -40 |
| 10-30 | 60 | 20 | 1200 | -2 | -120 |
| 30-40 | 50 | 35 | 1750 | -1 | -50 |
| 40-50 | 40 | 45 | 1800 | 0 | 0 |
| 50-60 | 20 | 55 | 1100 | 1 | 20 |
| Total | 180 | -190 |
X̅ = A + Σfd’/Σf × C
= 45 + (-190)/ 180 × 10 = 45 – 190/ 180
= 45 + 10 = 55.
Q. 2. A company is planning to improve plant safety. For this accident data for the last 180 weeks were complied. These data are grouped into the frequency distribution as shown below:
| No. of accidents | 1-10 | 11-20 | 21-30 | 31-40 | 41-50 | 51-60 |
| No. of weeks | 10 | 20 | 30 | 50 | 40 | 30 |
Calculate the arithmetic mean of the number of accident per day.
Ans.
| No. of accidents (in hrs.) | No. of weeks (f) | Mid point (M) | fm | d’=m-45.5/10 | fd’ |
| 1-10 | 10 | 5.5 | 55 | -4 | -40 |
| 11-20 | 20 | 15.5 | 310 | -3 | -60 |
| 21-30 | 30 | 25.5 | 765 | -2 | -60 |
| 31-40 | 50 | 35.5 | 1775 | -1 | -50 |
| 41-50 | 40 | 45.5 | 1820 | 0 | 0 |
| 51-60 | 30 | 55.5 | 1665 | 1 | 30 |
| Total | 180 | -180 |
X̅ = A + Σfd/Σf × C = 45.5 + -180/180 ×10
5.5 – 1 x 10 = 45.5 – 10
35.5 accidents per week.
INTEXT QUESTIONS 8.4.
Choose the correct answer:
Q.1. Sum of deviations of the individual data elements from their mean is:
(a) Always greater than zero.
(b) Always less than zero.
(c) Sometimes greater than and sometimes less than zero, depending on the data elements.
(d) Always equal to zero.
Ans. (d) Always equal to zero.
Q.2. In a group of 12 scores, the largest score is increased by 36 points. What effect will this have on the mean of the scores?
(a) It will be increased by 12 points.
(b) It will remain unchanged.
(c) It will be increased by 3 points.
(d) It will increase by 36 points.
(e) There is no way of knowing exactly how many points the mean will be increased.
Ans. (c) It will be increased by 3 points.
INTEXT QUESTIONS 8.5.
Q.1. The mean of a certain number of observations is 40. If two or more items with values 50 and 64 are added to this data, the mean rises to 42. Find the number of items in the original data.
Ans. Let the number of items in the original data = x
Mean = 40
Thus, according to questions
40x + 50+ 64/ X × 2 = 42
= 40 x + 50 + 64 = 42x + 84
= 114 – 84 = 42x – 40x
= 30 = 2x
= x = 30/2 = 15
number of items in original data is 15.
Q.2. Eight coins were tossed together and the number of times they fell on the side of heads was observed. The activity was performed 256 times and the frequency obtained for different values of x, (the number of times it fell on heads) is shown in the following table. Calculate then mean by:
(i) Direct method.
(ii) Short-cut method.
| x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| f | 1 | 9 | 26 | 59 | 72 | 52 | 29 | 7 | 1 |
Ans. (i) Direct method:
| x | f | fx |
| 0 | 1 | 0 |
| 1 | 9 | 9 |
| 2 | 26 | 52 |
| 3 | 59 | 177 |
| 4 | 72 | 288 |
| 5 | 52 | 260 |
| 6 | 29 | 174 |
| 7 | 7 | 49 |
| 8 | 1 | 8 |
| Total | 256 | 1017 |
X̅ = Σfx/Σf = 1017/256 = 3.97
(ii) Short cut method.
Q.3. Calculate the average age of employees working in a company from the following data:
| Age (years) below | 25 | 30 | 35 | 40 | 45 | 50 | 55 | 60 |
| No. of employees | 8 | 23 | 51 | 81 | 103 | 113 | 117 | 120 |
Ans. Given, X₁ = 25, X₂ = 30, X₃ = 35, X₄ = 40,
X₅ = 45, X₆ = 50, X₇ = 55, X₈ = 60
N₁ = 8, N₂ = 23, N₃ = 51, N₄ = 81,
N₅ = 103, N₆ = 113, N₇ = 117, N₈ = 120
X̅₁₂₃₄₅₆₇₈ = X₁N₁ + X₂N₂ + X₃N₃ + X₄N₄ + X₅N₅ + X₆N₆ + X₇N₇ + X₈N₈ / N₁ + N₂ + N₃ + N₄ + N₅
= 25 x 8 + 30 x 23 + 35 x 51 +40 x 81 + 45 x 103 + 50 x 113 + 55 x 117 + 60 × 120 / 8 + 23 + 51 + 81 + 103 + 113 + 117 + 120
= 200 + 690 + 1785 + 3240 + 4635 + 5650 + 6435 + 7200 / 616
= 29835 /616
= 48.43
INTEXT QUESTIONS 8.6.
Q.1. A big mall wants to know the weighted mean of the sales price of 2,000 units of one product that had its final price adjusted according to the first ten days of sales. The table below summarizes the relation between final price and number of sold units.
| Price per unit | No. of sold units | Price per unit | No. of sold units |
| ₹ 24.20 | 354 | ₹ 24.14 | 288 |
| ₹ 24.10 | 258 | ₹ 24.06 | 240 |
| ₹ 24.00 | 209 | ₹ 23.95 | 186 |
| ₹ 23.90 | 133 | ₹ 23.84 | 121 |
| ₹ 23.82 | 110 | ₹ 23.75 | 101 |
Compute both the average price and the weighted average sales price of this product.
Ans.
| Price per unit | No. of sold units | Price per unit | No. of sold units |
| ₹ 24.20 | 354 | ₹ 24.14 | 288 |
| ₹ 24.10 | 258 | ₹ 24.06 | 240 |
| ₹ 24.00 | 209 | ₹ 23.95 | 186 |
| ₹ 23.90 | 133 | ₹ 23.84 | 121 |
| ₹ 23.82 | 110 | ₹ 23.75 | 101 |
Average price = 24.20 + 24.10 + 24.00 + 23.90 + 23.82 + 24.14 + 24.06 + 23.95 + 23.84 + 23.75 /10
= 239.76 / 10 = 23.976
Weighted over average sales price = ΣWX/ΣW
= 24.20 × 354 + 24.10 x 258 + 24.00 x 209 + 23.90 x 133 + 23.82 × 110 + 24.14 x 288 + 24.06 x 240 +23.95 x 186 +23.84 x 121 + 23.75 × 101 / 354 +258 + 209 + 133 + 110 + 288 +240 + 186 + 121 + 101
= 8566.80 + 6217.80 + 5016 + 3178.70 + 2620.20 + 6952.32 + 5774.40 + 4454.70 + 2884.64 +2398.75 / 2000
= 48064 / 2000
= 24.032
∴ Average price is 24.032
INTEXT QUESTIONS 8.7.
Q.1. If a data set has an even number of observations, the median
(a) cannot be determined.
(b) is the average value of the two middle items.
(c) must be equal to the mean.
(d) is the average value of the two middle items when all items are arranged in ascending order.
Ans. (d) is the average value of the two middle items when all items are arranged in ascending order.
Q.2. A distribution of 6 scores has a median of 21. If the highest score increases 3 points, the median will become:
(a) 21
(b) 21.5
(c) 24
(d) cannot be determined without additional information.
(e) none of these.
Ans. (a) 21
INTEXT QUESTIONS 8.8.
Q.1. Calculate the median age of the persons from the following data:
| Age (years): | 20-25 | 25-30 | 30-35 | 35-40 | 40-45 |
| No. of person: | 70 | 80 | 180 | 150 | 20 |
Ans.
| Age | No. of person (f) | Cumulative frequency (cf) |
| 20-25 | 70 | 70 |
| 25-30 | 80 | 70+80=150 |
| 30-35 | 180 | 150+180=330 |
| 35-40 | 150 | 330+150=480 |
| 40-45 | 20 | 480+20=500 |
Median = N/2th item = 500/2 = 250
The median class is 30-35.
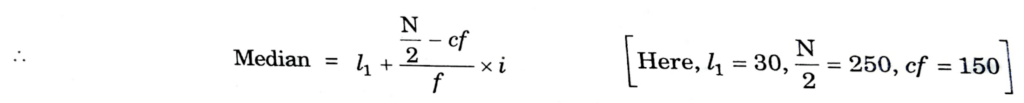
= 30 + 250 – 150/180 × 5 = 30 + 100/180 × 5 = 30 +25/9
= 30 + 2.7 = 32.7
Q. 2. Calculate the median marks of the students:
| Marks | 40-50 | 30-40 | 20-30 | 10-20 | 0-10 |
| No. of students | 10 | 12 | 40 | 30 | 8 |
Ans.
| Marks | No. of Students (f) | Cumulative frequency (cf) |
| 40-50 | 10 | 10 |
| 30-40 | 12 | 10+12=22 |
| 20-30 | 40 | 22+40=62 |
| 10-20 | 30 | 62+30=92 |
| 0-10 | 8 | 92+8=100 |
Median = N/2 = 100/2 = 50
The median class is 20-30.
∴ Median = l₁ + N/2 – cf/f × i
= 20 + 50 – 22/40 × 10 [Here, l₁ = 20, N/2 = 50, cf = 22]
= 20 + 28/4
= 20 + 7 = 27
INTEXT QUESTIONS 8.9
Q.1. The most frequently occurring value of data set is called:
(a) range.
(b) mode.
(c) mean.
(d) median.
Ans. (b) Mode.
Q.2. Find the mode of 12, 15, 18, 26, 15, 9, 12, 27.
Ans. The mode are 12 and 15. Since it occurs two times and the other values occur only once.
Q. 3. The measure of location which is the most likely to be influenced by extreme values in the data set is the:
(a) median.
(b) mode.
(c) mean.
(d) quartile.
Ans. (c) Mean.
Q.4. A researcher has collected the following sample individual data:
| 5 | 12 | 6 | 8 | 5 | 6 | 7 | 5 | 12 | 4 |
The median is:
(a) 5.
(b) 6.
(c) 7.
(d) 8.
Ans: Median is (b) 6.
And the Mode is:
(a) 5.
(b) 6.
(c) 7.
(d) 8.
Ans. Mode is (b) 6.
Q.5. Which of the following can have more than one value?
(a) Median.
(b) Quartile.
(c) mode. and
(d) mean.
Ans. (c) Mode.
TERMINAL EXERCISE
MEAN
Q.1. An average daily wages of all 90 workers in a factory is 60. An average daily wages of non-technical workers is 45. Calculate an average daily wages of technical workers if one-third workers are technical.
Ans. Average daily wages of 90 workers = ₹ 60
Average wages of non-technical workers = ₹ 45
1/3rd are technical workers = 60
Total wages = 90 x 60 = 5400
Total wages of non-technical workers = 60 x 45 = 2700
Total wages of technical workers = 5400 – 2700 = 2700
So, an average daily wages of technical workers = 2700/ 30 = 90
Q.2. For the two frequency distribution given below, the mean calculated from the first was 25.4 and that from the second was 32.5. Find the values of x and y.
| Class Interval | Distribution I | Distribution II |
| 10-20 | 20 | 4 |
| 20-30 | 15 | 8 |
| 30-40 | 10 | 4 |
| 40-50 | x | 2x |
| 50-60 | y | y |
Sol. In distribution I:
| Class Interval | Distribution I | Mid point (M) | fm |
| 10-20 | 20 | 15 | 300 |
| 20-30 | 15 | 25 | 375 |
| 30-40 | 10 | 35 | 350 |
| 40-50 | x | 45 | 45x |
| 50-60 | y | 55 | 55y |
Thus, 300 + 375 +350 + 45 x +55 y/ 45 + x + y = 25.4
= 1025 + 45 x + 55 y/ 45 + x + y = 25.4
= 1025 + 45x + 55y = 25.4 (45 + x + y)
= 1025 + 45x + 55y = 1143 +25.4x + 25.4y
= 45x – 25.4x + 55y-25.4y = 1143 – 1025
= 19.6x + 29.6y = 118
In distribution II:
| Class Interval | Distribution I | Mid point (M) | fm |
| 10-20 | 20 | 15 | 60 |
| 20-30 | 15 | 25 | 200 |
| 30-40 | 10 | 35 | 140 |
| 40-50 | x | 45 | 90x |
| 50-60 | y | 55 | 55y |
| 16+2x+y | 400+90x+55y |
Thus, 32.5 = 400 + 90 x + 55 y/ 16 + 2x + y
= 520 + 65x + 32.5y = 400 + 90x + 55y
= 520 – 400 = 90 x – 65 x + 55 y – 32.5y
= 120 = 25x + 22.5y
From (i) and (ii)
19.6x + 29.6y = 118
25x + 22.5y = 120
Equation (i) multiply 25 and eq.
(ii) multiply by 19.6.
490x + 740y = 2950
490x + 441y = 2352
299y = 598
y = 598/ 299 = 2
Put the value of y in equation (i)
19.6x + 29.6 (2) = 118
= 19.6x = 118 – 59.2 = 58.8
x = 58.8/19.6 = 3
Thus, x = 3 and y = 2.
Q.3. The mean of 99 items is 55. The value of 100th items is 99 more than the mean of 100 items. What is the value of 100th item?
Ans. If X is the mean of 100 items and Y + 99 is the value of 100 item.
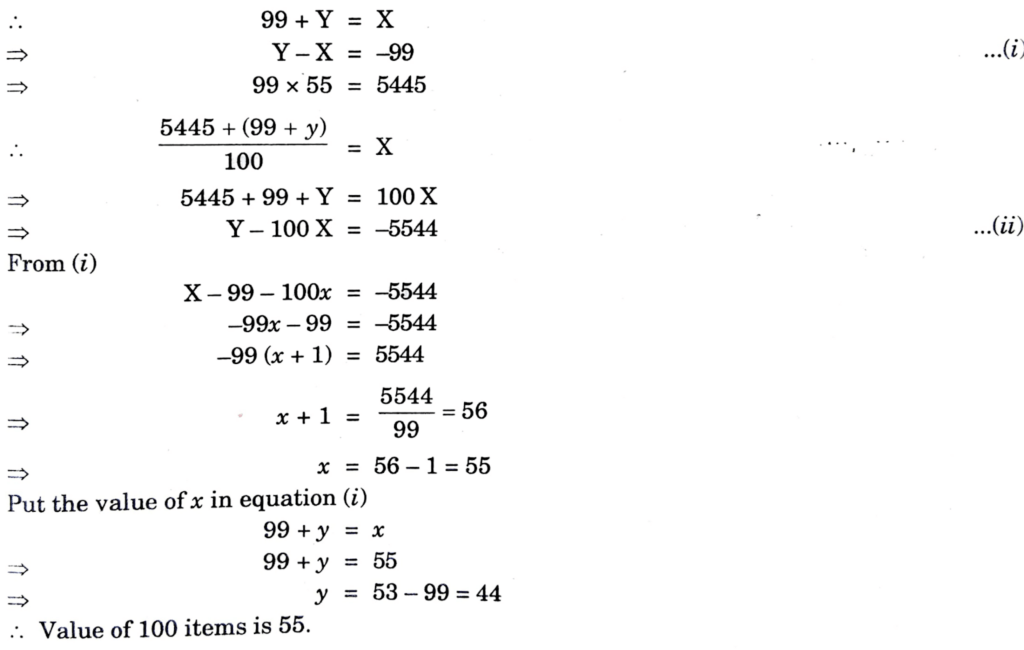
Q.4. The length of time taken by each of 18 workers to complete a specific job was observed to be the following:
| Time (in min) | 5-9 | 10-14 | 15-19 | 20-24 | 25-29 |
| No. of workers | 3 | 8 | 4 | 2 | 1 |
Calculate the median time and Q₁ and Q₃
Ans.
| Time | No. of workers | Cumulative frequency |
| 5-9 | 3 | 3 |
| 10-14 | 8 | 3+8=11 |
| 15-19 | 4 | 11+4=15 |
| 20-24 | 2 | 15+2=17 |
| 25-29 | 1 | 17+1=18 |
Here,
M = N/2 = 18/2 = 9
The median class is 10-14
Median = l1 + N/2 – cf/ f x i [Here, l2 = 10, N/2 = 9, cf = 3, f = 8]
= 10 + 9-3/18 x 4 = 10 + 1.3 = 11.3
Now, Q1 and Q3 will be
Here, N = 18, N/4 = 18/4 = 4.5
∴ Q1 lies between 10 – 14
Q1 = l1 + N/4 -cf/ f x i = 10 + 4.5 – 3/18 x 10
= 10 + 1.5/18 x 10 = -10 + 0.875 = 10.87
and Q₃ = Size of 3 (N+1)/4 = 3 (18+1)/4 = 57/4 = 14.25
Q₃ lies between 15 – 19
Q₃ = l₂ + 3 (N+1)/4 – cf/f × i
= 15 + 14.25 – 11/ 18 x 10 = 15 + 3.25/18 × 10
= 15 + 1.805 = 16.805
Q. 5. Calculate the median from the following data:
| Mid values | 115 | 125 | 135 | 145 | 155 | 165 | 175 | 185 | 195 |
| Frequency | 6 | 25 | 48 | 72 | 116 | 60 | 38 | 22 | 3 |
Ans.
| Class interval | Mid value | Frequency | Cumulative frequency (cf) |
| 100-120 | 115 | 6 | 6 |
| 120-130 | 125 | 25 | 6+25=31 |
| 130-140 | 135 | 48 | 31+48=79 |
| 140-150 | 145 | 72 | 79+72=151 |
| 150-160 | 155 | 116 | 151+116=267 |
| 160-170 | 165 | 60 | 267+60=327 |
| 170-180 | 175 | 38 | 327+38=365 |
| 180-190 | 185 | 22 | 365+22=387 |
| 190-200 | 195 | 3 | 387+3=390 |
Median = N/2 = 390/2 = 195
195 lies between 150-160
M = l₁ +N/2-cf/fxi= 150 + 195 – 151/116 x 10
= 150 + 440/116 = 150 + 3.79 = 153.79
Q.6. If the quartiles of the following distribution are Q₁ = 23.125 and Q₃ = 43.5, find the median:
| Daily Wages | 1-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 |
| No. of workers | 5 | – | 20 | 30 | – | 10 |
Ans.
| Daily Wages | No. of workers | Cumulative frequency |
| 0-10 | 5 | 5 |
| 10-20 | x | 5+x |
| 20-30 | 20 | 25+x |
| 30-40 | 30 | 55+x |
| 40-50 | y | 55+x+y |
| 50-60 | 10 | 65+x+y |
Here, Q₁ = 23.125 and Q₃ = 43.5
Median = l₂ + N/2 – cf/ f × i
Q₁ = N + 1/4 = 23.125
= N + 1 = 4 × 23.125
= N + 1 = 92.5
Q₃ = 3 (N+1)/4 = 43.5
= 3 (N+1) = 43.5 × 4 = 174
= 3N + 3 = 174
From (i) and (ii)
3N + 3 – N – 1 = 174 – 92.5
= 2N + 2 = 81.5
= 2N = 81.5 – 2 = 79.5
= N = 79.5/2 = 39.75
= N = 39.75
Q.7. The mean and median of a group of 25 observations are 143, 144 and 147 respectively. A set of 6 observations is added to this data with values 132, 125, 130, 160, 165 and 157. Find mean and median for the combined group of 31 observations.
Ans. Mean of 25 observations = 143
6 observation are added: 132 + 125 + 130 + 160 + 165 + 157 = 869
Mean of 1 observation = 25 x 143 = 3575
Mean = 3575 + 869 / 31= 143.35
New mean will be 143.35
Since
Median = N + 1/ 2
New,
median will be = 31+1/ 2 = 32/2 = 16
In 25 observations: 144 (12+ 4 portion) and 147 (13th portion).
In 31 observation: 125, 130, 132 will come before (144) and 157, 160, 165 will come later 147. Thus 147th will be at the 16th portion that mean, new median will be 147.
Q.8. Locate mode in the class data:
7, 12, 8, 5, 9, 6, 10, 9, 4, 9, 9
Ans. Arrange the series in ascending order
4, 5, 6, 7, 8, 9, 9, 9, 9, 10, 12
The mode is 9 since it occurs four times and the other values occur only once.
Q.9. Determine the modal value in the following series:
| Values | 10 | 12 | 24 | 16 | 18 | 20 | 22 | 24 | 26 | 28 | 30 | 32 |
| Frequency | 7 | 15 | 21 | 38 | 34 | 34 | 11 | 19 | 10 | 38 | 5 | 2 |
Ans. From the above data one can clearly see that modal value is 16 and 18.
Q.10. The median and mode of the following wage distribution are known to be ₹ 33.5 and ₹ 34 respectively. Three frequency values from the table are however Find the missing values.
| Wages | Frequency |
| 0-10 | 10 |
| 10-20 | 10 |
| 20-30 | ? |
| 30-40 | ? |
| 40-50 | ? |
| 50-60 | 6 |
| 60-70 | 4 |
| 230 |
Ans.
| Wages | Frequency | Cumulative frequency |
| 0-10 | 10 | 10 |
| 10-20 | 10 | 20 |
| 20-30 | ? | 20+x |
| 30-40 | ? | 20+x+y |
| 40-50 | ? | 220 |
| 50-60 | 6 | 226 |
| 60-70 | 4 | 230 |
| 230 |
Median is 33.5
Q.11. The details of monthly salary of various categories of employees working in a university are given below. From these details, calculate mode of monthly salary.
| Various category | Monthly salary | No. of employee |
| Principal | 10,00,000 | 1 |
| Vice Principal | 2,50,000 | 1 |
| Senior | 75,000 | 5 |
| Professor Professor | 30,000 | 8 |
| Associate Professor | 20,000 | 13 |
| Assistant Professor | 18,000 | 9 |
Sol. The mode is in 13.
Q.12. The distribution of age of patients a turned out in a hospital on a particular day was as under:
| Age (in years) | No. of patients |
| More than 10 | 148 |
| More than 20 | 124 |
| More than 30 | 109 |
| More than 40 | 71 |
| More than 50 | 30 |
| More than 60 | 16 |
| More than 70 and up to 80 | 1 |
Find the median age and modal age of the patients.
Ans.
| Age (in years) | No. of patients | Cumulative frequency |
| More than 10 | 148 | 148 |
| More than 20 | 124 | 148+124=272 |
| More than 30 | 109 | 272+109=381 |
| More than 40 | 71 | 381+71=452 |
| More than 50 | 30 | 452+30=482 |
| More than 60 | 16 | 482+16=498 |
| More than 70 and up to 80 | 1 | 498+1=499 |
N = 499
Median = 499 / 2 = 249.5 lies in 20-30
Median = l₁ + N / 2 – cf / f × i
= 20 + 249.5 – 148 / 124 × 10
= 20 + 1015 / 124 = 20 + 8.18 = 28.18
Mode = l₁ + f₁ – f₀ / 2f₁ – f₀ – f₂ × i [Median class lies in 20-30]
= 20 + 148 – 0 / 1(148) – 0 – 124 × 10
= 20 + 148 / 296 – 124 × 10
= 20 + 1480 / 172
= 20 + 8.604
= 28.604

Hi! my Name is Parimal Roy. I have completed my Bachelor’s degree in Philosophy (B.A.) from Silapathar General College. Currently, I am working as an HR Manager at Dev Library. It is a website that provides study materials for students from Class 3 to 12, including SCERT and NCERT notes. It also offers resources for BA, B.Com, B.Sc, and Computer Science, along with postgraduate notes. Besides study materials, the website has novels, eBooks, health and finance articles, biographies, quotes, and more.


