Class 12 Education Chapter 7 Educational Statistics answer to each chapter is provided in the list so that you can easily browse throughout different chapters SCERT Class 12 Education Chapter 7 Educational Statistics and select need one.
Class 12 Education Chapter 7 Educational Statistics
Also, you can read the SCERT book online in these sections Solutions by Expert Teachers as per SCERT (CBSE) Book guidelines. These solutions are part of SCERT All Subject Solutions. Here we have given Assam Board/NCERT Class 12 Education Chapter 7 Educational Statistics Solutions for All Subject, You can practice these here.
Educational Statistics
Chapter: 7
A. Questions for 1 Mark Each:
( a ) Fill in the blanks:
Q.1. …………… is the measure of central tendency which occurs most frequently.
Ans :- Mode.
Q.2. In a histogram class intervals are plotted on…………….. axis.
Ans :- Ox
Q.3. In a histogram the scores within a given interval are represented by ……………….. drawn on class intervals.
Ans :- Separate rectangle.
Q.4. The interval between the highest and lowest score is called ………………
Ans :- Range.
Q.5. ………………..is a bar graph with no gap in-between.
Ans :- Histogram.
Q.6. …………….. is essentially a line graph.
Ans :- Frequency Polygon .
Q.7. In assumed mean method AM is the …………… of any class interval.
Ans :- Midpoint.
Q.8. The three most commonly used measures of central and tendency are mean …………… and Mode.
Ans :- Median .
Q.9. 3 Mdn-2M = ………………..
Ans :- Mode.
Q.10. Mid value of the distance between the third and the first quartile is …………….
Ans :- Median.
Q.11. Range is a measure of ………………
Ans :- Variability or dispersion.
Q.12. Median is a score below which we can find…………….. % of score.
Ans :- 50%
Q.13. The extent of scatter of scores in the neighborhood of the average is called …………….
Ans :- Variability.
Q.14. The difference between the highest sore and lowest score of a frequency distribution is known as…………….
Ans :- Magnitude.
Q.15. The score below which we can find out half of the scores is the ……………
Ans :- Median.
Q.16. A histogram is a …………….
Ans :- Column diagram.
Q.17. In a polygon frequencies are plotted on………………
Ans :- Oy axis.
Q.18. The mode of distribution is the value that has the highest of ……………….
Ans :- The mode of distribution is the value that has the highest of series.
Q.19. In a histogram the area of each rectangle is proportional to ………………
Ans:- In a histogram the area of each rectangle is proportional to the number of measures with the interval.
Q.20. In a histogram, each class rectangles is constructed with base as………….
Ans :- In a histogram, each class rectangles is contracted with base as frequencies.
B. Write true or false:
Q.21. Mean can be computed by inspecting the scores.
Ans :- True.
Q.22. Medians are affected by extreme values.
Ans :- False.
Q.23. Mode is not affected by range of the scores.
Ans :- True.
Q.24. Median is the mid value of a class interval.
Ans :- True.
Q.25. Polygon is a line graph.
Ans :- True.
Q.26. Central tendency is a representative value of the scores.
Ans :- True.
Q.27. It is possible to compute median from open-ended distribution.
Ans :- True.
Q.28. In a polygon frequencies are plotted on x-axis.
Ans :- False.
Q.29. Mean is the most reliable measure of central tendency.
Ans :- True.
Q.30. Quartile deviation is a measure of variability.
Ans :- True.
Q.31. We can compute statistics from statistics by statistics.
Ans :- True.
Q.32. Range is a measure of central tendency.
Ans :- False.
Q.33. Median is a measure of variability.
Ans :- False.
Q.34. The gap between highest score and lowest score is called range.
Ans :- True.
Q.35. Median is sensitive to the extreme values of the distribution.
Ans :- False.
Q.36. The first quartile (Q₁) is the score blow which lie 75% of the scores.
Ans :- False.
Q.37. Median and Mode can be determined graphically.
Ans :- Median and mode can be determined graphically False.
Q.38. Quartile deviation is suitable for open-ended distribution.
Ans :- Quartile deviation is suitable for open ended distribution-False.
Q.39. Pie diagram is a circle broken down into components.
Ans :- Pie diagram is a circle broken down into components -True.
C. Give short answer:
Q.40. What is frequency?
Ans :- Frequency refers to the number times a particular value appears in a distribution. For example, in a distribution of values, 4, 5, 5, 6, 5, 4, the frequency of 5 is 3, that of 4 is 2 and that of 6 is 1.
Q.41. What do you mean by class interval?
Ans :- The size of the class interval is determined in view of the total number of values in a given distribution of data.
Q.42. What is the representational graph?
Ans :- It is a pictured representation of an event for which it bears reliability and objectivity. It can be done through frequency polygon, histogram, cumulative frequency graph and cumulative percentage curve or ogive.
Q.43. Write the formula for finding out Median from grouped data?
Ans :-
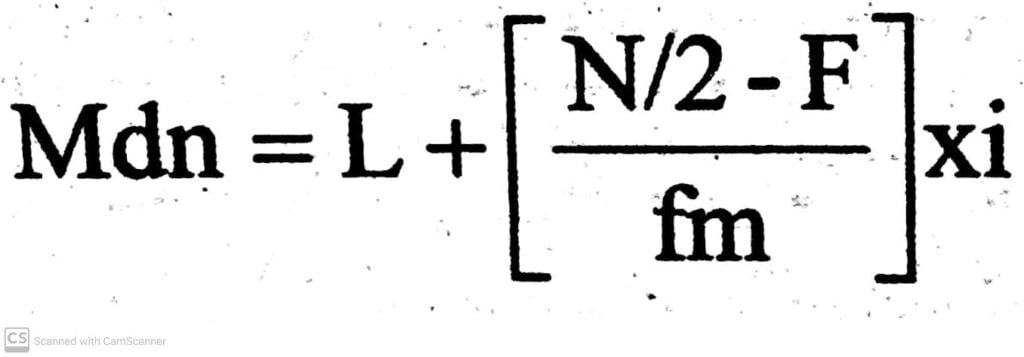
Q.44. Why is histogram called a continuous bar graph?
Ans :- Histogram is called a continuous bar graph because the frequency distribution of the class interval uniformly.
Q.45. What is a frequency distribution table?
Ans :- A frequency distribution table is a chart that summarizes values and their frequency. It’s a useful way to organize data if have a list of numbers that represent the frequency of a certain outcome in a sample. A frequency distribution table has two columns.
Q.46. What is pie diagram?
Ans :- When a circle is subdivided into various sectors for representing different data it is called a Pie-diagram. To represent the data by a pie-diagram we first draw a circle with any radius. The angle at the centre of the circle is of 360 degrees which represents the aggregate. It can be divided into a number of smaller angles whose degrees would be in proportion to the values of the components. The angle representing each item or sector may be calculated with the help of the following formula .
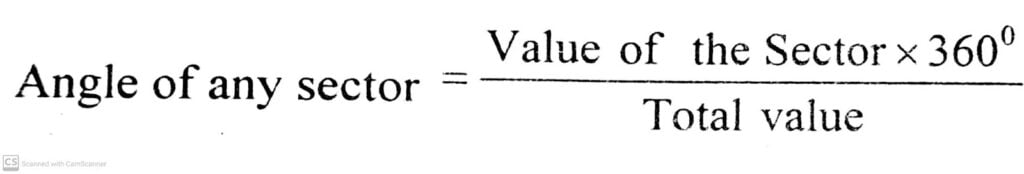
Suppose, in a college there are 120 students in Arts and 60 students in science faculty. If the values are represented by a pie-diagram

Q.47. What are the first quartile and third quartile?
Ans :- Q₁ is the 25th percentile above on 25 percent below the median on half the distance between Q₃ and Q₁. Q₃ is the 75th percentile or the third percentile.
Q.48. Which measure of central tendency will be applicable-when greatest stability is wanted?
Ans :- Mean.
Q.49. What is meant by the central tendency of a group of obtained scores?
Ans :- Quantitative data show a common characteristic to concentrate at certain values, usually somewhere in the centre of the distribution. So there is a typical value which can represent all the figures in a series. This value around which the values of a distribution tend to cluster is called a measure of central tendency. Arithmetic Mean is an important measure of central tendency.
Q.50. Given the following sets of scores. Calculate the range for each Set.
Set – I : 15, 12, 18, 22, 5, 27, 8, 35
Set – II : 45, 52, 33, 42, 23, 58, 70, 79, 56
Ans :- Set – I : 35 – 5 = 30
Set – II : 79 – 23 = 56
Q.51. Write two differences between polygon and histogram.
Ans :- Two differences between polygon and histogram are:-
i) Frequency polygon is an improvement over histogram because it provides a continuous curve indicating the causes of rise and fall in the data. On the other hand, frequency polygon is an approximate curve, but still it is more useful as compared to histogram.
ii) In the frequency polygon, it is assumed that the frequency distribution in a particular class-whereas histogram may be used to represent frequency distribution with equal as well as with unequal class width.
Q.52. Write two merits of mean.
Ans :- Two merits of mean are:-
i) It is based on all the observations.
ii) It is uniquely defined or it has one and only one value.
Q.53. Write two demerits of median.
Ans :- Two demerits of median:-
i) It is not based upon all the observations.
ii) It is affected much by sampling fluctuation.
Q.54. Which is the most unstable central tendency?
Ans :- The most unstable Central tendency is Mean.
Q.55. Which is the positional measure of central tendency?
Ans :- Median is the positional measure of central tendency.
Q.56. Which measure of Central tendency is most affected by extreme observation?
Ans :- The measure of central tendency affected most by extreme observation is median.
Q.57. From a batch of 13 students who had appeared for an examination 4 students had failed. The marks of the successful students were – 41, 57, 38, 61, 35, 39, 75, 50, 45. Calculate the Median.
Ans :- The formula of median is
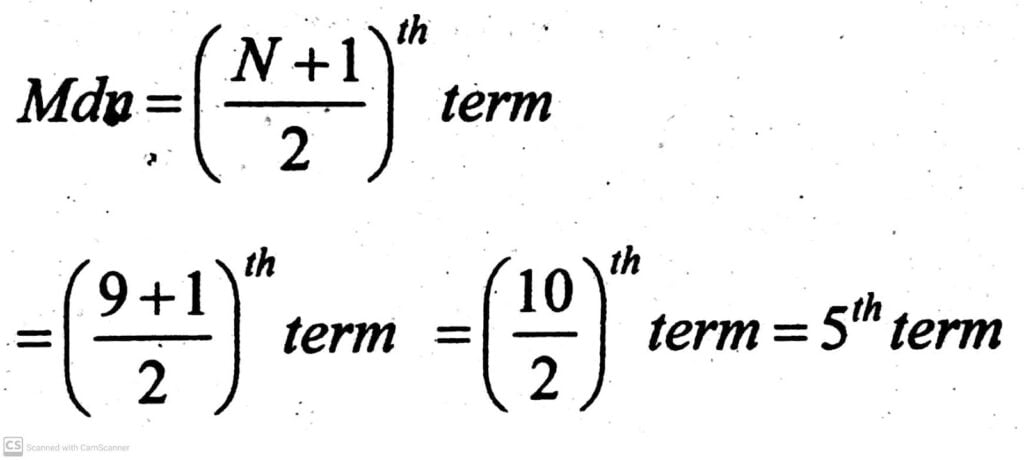
If the marks of the successful students are arranged in an ascending order, 35, 38, 39, 41, 45, 50, 57, 61, 75
Mdn = 5ᵗʰ term = 45
Mdn = 45
Q.58. In a histogram the class intervals are taken along – X – Axis/ Y – Axis.
Ans :- Ina Histogram the class intervals are taken along x-Axis (xAxis/ y-Axis)
Q.59. Given Mean is 25, Mode is 24, the Median would be ……………
Ans :- Given Mean is 25, Mode is 24, the median would be 24.6
[Mode= (3Mdn- 2m)]
⇒ 24 = ( 3 × Mdn – 2 × 25 )
⇒ 24 = 3 Mdn – 50
⇒ 3Mdn – 50 = 24
⇒ 3Mdn = 24 + 50
⇒ Mdn = 24 + 50/2
= 73/3
= 24.6
Q.60. The mean of marks scored by 100 students was found to be 40. Later on it was discovered that a score of 53 was misread as 63. Find the correct meaning.
Ans :- The correct mean is 58.
Q.61. The mean of five numbers is 27. If one number is excluded, their mean is 25. Find the excluded number.
Ans :- The excluded number is 125
M = ∑x/N
⇒ 24 = ∑x/5
⇒ x = 125
Q.62. Find the Median of the following data : 41, 43, 125, 99, 61, 92, 71, 58, 57. If 58 is replaced by 85. What will be the new median?
Ans :- The given data are arranged in an ascending order, 41, 43, 57, 61, 71, 85, 92, 99, 125
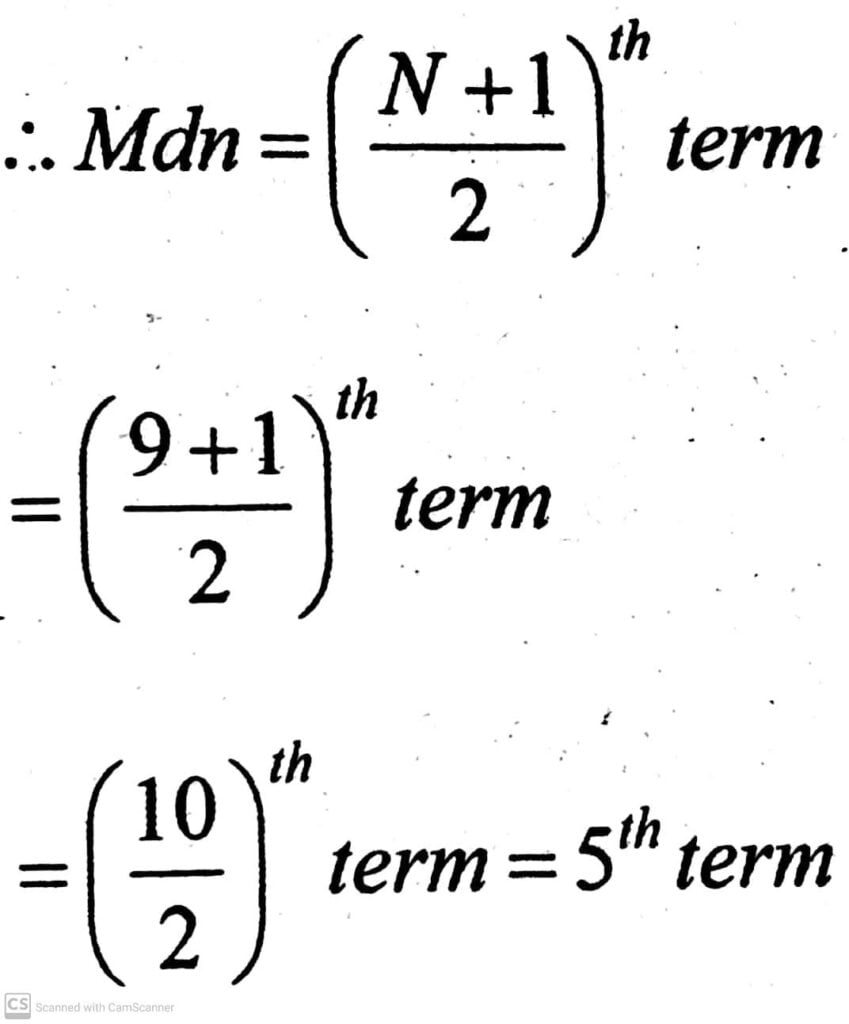
Mdn = 5ᵗʰ term = 71
The new median is 71 .
D. Questions for 3 Mark Each:
Q.63. What are the different measures of central tendency? Give examples.
Ans :- The different measures of central tendency are :-
Mean:- Mean is the best known and the most commonly used measure of central tendency. It is equal to the sum of all the scores divided by the total number of scores.
The formula used for calculating Mean by short method from grouped data is –
Mean = AM + ∑fx/N × 1
Where AM = Assumed Mean
x’ = Deviation of x from the AM which is obtained by using
X-AM/1
f = frequency in the class-intervals
N = Total frequency
i = Span of the class-interval.
Median:- Median is defined as the point of the variable which divides distribution into two equal parts. The position of the median in a series is such that half of the scores are less and half of the scores are more than the median.
Mode:- Mode is the value that occurs most frequently in a set of observations. It is the point of maximum frequency around which other items of the set cluster densely. In a number of scores 4, 5, 5, 6, 7, 5, 6 mode is 5. Because 5 occurs the highest number of times.
Q.64. Compute the means, median and mode for each of the following set of scores : (a) 9, 12, 5, 14, 18, 22, 27, 25, 22
Ans:- Mean: X = 9, 12, 5, 14, 18, 22, 27, 25, 22.
∑x = 9+12+5+14+18+22+27+25+22 =154.
M = ∑x/N
= 154/9
= 17.11
∴ M = 17.11
Median :- Secores serially arranged 5,9,12,14,18,22,22,25,27
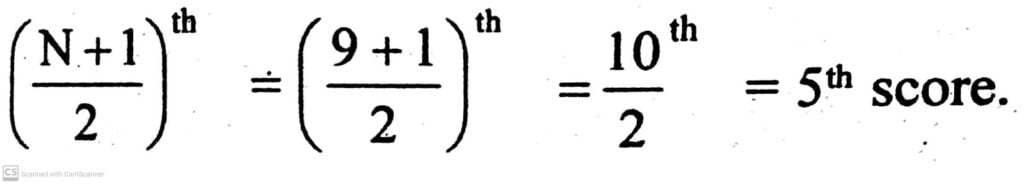
∴ Mdn = 5ᵗʰ Score = 18.
Mdn:
Scores = 9,12,5,14,18,22,27,22,25
Q.65. What do you understand by range? Give examples.
Ans:- The range in statistics for a given data set is the difference between the highest and lowest values. For example, if the given data set is { 2,5,8,10,3 }, then the range will be 10-2=8. Thus, the range could also be difined as the difference between the highest observation and lowest observation.
Q.66. What is a bar diagram? What are its types?
Ans:- A bar diagram is a type of data handling method that is popularly used in statistics. A bar graph or bar chart is a visual presentation of a group of data that is made up of vertical or horizontal rectangular bars with lengths that are equal to the measure of the data.
Types of bar diagram:-
1. Simple Bar Diagram.
2. Multiple Bar Diagram.
3. Sub-Divided Bar Diagram or Component Bar Diagram.
4. Percentage Bar Diagram.
5. Broken-Scale Bar Diagram.
6. Deviation Bar Diagram.
Q.67. What is a pie diagram? Explain the procedure of making a pie diagram.
Ans :- A pie diagram is a circular statistical graphic, which is divided into slices to illustrate numerical proportion.
The procedure of making a pie diagram are:-
Step 1. Convert the data to percentages. The first step is to convert the data to percentages.
Step 2. Calculate the angle for each pie segment. A complete circle or pie chart has 360°.
Step 3. Draw the pie chart. For this you’ll need compasses and a protractor.
Step 4. Add labels. The chart requires a little and labels.
E. Questions for 4 Mark Each :
Q.68. Distinguished between histogram and polygon.
Ans :- Difference between histogram and polygon are:-
i) Frequency polygon is an improvement over histogram because it provides a continuous curve indicating the causes of rise and fall in the data. On the other hand, frequency polygon is an approximate curve, but still it is more useful as compared to histogram.
ii) In the frequency polygon, it is assumed that the frequency distribution in a particular class-width whereas histogram may be used to represent frequency distribution with equal as well as with unequal class width.
iii) In case of frequency polygon, it is assumed that all frequencies in a particular class are connected at the mid point of that class whereas in case of histogram, it is supposed that they are evenly spread over the class interval.
Q.69. What are the different parts of a frequency distribution table?
Ans :- The different parts of a frequency distribution table are :-
(i) Class interval:- The range of such class of data is called the class interval.
(ii) Class limit:- The lower and upper limits of a class interval are known as class Limits.
(iii) Class boundaries:- The values between the upper limit of one interval and the lower limit of the next interval.
(iv) Class mark:- Midpoint of a class interval.
(v) Class frequency:- Refers to the number of observation belonging to a class interval.
(v) Class size:- The total number of observations on which class interval is formed.
Q.70. What do you understand by frequency distribution of statistical data?
Ans :- Frequency distribution table :- The table where the raw scores are systematically arranged may be known as ‘Frequency distribution table.’ In a frequency distribution table there are three columns that are class intervals, tally marks and frequencies. The range of the variable in a particular sub-range is called class- interval and the number of items in a class-interval is known as class frequency. The distribution as class frequency over the class intervals is known as the frequency distribution of the variables.
In order to prepare a frequency distribution table, the following methodical steps must be followed :
( a ) To identify the lowest and highest scores of the given number.
( b ) Choose the magnitude of class intervals. Fix up the position of intervals.
( c ) To give tally marks of the scores in the tally column of the respective class intervals.
( d ) To place in figure the tally marks of the respective intervals to show the number of frequencies clearly.
The different parts of a frequency distribution table are :
( i ) Size :- For making the classification the size of the grouping is to be determined. Using 3, 4, 5, 10 units in length may be taken and size of the class interval depending upon the range. The number of class intervals may be determined by dividing the range by the grouping interval tentatively selected.
( ii ) Tally :- A tally represents a score that lies in any one particular class interval. One tally is given for each score opposite the corresponding class interval. The fifth tally is by the oblique line over the previous four tallies.
( iii ) Frequencies :- Frequencies are represented in the 3rd column of the frequency distribution. When all the frequencies are added up, the total number of cases of N is obtained.
( iv ) The midpoint of an interval in a frequency distribution :- If all the scores are represented within a given class-interval by some single the midpoint of the interval is logical.
Q.71. Explain how a frequency table is prepared, indicating the choice of the number of classes and the width of a class interval.
Ans :- Frequency refers to the number times a particular value appears in a distribution. For example, in a distribution of values, 4, 5, 5, 6, 5, 4, the frequency of 5 is 3, that of 4 is 2 and that of 6 is 1.
Q.72. Discuss the various methods of graphical representation of educational data.
Ans :- The graphic representation of the frequency distribution can be done with the help of mainly four types of group.
They are:
( i ) Frequency polygon:- A frequency polygon is a figure representing the data on graph paper. The score values are measured along the x-axis and the frequencies along y axis. The exact limits of intervals are represented at regular distances along the baseline from the origin and the frequencies against each interval are measured upon the y axis.
( ii ) Histogram:- It is another means where the class interval is represented by a separate rectangle. The area of each rectangle of histogram is directly proportional to measures within the interval.
( iii ) Bar diagram:- When statistical data are represented by rectangular bars, the diagrams so drawn are called bar diagrams. They consist of a group of equidistant rectangles, one for each group or category of data in which the values are represented by the height of the rectangles.
( iv ) Pie-diagram:- When a circle is subdivided into various sectors for representing different data it is called a Pie-diagram. To represent the data by a pie-diagram we first draw a circle with any radius. The angle at the centre of the circle is of 360 degrees which represents the aggregate. It can be divided into a number of smaller angles whose degrees would be in proportion to the values of the components. The angle representing each item or sector may be calculated with the help of the following formula
Angle of any sector = Value of the Sector × 360°/Total value
Suppose, in a college there are 120 students in Arts and 60 students in science faculty. If the values are represented by a pie-diagram
the angle for Arts students will be
120 × 360°/180 = 240°
Similarly the angle for the science students will be
60x 360°/180 = 120°
Q.73. Represent the following data of students enrollment in a primary school by bar diagram
| Class | Boys | Girls |
| I | 24 | 16 |
| II | 20 | 12 |
| III | 20 | 14 |
| IV | 16 | 12 |
Ans :-
| Class | Boys | Girls |
| IIIIIIIV | 24202016 | 16121412 |
Q.74. What is Quartile Deviation? If 1st quartile is 34.56 and Quartile Deviation is 8.97, find the 3rd Quartile.
Ans:- Quartile deviation is the one half distance between the first and third quartile or 25ᵗʰ and 75the percentile in the frequency distribution. First quartile in a distribution means the point below which 25% of the scores lies i.e. one fourth of the total number of scores which is represented by the symbol Q₁. The third quartile in a distribution means the point below which 75% of the scores lies i.e. three fourth of the total number of scores which is represented by Q₃ .
Quartile deviation is also called semi interquartile or interquartile range. Quartile deviation or Q is computed by the formula.
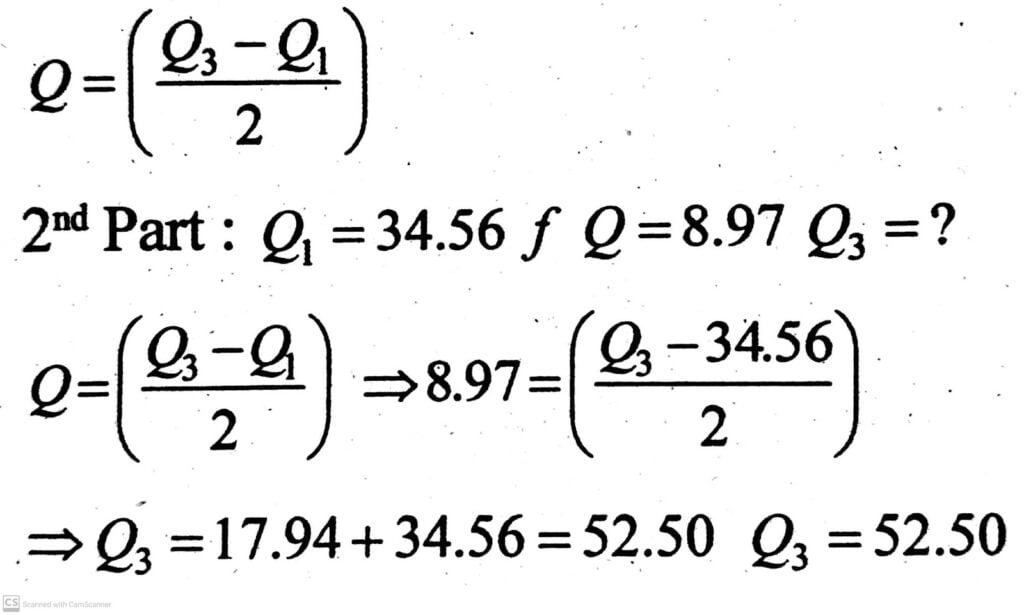
E. Questions for 5 Mark Each :
Q.75 Define statistics? Explain the utility of the science in the field of education and Psychology.
Ans :- Statistics is a branch that deals with every aspect of the data. Statistical knowledge helps to choose the proper method of collecting the data and employ those samples in the correct analysis process in order to effectively produce the results. In short, statistics is a crucial process which helps to make the decision based on the data.
Utility of statistics in education and psychology may be described below:
( i ) To measure intelligence, memory etc.:- Statistics help to measure and record the intelligence, memory, thinking, taste, etc. of students and such data when analysed generally render useful conclusions.
( ii ) To construct intelligence tests:- It also helps in the construction of intelligence tests which are based on the analysis of the past records of the intelligence of the students. The importance of intelligence tests in the field of education and psychology can hardly be exaggerated.
( iii ) Determination of the norm:- For effective measurement of attainment and other mental abilities of the students determination of the norm as standard of comparison is essential. Without a standard norm comparative study of the mental abilities in terms of the individual scores is not possible. Statistics can only help us to lay down a reliable norm for such study.
( iv ) To maintain records:- Records are generally maintained in all the educational institutions in respect of income and expenditure, number of students, examination results, etc. Statistical methods are put into use in keeping of such records.
Q.76. What is a frequency distribution? Point out its importance in statistical investigation.
Ans :- A frequency distribution is a representation, either in a graphical or tabular format, that displays the number of observations within a given interval. The frequency is how often a value occurs in an interval while the distribution is the pattern of frequency of the variable.
In order to prepare a frequency distribution table, the following methodical steps must be followed:
( a ) To identify the lowest and highest scores of the given number.
( b ) Choose the magnitude of class intervals. Fix up the position of intervals.
( c ) To give tally marks of the scores in the tally column of the respective class intervals.
( d ) To place in figure the tally marks of the respective intervals to show the number of frequencies clearly.
Importance :- ( i ) It may reduce the size of the scores to make them easily comprehensible.
( ii ) The table can show us whether the scores are more inclined towards the upper or lower limit of the distribution whether they are normally distributed.
( iii ) The toble can show in which class interval there is the highest or the lowest number of frequency to be found.
( iv ) Moreover the total is helpful for making a graphic representation of the data by drawing the polygon or histogram.
Q.77. The following scores were obtained by a group of students on an achievement tests in mathematics. (5 marks for each question)
60 49 55 48 58 65 63 66 58 58 82 84
49 66 77 54 62 38 52 52 64 82 61 78
72 58 83 67 55 62 77 55 51 59 53 58
41 54 68 72 62 56 74 51 71 60 51 69
70 68 67 48 57 44 55 48 82 77 70 42
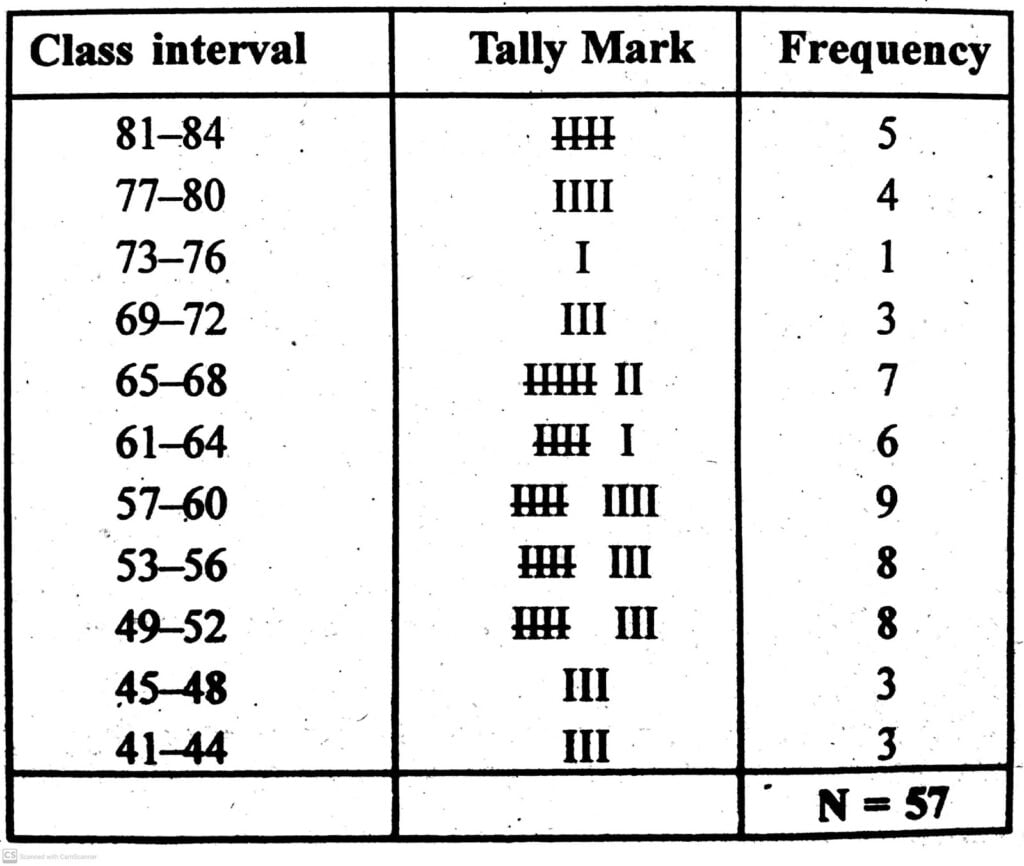
( a ) Construct a frequency distribution using class interval 5 and 41– 44 as the score limits of the lowest interval.
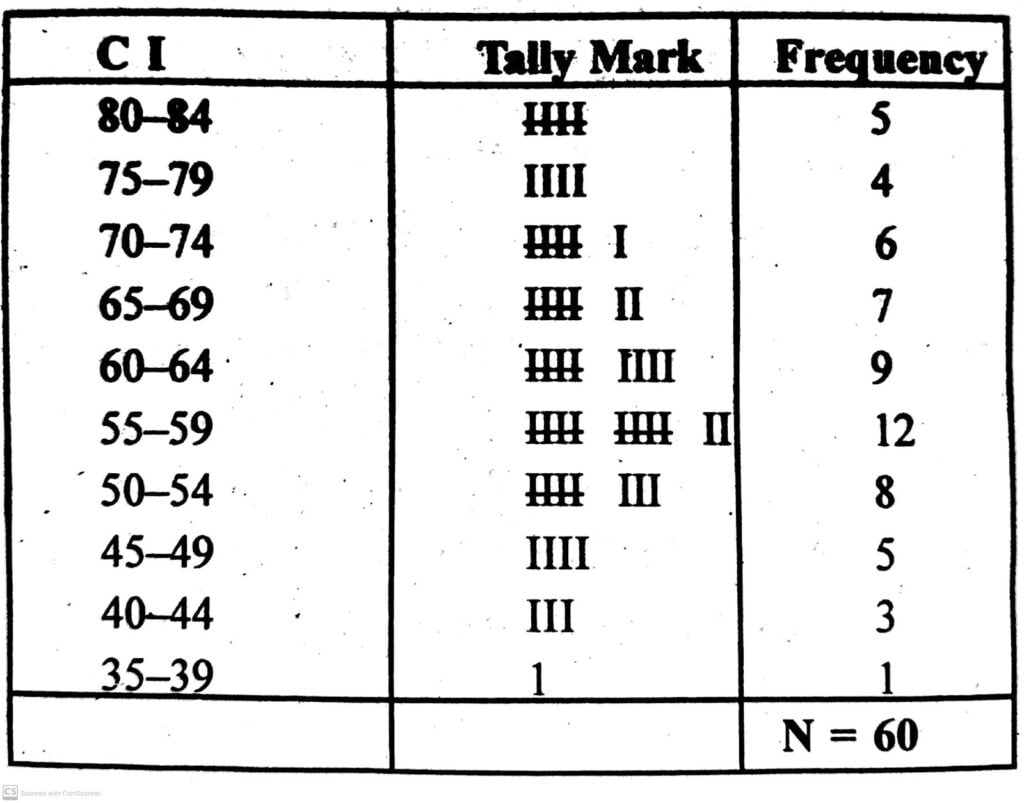
( b ) Set up a frequency distribution by using a class interval of 5 units.
( c ) Construct a frequency, a histogram of the data given in Question No. 61
( d ) Construct a frequency polygon of the data given in Question No.61
Ans :- ( a )
( b )
( c )
| C I | Tally | Frequency |
| 80-84 | 5 | 82 |
| 75-79 | 4 | 77 |
| 70-74 | 6 | 72 |
| 65-69 | 7 | 67 |
| 60-64 | 9 | 62 |
| 55-59 | 12 | 57 |
| 50-54 | 8 | 52 |
| 45-49 | 5 | 47 |
| 40-44 | 3 | 42 |
| 35-39 | 1 | 37 |
Q.78. Draw a histogram and frequency polygon on the same axis of following data and discuss the relative advantages of these two methods of representation of data. (8 marks)
| Scores | F | Mid Points |
| 50-54 | 1 | ( x ) |
| 45-49 | 7 | |
| 40-44 | 3 | |
| 35-39 | 4 | |
| 30-34 | 6 | |
| 25-29 | 7 | |
| 20-24 | 12 | |
| 15-19 | 6 | |
| 10-14 | 8 | |
| 5-9 | 2 |
What are the different measures of central tendency? What ara their advantages?
Ans :-
| Scores | F | Mid Points( x ) |
| 50-54 | 1 | 52 |
| 45-49 | 7 | 47 |
| 40-44 | 3 | 42 |
| 35-39 | 4 | 37 |
| 30-34 | 6 | 32 |
| 25-29 | 7 | 27 |
| 20-24 | 12 | 22 |
| 15-19 | 6 | 17 |
| 10-14 | 8 | 12 |
| 5-9 | 2 | 7 |
POLYGON, HISTOGRAM.
Q.79. The Mean of a group of scores is 35. When the mean would be if the score were first altered by each of the following methods:
( a ) 15 points are added to each score.
( b ) 10 points are subtracted from each score.
( c ) Each score is multiplied by 5.
( d ) Each score is divided by 4.
( e ) 10 points are added to each score.
Ans :- Properties of Mean :- ( i ) It is used when we wish to know the representative value of the scores.
( ii ) It may be used when the greatest sampling stability is wanted.
( iii ) It is usable when the distribution of the scores is normal or Symmetrical.
Properties of median :- ( i ) It can be used when we do not have sufficient time to compute the mean.
( ii ) It can be used when the scores are abnormally distributed or marked skewed.
( iii ) It is used when the midpoint of the distribution i.e. 50% is wanted.
( iv ) It may be used when there is a gap in the distribution of the frequency.
Properties of mode :- ( i ) It is used when rough estimate of central tendency may serve the purpose.
( ii ) It is helpful when we must to know the most typical case arising out of the distribution.
( iii ) It appears best to represent the group.
Q.80. Calculate the mean by long method from the following table
| Class Interval | Frequencies |
| 78-79 | 3 |
| 76-77 | 5 |
| 74-75 | 6 |
| 72-73 | 8 |
| 71-70 | 7 |
| 68-69 | 14 |
| 66-67 | 11 |
| 64-65 | 2 |
| 62-63 | 5 |
| 60-61 | 4 |
Ans :- Mean = 35
15 points are added.
∴ 30 + 15 = 50
50 + 50 + 50 + 50 + 50/5
= 250/5
= 50 .
( b ) 10 points is subtracted .
30 – 10 = 25
25 + 25 + 25 + 25 + 25/5
= 25
( c ) Each score is multiplied by 5.
35 × 5 = 175
175 + 175 + 175 + 175 + 175/5
= 175
( d ) Each score is divided by 4
35 ÷ 4 = 8.75
8.75 + 8.75 + 8.75 + 8.75 + 8.75/5
= 8.75
( e ) 10 points are added
35 + 10 = 45
45 + 45 + 45 + 45 + 45/5
= 45
Q.81. Calculate the mean marks of 30 students given in the following distribution by ‘Assumed method and also determine the Median of the distribution. (8 marks)
Class interval
33-36 29-32 25-28 21-24 17-20 13-16 9-12 5-8 1-4
F 1 1 4 10 5 3 3 2 1
Ans :-
| C.I. | Frequency | x | fx |
| 78-79 | 3 | 78.5 | 235.5 |
| 76-77 | 5 | 76.5 | 282.5 |
| 74-75 | 6 | 74.5 | 447 |
| 72-73 | 8 | 72.5 | 580 |
| 71-70 | 7 | 71.5 | 500.5 |
| 68-69 | 14 | 68.5 | 959 |
| 66-67 | 11 | 66.6 | 731.5 |
| 64-65 | 2 | 66.5 | 129 |
| 62-63 | 5 | 62.5 | 312.5 |
| 60-61 | 4 | 60.5 | 242 |
| N = 65 | ∑fx = 4519.5 |
Mean ( M ) = AM + ∑fx/N× i
= 22.5 + 12/30× 4
= 22.5 + 48/30
= 22.5 + 16
Mean = 388.5
Median = N + 1/2
= 30 + 1/2
= 15.5
Q.82. What is meant by variability of scores and what are its measures? Discuss the uses of range.
Ans :-
| C.I. | f | x | fx |
| 150-154 | 4 | 152 | 608 |
| 145-149 | 8 | 147 | 1176 |
| 140-149 | 10 | 142 | 1420 |
| 135-139 | 12 | 137 | 1644 |
| 130-134 | 2 | 132 | 264 |
| 125-129 | 10 | 127 | 1270 |
| 120-124 | 7 | 122 | 854 |
| 115-119 | 8 | 117 | 936 |
| 110-114 | 5 | 112 | 560 |
| N = 66 | ∑fx = 8732 |
Mean = ∑fx/N
= 8732/66
= 132.30
Median = N + 1/2
= 66 + 1/2
= 67/2
= 33.5
Q.83. What is quartile deviation? Discuss the uses of quartile deviation as measures variability.
Ans :- Quartile deviation is the difference between the first quartile and the third quartile in the frequency distribution table. This is also known as the interquartile range. The quartile deviation helps to examine the spread of a distribution about a measure of its central tendency, usually the mean or the average. Hence, it is in use to give you an idea about the range within which the central 50% of your sample data lies.
Q.85. Explain the properties of range and quartile deviation as measures of variability.
Ans :- Properties of Range:-
i) The value of the range is always non- negative. Since finding the range involves substracting a smaller number from a large number we see that range is always either positive or equal to zero.
ii) If the range is non-zero we can conclude that the given data values are not all equal to each other.
iii) For a continuous frequency distribution, we can find the range by subtracting the lower limit of the smallest class from the upper limit of the highest class.
iv) When finding the range for a frequency distribution we do not consider frequencies of the classes. This is because the range is calculated only on the basis of the two extreme observations.
Q.86. Discuss their relative importance of range and quartile deviation as measures variability.
Ans :- Properties of quartile deviation :- Quartile deviation defined as one-half the scale distance between the 75th and 25th percentiles in the frequency distribution. The 25th percentile of distribution of scores in the first quartile or Q₁ and the 75th percentile is the third quarter or Q₃ The 50th percentile of may be distribution is popularly known as or the median. The difference between the third and the first quartile value is the inter-quartile difference. This difference when divided by 2, we get the quartile deviation.
The formula representing the concept is :
Q = Q₃ – Q₁/2
( i ) When the median is the measure of centre frequency.
( ii ) When there are scattered or extreme scores which would influence the standard deviation (SD) disproportionality.
( iii ) When the concentration around the mean the middle 50% of cases is of primary interest.
( iv ) When the distribution is incomplete at either end.
Q.86. Calculate the Quartile deviation for the following distribution of scores.
Class interval 50-54 45-49 40-44 35-39 30-34 25-29 20-24 15-19 10-14 5-9
frequencies 1 7 3 4 6 7 12 6 8 2
Ans :-
| Class Interval | frequencies | Deviation frequencies |
| 50-54 | 1 | 56 |
| 44-49 | 7 | 55 |
| 40-41 | 3 | 48 |
| 35-39 | 4 | 45 Q3 |
| 30-34 | 6 | 41 |
| 25-29 | 7 | 35 |
| 20-24 | 12 | 28 |
| 15-19 | 6 | 16 Q1 |
| 10-14 | 8 | 10 |
| 5-9 | 2 | 2 |
| N = 56 |
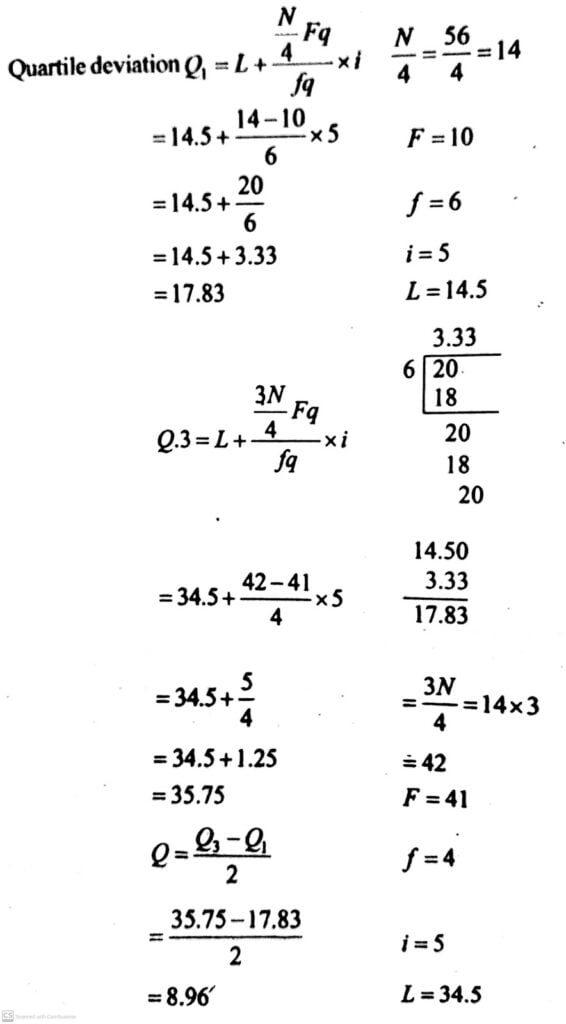
Q.88. Calculate the mean by long method from the following table:
Class 78-79 76-77 74-75 73-72 71-70 68-69 66-67 64-65 62-63 60-61
Interval Frequency 3 5 6 8 7 14 11 2 5 4
Ans :- The formula of mean by long method is –
M = ∑fx/N
Computing Mean by long method:
| Class Interval | Frequency | X Middle Point | f.x. |
| 78-79 | 3 | 78.5 | 235.5 |
| 76-77 | 5 | 76.5 | 382.5 |
| 74-75 | 6 | 74.5 | 447.0 |
| 73-72 | 8 | 73.5 | 588.0 |
| 71-70 | 7 | 71.5 | 500.5 |
| 68-69 | 14 | 68.5 | 959.0 |
| 66-67 | 11 | 66.5 | 731.5 |
| 64-65 | 2 | 64.5 | 129.0 |
| 62-63 | 5 | 62.5 | 312.5 |
| 60-61 | 4 | 60.5 | 242.0 |
| N = 65 | ∑fx = 4527.5 |
∴ M = ∑fxN[ ∑fx = 4527.5, N = 65 ]
= 4527.5/65
= 69.65
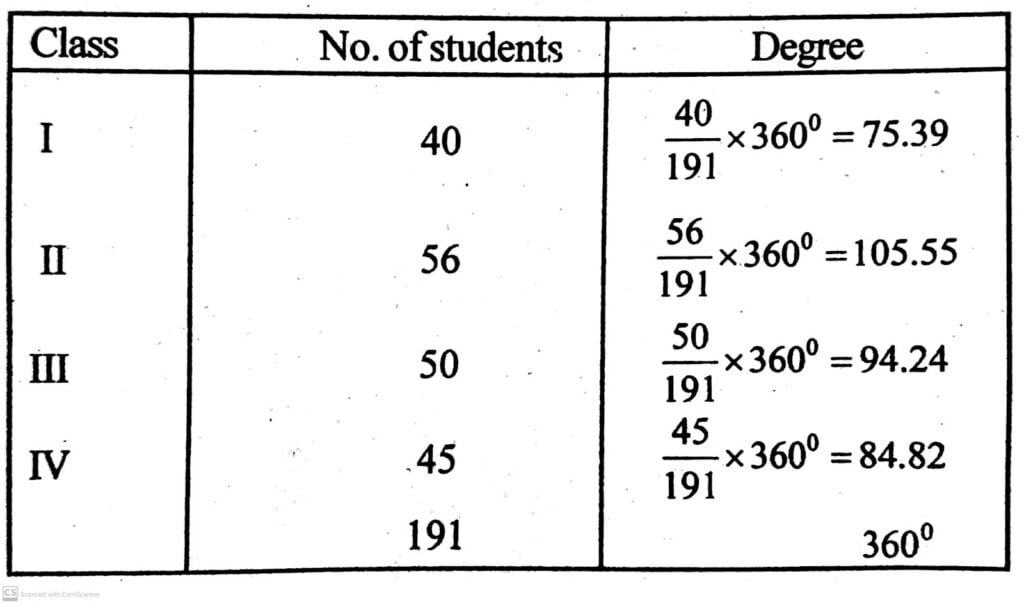
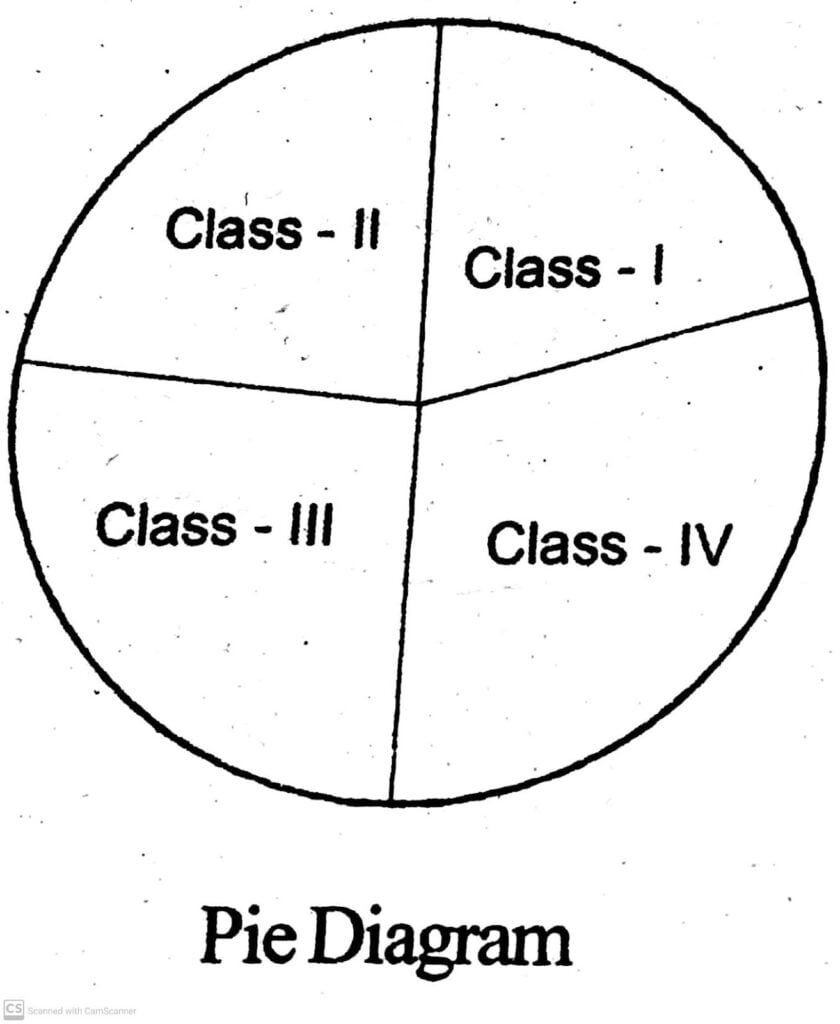
Q.89. Represent the following data of students enrollment in a primary school by pie diagram
| Class | No. of Students |
| I | 40 |
| II | 46 |
| III | 50 |
| IV | 45 |
Ans :- Calculation of pie diagram:
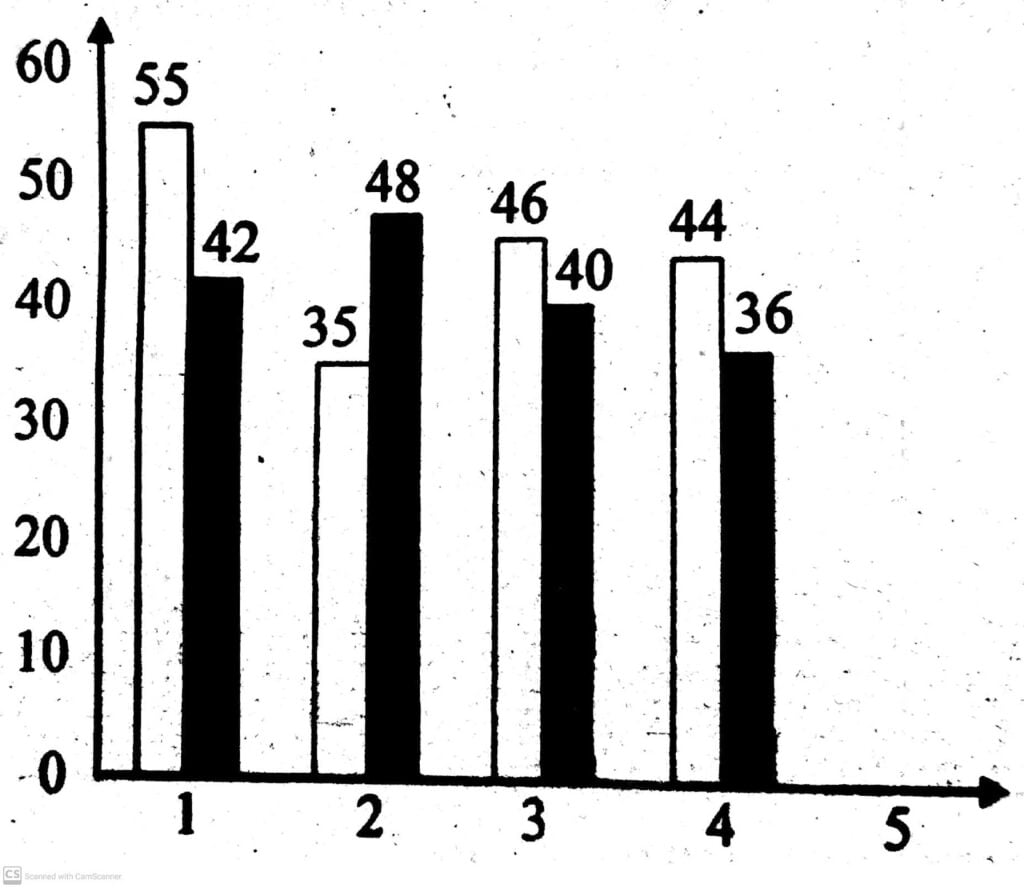
Q.90. Represent the following data of students, enrollment in a primary school by bar diagram. (Use graph paper).
| Class | Boys | Girls |
| Class – I | 55 | 42 |
| Class – II | 35 | 48 |
| Class – III | 46 | 40 |
| Class – IV | 44 | 36 |

Hi! my Name is Parimal Roy. I have completed my Bachelor’s degree in Philosophy (B.A.) from Silapathar General College. Currently, I am working as an HR Manager at Dev Library. It is a website that provides study materials for students from Class 3 to 12, including SCERT and NCERT notes. It also offers resources for BA, B.Com, B.Sc, and Computer Science, along with postgraduate notes. Besides study materials, the website has novels, eBooks, health and finance articles, biographies, quotes, and more.




